In the below fig. POQ is a line. Ray OR is perpendicular to line PQ. OS is another ray lying between rays OP and OR. Prove that ∠ROS = 1/2(∠QOS − ∠POS).
Question:
In the below fig. POQ is a line. Ray OR is perpendicular to line PQ. OS is another ray lying between rays OP and OR. Prove that ∠ROS = 1/2(∠QOS − ∠POS).
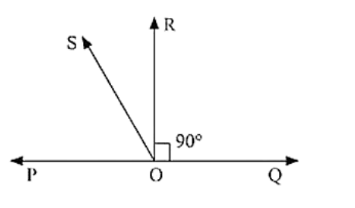
Solution:
Given that
OR perpendicular
∴ ∠POR = 90°
∠POS + ∠SOR = 90 [∴ ∠POR = ∠POS + ∠SOR]
∠ROS = 90° − ∠POS ... (1)
∠QOR = 90 (∵ OR ⊥ PQ)
∠QOS − ∠ROS = 90°
∠ROS = ∠QOS − 90°
By adding (1) and (2) equations, we get
∴ ∠ROS = ∠QOS − ∠POS
∠ROS = 1/2(∠QOS − ∠POS)
