In the adjoining figure, ABCD is a parallelogram in which ∠A = 60°.

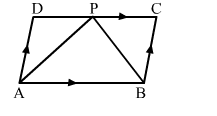
ABCD is a parallelogram.
∴ ​∠A = ​∠C and ∠B =​ ∠D (Opposite angles)
And ​∠A + ​∠B = 180o                (Adjacent angles are supplementary)
∴ ​∠B = 180o − ∠A 
⇒ 180o − 60o = 120o             ( ∵∠A = 60o)
∴ ​∠A = ​∠C = 60o and ∠B =​ ∠D​ = 120o

(i) $\ln \triangle A P B, \angle P A B=\frac{60^{\circ}}{2}=30^{\circ}$ and $\angle P B A=\frac{120^{\circ}}{2}=60^{\circ}$

$\therefore \angle A P B=180^{\circ}-\left(30^{\circ}+60^{\circ}\right)=90^{\circ}$

(ii) In ∆ ADP, ∠​PAD = 30o and ∠ADP = 120o
    ∴ ∠APB = 180o − (30o + 120o) = 30o
   Thus, ∠​PAD = ​∠APB = ​30o
   Hence, ∆ADP is an isosceles triangle and AD = DP.

   In ∆ PBC, ∠​ PBC = 60o,  ∠​ BPC = 180o − (90o +30o) = 60o and ∠​ BCP  = 60o (Opposite angle of ∠A)
   ∴ ∠ PBC = ∠​ BPC = ∠​ BCP
   Hence, ∆PBC is an equilateral triangle and, therefore, PB = PC = BC.​

(iii) DC = DP + PC
  From (ii), we have:
   DC = AD + BC               [AD = BC, opposite sides of a parallelogram]
 ⇒ DC = AD + AD