In the adjoining figure, ABCD is a || gm in which E and F are the midpoints of AB and CD respectively.
Question:
In the adjoining figure, ABCD is a || gm in which E and F are the midpoints of AB and CD respectively. If GH is a line segment that cuts AD, EF and BC at G, P and H respectively, prove that GP = PH.
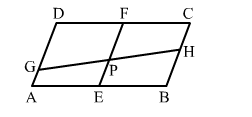
Solution:
In parallelogram ABCD, we have:
AD || BC and AB || DC
AD = BC and AB = DC
AB = AE + BE and DC = DF + FC
AB = AE + BE and DC = DF + FC
∴ AE = BE = DF = FC
Now, DF = AE and DF || AE.
i.e., AEFD is a parallelogram.
∴ AD || EF
Similarly, BEFC is also a parallelogram.
∴ EF || BC
∴ AD || EF || BC
Thus, AD, EF and BC are three parallel lines cut by the transversal line DC at D, F and C, respectively such that DF = FC.
These lines AD, EF and BC are also cut by the transversal AB at A, E and B, respectively such that AE = BE.
Similarly, they are also cut by GH.
∴ GP = PH (By intercept theorem)
Comments
Bla bla 🖤 Darling 😘💟
Feb. 23, 2025, 6:35 a.m.
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️✨✨✨✨✨✨✨✨✨✨✨✨✨✨💗💗💗💗💗💗💗💗💗💗💗💗💗😙😙❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️❤️😙❤️❤️😙😙❤️❤️❤️❤️❤️❤️💯💯💯💯💯💯💯💯💯💯💯💯💯💯💯💯💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💞💝💝💝💝💝💝💝💝💝💝💝💝❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️❣️💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💓💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥💥🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟🌟⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐🎉🎉🎉🎉💝🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉🎉💫💫💫💫💫💫💫💫💫💫💫💫⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐⭐💫💫💫💫💫💫💫💫💫💫💫💫💫💫✌️✌️✌️✌️✌️✌️✌️✌️✌️🤞✌️✌️🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤞✌️🤙✌️🤞✌️🤞✌️🤞✌️🤞✌️🤟🖖🤘🤟👌✌️👉✌️👉👊🖐️✊✋✌️👉🤟👌✌️🖖🤞👆🤞👆🤙🤘🤙🤘👌✊🖐️✊🖐️✊🤙🤛🤙🤘🤞🤟🤞👆🤞👆✌️👇🤞🤞🤟👋🤜🤛🤙🤙✊✌️🤚🖐️🤛👉🤛👍👂👂💪👐💪👐🖐️🤛🖐️👌🤛🤛🤙🤛🤞🤞🤜🤞🤞👆🤞👆👇👋👆👋👆👋👆👍👍👍👍🤜👍🤛🤞🤛🤙✊🤚✊🖐️🤛✋🤘👉🤞🤟👆👊🤟👋🤞🤜🤞🤛🤚🤛🤙🤛🤛👌🤛✋🤜👌🤘👋👆👊👊✊🤲👇☝️👇🤘🖖✊🤟✌️🖖🖖👌🤟👉👉🤘👉🤛🖐️✊🖐️💪🖐️👏💪👋👍🤛👊🤛👊🤛👊🤘👀👀👀👀👀👀👁️👁️👁️👥👄👥👄👥👄👥👄👥👄👥👀👥💋💋💋💋💋💋💋💋💗💟💛🕳️🕳️🕳️🌜🕳️😽💫😽💩🙊😎👻🤡💀