Question:
In following figure if $A D$ is the bisector of $\angle B A C$, then prove that $A B>B D$.
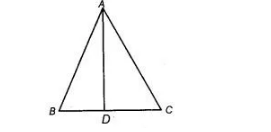
Solution:
Given ABC is a triangle such that AD is the bisector of ∠BAC. To prove AB > BD.
Proof Since, AD is the bisector of ∠BAC.
But ∠BAD = CAD …(i)
∴ ∠ADB > ∠CAD
[exterior angle of a triangle is greater than each of the opposite interior angle]
∴ ∠ADB > ∠BAD [from Eq. (i)]
AB > BD [side opposite to greater angle is longer]
Hence proved.
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.