Question:
In a parallel plate capacitor set up, the plate area of capacitor is $2 \mathrm{~m}^{2}$ and the plates are separated by $1 \mathrm{~m}$. If the space between the plates are filled with a dielectric material of thickness $0.5 \mathrm{~m}$ and area $2 \mathrm{~m}^{2}$ (see fig.) the capacitance of the set-up will be_________$\varepsilon_{0}$
(Dielectric constant of the material $=3.2$ )
(Round off to the Nearest Integer)
Solution:
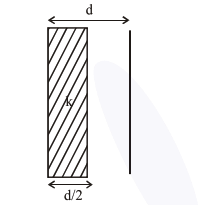
Ans. (3)
$\mathrm{C}=\frac{\varepsilon_{0} \mathrm{~A}}{\frac{\mathrm{d}}{2 \mathrm{~K}}+\frac{\mathrm{d}}{2}}=\frac{2 \varepsilon_{0} \mathrm{~A}}{\frac{\mathrm{d}}{\mathrm{K}}+\mathrm{d}}$
$=\frac{2 \times 2 \varepsilon_{0}}{\frac{1}{3.2}+1}=\frac{4 \times 3.2}{4.2} \varepsilon_{0}$
$=3.04 \varepsilon_{0}$
