Question:
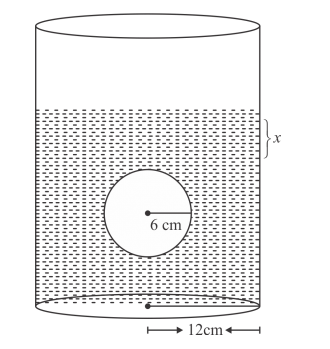
In a cylindrical vessel of diameter 24 cm, filled up with sufficient quantity of water, a solid spherical ball of radius 6 cm is completely immersed. Find the increase in height of water level.
Solution:
Radius of spherical ball r = 6 cm, radius of cylindrical vessel r1 = 12 cm
Since, the ball completely immersed into the vessel, the water level is increased.
Let the height of increased level.
Therefore,
The volume of increase water level = volume of ball
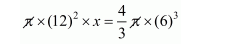 '
'
$144 x=\frac{4}{3} \times 216$
$144 x=4 \times 72$
$x=\frac{4 \times 72}{144}$
$x=2 \mathrm{~cm}$
Hence, the level of water increased by 2 cm.
