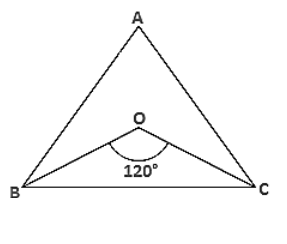
Question:
In a ΔABC, ∠ABC = ∠ACB and the bisectors of ∠ABC and ∠ACB intersect at O such that ∠BOC = 120°. Show that ∠A = ∠B = ∠C = 60°.
Solution:
Given,
In ΔABC,
∠ABC = ∠ACB
Dividing both sides by '2'
$\frac{1}{2} \angle \mathrm{ABC}=\frac{1}{2} \angle \mathrm{ACB}$
⇒ ∠OBC = ∠OCB [ ∴ OB, OC bisects ∠B and ∠C]
Now,
$\angle \mathrm{BOC}=90^{\circ}+\frac{1}{2} \angle \mathrm{A}$
$\Rightarrow 120^{\circ}-90^{\circ}=\frac{1}{2} \angle \mathrm{A}$
⇒ 30°∗ (2) = ∠A
⇒ ∠A = 60°
Now in ΔABC
∠A + ∠ABC + ∠ACB = 180° (Sum of all angles of a triangle)
⇒ 60° + 2∠ABC = 180° [∴ ∠ABC = ∠ACB]
⇒ 2∠ABC = 180° − 60°
⇒ ∠ABC = 120°/2 = 60°
⇒ ∠ABC = ∠ACB
∴ ∠ACB = 60°
Hence Proved.
