If the length of the shadow of a tower is

(b) 30°

Let $A B$ be the pole and $B C$ be its shadow.

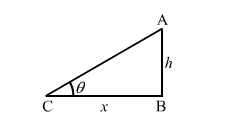
Let $A B=h$ and $B C=x$ such that $x=\sqrt{3} h$ (given) and $\theta$ be the angle of elevation.

From $\triangle A B C$, we have:

$\frac{A B}{B C}=\tan \theta$

$\Rightarrow \frac{h}{x}=\frac{h}{\sqrt{3} h}=\tan \theta$

$\Rightarrow \tan \theta=\frac{1}{\sqrt{3}}$

$\Rightarrow \theta=30^{\circ}$

Hence, the angle of elevation is $30^{\circ}$.