If the elevation of the sun changes form 30° to 60°, then the difference between the lengths of shadows of a pole 15 m high, is

(c) $10 \sqrt{3} \mathrm{~m}$

Let $A B$ be the pole and $A C$ and $A D$ be its shadows.

We have:

$\angle A C B=30^{\circ}, \angle A D B=60^{\circ}$ and $A B=15 \mathrm{~m}$

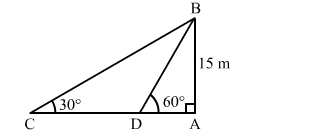
In $\triangle A C B$, we have:

$\frac{A C}{A B}=\cot 30^{\circ}=\sqrt{3}$

$\Rightarrow \frac{A C}{15}=\sqrt{3} \Rightarrow A C=15 \sqrt{3} \mathrm{~m}$

Now, in $\triangle A D B$, we have:

$\frac{A D}{A B}=\cot 60^{\circ}=\frac{1}{\sqrt{3}}$

$\Rightarrow \frac{A D}{15}=\frac{1}{\sqrt{3}} \Rightarrow A D=\frac{15}{\sqrt{3}}=\frac{15 \times \sqrt{3}}{\sqrt{3} \times \sqrt{3}}=\frac{15 \sqrt{3}}{3}=5 \sqrt{3} \mathrm{~m}$

$\therefore$ Difference between the lengths of the shadows $=A C-A D=15 \sqrt{3}-5 \sqrt{3}=10 \sqrt{3} \mathrm{~m}$