If the angles of elevation of the top of a tower from two points at a distance of

Let  be the height of tower is meters.

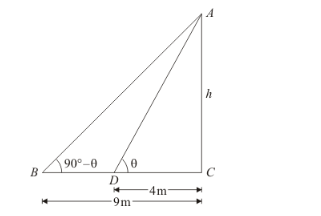
Given that angle of elevation are $\angle B=90^{\circ}-\theta$ and $\angle D=\theta$ and also $C D=4 \mathrm{~m}$ and $B C=9 \mathrm{~m}$.

Here we have to find height of tower.

So we use trigonometric ratios.

In a triangle,

$\tan \theta=\frac{h}{4}$

Again in a triangle ,

$\Rightarrow \tan \left(90^{\prime}-\theta\right)=\frac{A C}{B C}$

$\Rightarrow \cot \theta=\frac{h}{9}$

$\Rightarrow \frac{1}{\tan \theta}=\frac{h}{9}$

Put $\tan \theta=\frac{h}{4}$

$\Rightarrow \frac{4}{h}=\frac{h}{9}$

$\Rightarrow h^{2}=36$

$\Rightarrow h=6$

Hence height of tower is meters.