Question:
If $\vec{a}=\vec{b}+\vec{c}$, then is it true that $|\vec{a}|=|\vec{b}|+|\vec{c}| ?$ Justify your answer.
Solution:
In $\triangle \mathrm{ABC}$, let $\overrightarrow{\mathrm{CB}}=\vec{a}, \overrightarrow{\mathrm{CA}}=\vec{b}$, and $\overrightarrow{\mathrm{AB}}=\vec{c}$ (as shown in the following figure).
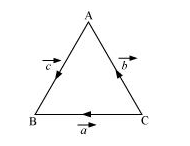
Now, by the triangle law of vector addition, we have $\vec{a}=\vec{b}+\vec{c}$.
It is clearly known that $|\vec{a}|,|\vec{b}|$, and $|\vec{c}|$ represent the sides of $\triangle \mathrm{ABC}$.
Also, it is known that the sum of the lengths of any two sides of a triangle is greater than the third side.
$\therefore|\vec{a}|<|\vec{b}|+|\vec{c}|$
Hence, it is not true that $|\vec{a}|=|\vec{b}|+|\vec{c}|$.
