If PT is tangent drawn from a point P to a circle touching it at T and

Let us first put the given data in the form of a diagram.

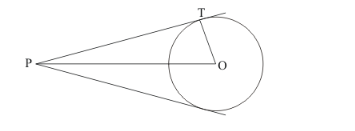
We know that the radius will always be perpendicular to the tangent at the point of contact. Therefore,

$O T \perp P T$

$\angle O T P=90^{\circ}$

Consider $\triangle O T P$. We know that sum of all angles of a triangle will be $180^{\circ}$. Therefore,

$\angle O T P+\angle P O T+\angle O P T=180^{\circ}$

Since $\angle O T P=90^{\circ}$, we have,

$\angle O T P+\angle P O T+\angle O P T=180^{\circ}$

$90^{\circ}+\angle P O T+\angle O P T=180^{\circ}$

$\angle P O T+\angle O P T=90^{\circ}$

Choice (c) is the right answer.