If n is the number of irrational terms in the expansion o

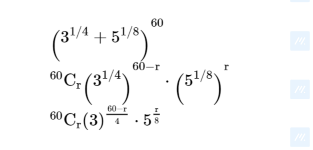
For rational terms.

$\frac{r}{8}=k ; 0 \leq r \leq 60$

$0 \leq 8 k \leq 60$

$0 \leq k \leq \frac{60}{8}$

$0 \leq k \leq 7.5$

$\mathrm{k}=0,1,2,3,4,5,6,7$

$\frac{60-8 \mathrm{k}}{4}$ is always divisible by 4 for all value of $\mathrm{k}$ Total rational terms $=8$ Total

terms $=61$

irrational terms $=53$

$n-1=53-1=52$

52 is divisible by 26 .