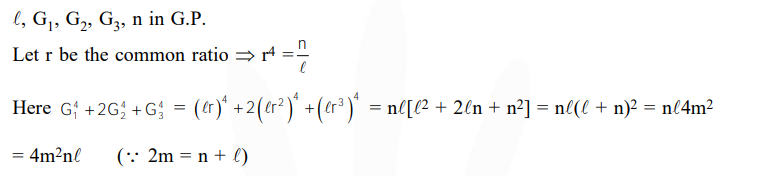Question:
If $\mathrm{m}$ is the A.M. of two distinct real numbers $l$ and $n(1, n>1)$ and $G_{1}, G_{2}$ and $G_{3}$ are three geometric means between 1 and $n$, then $G_{1}^{4}+2 G_{2}^{4}+G_{3}^{4}$ equals -
Correct Option: , 4
Solution: