If angles A, B, C and D of the quadrilateral

(c) Given, ratio of angles of quadrilateral ABCD is 3 : 7 : 6 : 4.

Let angles of quadrilateral ABCD be 3x, 7x, 6x and 4x, respectively. We know that, sum of all angles of a quadrilateral is 360°.

3x + 7x + 6x + 4x = 360°

=> 20x = 360°

=> x=360°/20° = 18°

$\therefore \quad$ Angles of the quadrilateral are

$\angle A=3 \times 18=54^{\circ}$

$\angle B=7 \times 18=126^{\circ}$

$\angle C=6 \times 18=108^{\circ}$

and          $\angle D=4 \times 18=72^{\circ}$

From finure. $\quad \angle B C E=180^{\circ}-\angle B C D \quad$ [linear pair axiom]

$\Rightarrow \quad \angle B C E=180^{\circ}-108^{\circ}=72^{\circ}$

Here, $\angle B C E=\angle A D C=72^{\circ}$

Since the corresponding angles are equal.

$\therefore$ $B C \| A D$

Now, sum of cointerior angles,

$\angle A+\angle B=126^{\circ}+54^{\circ}=180^{\circ}$

and $\quad \angle C+\angle D=108^{\circ}+72^{\circ}=180^{\circ}$

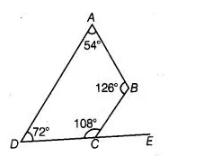
Hence, $A B C D$ is a trapezium.