From the top of a 7-metre-high building, the angle of elevation of the top of a cable tower is 60° and the angle of depression of its foot is 45°.

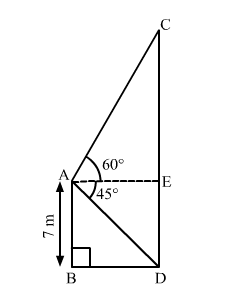
Let AB be the 7-m high building and CD be the cable tower.

We have,

$\mathrm{AB}=7 \mathrm{~m}, \angle \mathrm{CAE}=60^{\circ}, \angle \mathrm{DAE}=\angle \mathrm{ADB}=45^{\circ}$

Also, $\mathrm{DE}=\mathrm{AB}=7 \mathrm{~m}$

In $\triangle \mathrm{ABD}$,

$\tan 45^{\circ}=\frac{\mathrm{AB}}{\mathrm{BD}}$

$\Rightarrow 1=\frac{7}{\mathrm{BD}}$

$\Rightarrow \mathrm{BD}=7 \mathrm{~m}$

So, $A E=B D=7 \mathrm{~m}$

Also, in $\triangle \mathrm{ACE}$,

$\tan 60^{\circ}=\frac{\mathrm{CE}}{\mathrm{AE}}$

$\Rightarrow \sqrt{3}=\frac{\mathrm{CE}}{7}$

$\Rightarrow \mathrm{CE}=7 \sqrt{3} \mathrm{~m}$

Now, $C D=C E+D E$

$=7 \sqrt{3}+7$

$=7(\sqrt{3}+1) \mathrm{m}$

$=7(1.732+1)$

$=7(2.732)$

$=19.124$

$\approx 19.12 \mathrm{~m}$

So, the height of the tower is 19.12 m.