Following are the marks obtained,

Given the marks obtained, out of 100, by two students Ravi and Hashina in 10

tests

Now we have to find who is more intelligent and who is more consistent Case 1: For Ravi

The marks of Ravi being taken separately and finding other values can be tabulated as shown below,

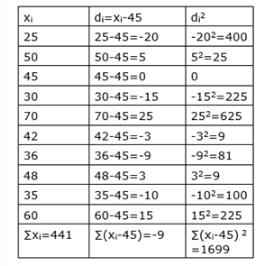
Here we have assumed 45 as mean.

Total there are marks of 10 subjects.

Now we know standard deviation is given by,

$\sigma=\sqrt{\frac{\sum\left(\mathrm{x}_{\mathrm{i}}-\mathrm{a}\right)^{2}}{\mathrm{n}}-\left(\frac{\sum\left(\mathrm{x}_{\mathrm{i}}-\mathrm{a}\right)}{\mathrm{n}}\right)^{2}}$

Substituting the corresponding values, we get

$\sigma=\sqrt{\frac{1699}{10}-\left(\frac{-9}{10}\right)^{2}}$

$\sigma=\sqrt{169.9-0.81}$

$\sigma=\sqrt{169.09}$

$\sigma=13$

And mean is

$\overline{\mathrm{x}}=\mathrm{A}+\frac{\sum \mathrm{d}_{\mathrm{i}}}{\mathrm{N}}=45-\frac{9}{10}=44.1$

Hence the mean and standard deviation of the Ravi is $44.1$ and 13 respectively.

Case 1: For Hashina

The marks of Hashina being taken separately and finding other values can be tabulated as shown below,

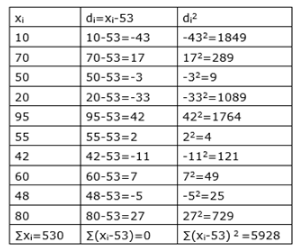
Here as $\frac{530}{10}=53$, so 53 is mean.

Total there are marks of 10 subjects.

Now we know standard deviation is given by,

$\sigma=\sqrt{\frac{\sum\left(\mathrm{x}_{\mathrm{i}}-\mathrm{a}\right)^{2}}{\mathrm{n}}-\left(\frac{\sum\left(\mathrm{x}_{\mathrm{i}}-\mathrm{a}\right)}{\mathrm{n}}\right)^{2}}$

Substituting the corresponding values, we get

$\sigma=\sqrt{\frac{5928}{10}-\left(\frac{0}{10}\right)^{2}}$

$\sigma=\sqrt{592.8}$

$\sigma=24.35$

Hence the mean and standard deviation of the Hasina is 53 and $24.35$ respectively.

Now we will analyze them,

For Ravi,

C. $V=\frac{\sigma}{\bar{x}} \times 100=\frac{13}{44.1} \times 100=29.48$

For Hasina,

C. $V=\frac{\sigma}{\bar{x}} \times 100=\frac{24.35}{53} \times 100=45.94$

Now as CV (of Ravi) < CV of Hashina Hence Ravi is more consistent. Mean of Hashina $>$ Mean of Ravi, Hence Hashina is more intelligent.