Question:
Find the values of $a$ and $b$ such that the following functions $f$, defined as $\left\{\begin{array}{l}\operatorname{asin} \frac{\pi}{2}(x+1), x \leq 0 \\ \frac{\tan x-\sin x}{x^{3}}, x>0\end{array}\right.$ is continuous
at $x=0$
Solution:
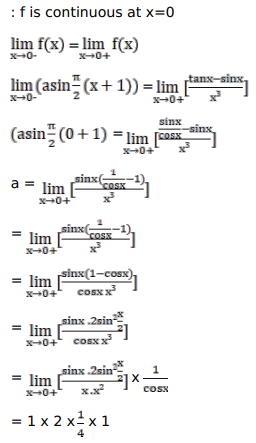
$=\frac{1}{2}$
