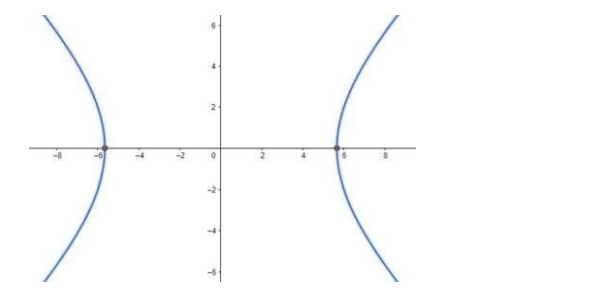
Find the equation of the hyperbola with eccentricity

Solution:

Given: Eccentricity is $\sqrt{2}$, and the distance between foci is 16

Need to find: The equation of the hyperbola.

Let, the equation of the hyperbola be:

$\frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}=1$

Distance between the foci is 16, i.e., $2 \mathrm{ae}=16$

And also given, the eccentricity, e =

$\sqrt{2}$

Therefore,

$2 \mathrm{a} \sqrt{2}=16$

$a=\frac{16}{2 \sqrt{2}}=\frac{8}{\sqrt{2}}=4 \sqrt{2} \cdots(1)$

We know that, $e=\sqrt{1+\frac{b^{2}}{a^{2}}}$

Therefore,

$\Rightarrow \sqrt{1+\frac{b^{2}}{a^{2}}}=\sqrt{2}$

$\Rightarrow 1+\frac{\mathrm{b}^{2}}{\mathrm{a}^{2}}=2$ [Squaring both sides]

$\Rightarrow \frac{\mathrm{b}^{2}}{\mathrm{a}^{2}}=1$

$\Rightarrow \mathrm{b}^{2}=\mathrm{a}^{2}=32[$ From (1) $]$

So, the equation of the hyperbola is,

$\frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}=1 \Rightarrow \frac{x^{2}}{32}-\frac{y^{2}}{32}=1 \Rightarrow x^{2}-y^{2}=32$ [Answer]