Find the equation of the hyperbola whose foci are

Given: Foci are $(0, \pm 10)$ and the length of latus rectum is 9 units

Need to find: The equation of the hyperbola.

Let, the equation of the hyperbola be:

$\frac{y^{2}}{a^{2}}-\frac{x^{2}}{b^{2}}=1$

The length of the latus rectum is 9 units.

Therefore, $\frac{2 b^{2}}{a}=9 \Rightarrow b^{2}=\frac{9}{2} a \cdots$ (1)

The foci are given at (0, ±10)

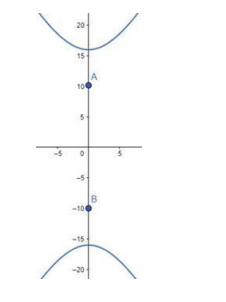
That means, ae = 10, where e is the eccentricity.

We know that,

$e=\sqrt{1+\frac{b^{2}}{a^{2}}}$

Therefore,

$\Rightarrow \sqrt{1+\frac{b^{2}}{a^{2}}}=10$

$\Rightarrow \frac{a \sqrt{a^{2}+b^{2}}}{a}=10$

$\Rightarrow a^{2}+b^{2}=100$ [Squaring both sides]

$\Rightarrow \mathrm{a}^{2}+\frac{9}{2} \mathrm{a}=100[$ From $(1)]$

$\Rightarrow 2 \mathrm{a}^{2}+9 \mathrm{a}-200=0$

$\Rightarrow 2 \mathrm{a}^{2}+25 \mathrm{a}-16 \mathrm{a}-200=0$

$\Rightarrow(2 \mathrm{a}+25)(\mathrm{a}-16)=0$

So, either $a=16$ or, $a=-\frac{25}{2}$

That means, either $b=\sqrt{\frac{9}{2} \times 16}=6 \sqrt{2}$ or, $b=\sqrt{-\frac{9 \times 25}{2 \times 2}}$

The value of $b=\sqrt{-\frac{9 \times 25}{2 \times 2}}$

is not a valid one. So, the b value and its corresponding a value is not acceptable.

Hence, the acceptable value of $a$ is 16 and $b$ is $6 \sqrt{2}$

So, the equation of the hyperbola is,

$\frac{\mathrm{y}^{2}}{\mathrm{a}^{2}}-\frac{\mathrm{x}^{2}}{\mathrm{~b}^{2}}=1 \Rightarrow \frac{\mathrm{y}^{2}}{256}-\frac{\mathrm{x}^{2}}{72}=1$ [Answer]