Find the area under the given curves and given lines:

Solution:

i. The required area is represented by the shaded area ADCBA as

Area $\mathrm{ADCBA}=\int_{1}^{2} y d x$

$=\int_{1}^{2} x^{2} d x$

$=\left[\frac{x^{3}}{3}\right]_{1}^{2}$

$=\frac{8}{3}-\frac{1}{3}$

$=\frac{7}{3}$ units

ii. The required area is represented by the shaded area ADCBA as\

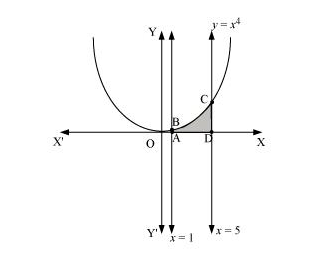
Area $\mathrm{ADCBA}=\int_{1}^{5} x^{4} d x$

$=\left[\frac{x^{5}}{5}\right]_{1}^{5}$

$=\frac{(5)^{5}}{5}-\frac{1}{5}$

$=(5)^{4}-\frac{1}{5}$

$=625-\frac{1}{5}$

$=624.8$ units