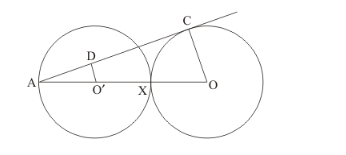
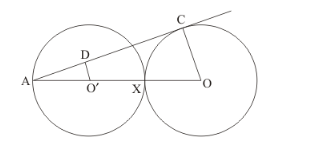
Equal circles with centres $\mathrm{O}$ and $\mathrm{O}^{\prime}$ touch each other at $\mathrm{X}$. OO' produced to meet a circle with centre $\mathrm{O}^{\prime}$, at $\mathrm{A}$. AC is a tangent to the circle whose centre is $\mathrm{O}$. O'D is perpendicular to AC. Find the value of $\frac{D O^{\prime}}{C O}$.
Consider the two triangles 
and 
.
We have,
is a common angle for both the triangles.
(Given in the problem)
(Since OC is the radius and AC is the tangent to that circle at C and we know that the radius is always perpendicular to the tangent at the point of contact)
Therefore,
From AA similarity postulate we can say that,
~
Since the triangles are similar, all sides of one triangle will be in same proportion to the corresponding sides of the other triangle.
Consider AO′ of and AO of .
$\frac{A O^{\prime}}{A O}=\frac{A O^{\prime}}{A O^{\prime}+O^{\prime} X+O X}$
Since AO′ and O′X are the radii of the same circle, we have,
AO′ = O′X
Also, since the two circles are equal, the radii of the two circles will be equal. Therefore,
AO′ = XO
Therefore we have
$\frac{A O^{\prime}}{A O}=\frac{A O^{\prime}}{A O^{\prime}+A O^{\prime}+O^{\prime} A}$
$\frac{A O^{\prime}}{A O}=\frac{1}{3}$
Since $\triangle A C O \sim \Delta A D O^{\prime}$,
$\frac{A O^{\prime}}{A O}=\frac{D O}{C O}$
We have found that,
$\frac{A O^{\prime}}{A O}=\frac{1}{3}$
Therefore,
$\frac{D O^{\prime}}{C O}=\frac{1}{3}$