Eight chairs are numbered 1 to 8.

Solution:

We know that,

nPr

According to the question,

W1 can occupy chairs marked 1 to 4 in 4 different way.

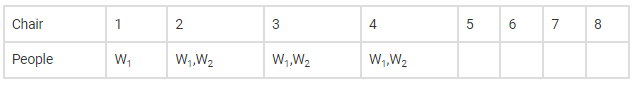
W2 can occupy 3 chairs marked 1 to 4 in 3 different ways.

So, total no of ways in which women can occupy the chairs,

4P2 = 4!/(4 – 2)!

= (4 × 3 × 2 × 1)/(2 × 1)

4P2 = 12

Now, 6 chairs will be remaining.

M1 can occupy any of the 6 chairs in 6 different ways,

M2 can occupy any of the remaining 5 chairs in 5 different ways

M3 can occupy any of the remaining 4 chairs in 4 different ways.

So, total no of ways in which men can occupy the chairs,

6P3 = 6!/(6 – 3)!

=120

Hence, total number of ways in which men and women can be seated

4P2x6P3 = 120 × 12

=1440