Question:
Construct a ∆ABC in which base AB = 5 cm, ∠A = 30° and AC – BC = 2.5 cm. Justify your construction.
Solution:
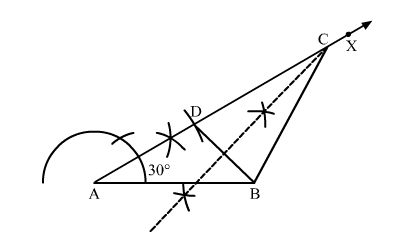
Steps of construction:
1. Draw a line segment AB = 5 cm.
2. Construct $\angle B A X=30^{\circ}$.
3. Set off AD = 2.5 cm.
4. Join DB.
5. Draw the right bisector of DB, meeting DB produced at C.
6. Join CB.
Thus, $\triangle A B C$ is the required triangle.
Justification:
Point C lies on the perpendicular bisector of DB.
⇒ CD = BC
Now
AD = AC − DC = AC − BC
