Compute the median for each of the following data:

(i)

We prepare the cumulative frequency table, as given below.

Now, we have

$N=100$

So, $\frac{N}{2}=50$

Now, the cumulative frequency just greater than 50 is 65 and the corresponding class is $70-90$.

Therefore, $70-90$ is the median class.

Here, $l=70, f=22, F=43$ and $h=20$

We know that

Median $=l+\left\{\frac{\frac{N}{2}-F}{f}\right\} \times h$

$=70+\left\{\frac{50-43}{22}\right\} \times 20$

$=70+\frac{7 \times 20}{22}$

$=70+6.36$

$=76.36$

Hence, the median is 76.36.

Note: The first class in the table can be omitted also.

(ii)

We prepare the cumulative frequency table, as given below.

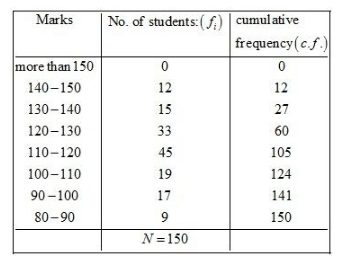
Now, we have

$N=150$

So. $\frac{N}{2}=75$

Thus, the cumulative frequency just greater than 75 is 105 and the corresponding class is $110-120$.

Therefore, $110-120$ is the median class.

$l=120, f=45, F=60$ and

$h=-10$ (Because class interval given in descending order)

We know that

Median $=l+\left\{\frac{\frac{N}{2}-F}{f}\right\} \times h$

$=120+\left\{\frac{75-60}{45}\right\} \times(-10)$

$=120-\frac{15 \times 10}{45}$

$=120-\frac{150}{45}$

$=120-3.333$

$=116.67$ (approx)

Hence, the median is 116.67.