Question:
AD is a median of the ΔABC. Is it true that AB + BC +CA > 2AD? Give reason for your answer.
Solution:
Yes,
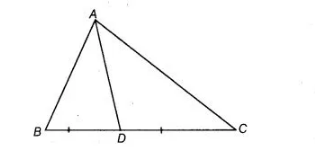
In $\triangle A B D$, we have $A B+B D>A D$$\ldots($ i)
[sum of any two sides of a triangle is greater than third side]
In $\triangle A C D$, we have $A C+C D>A D$ ....(ii)
[sum of any two sides of a triangle is greater than third side]
On adding Eqs. (i) and (ii), we get
$(A B+B D+A C+C D)>2 A D$
$\Rightarrow \quad(A B+B D+C D+A C)>2 A D$
Hence, $A B+B C+A C>2 A D$ $[\because B C=B D+C D]$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.