AC voltage $V(t)=20 \sin \omega t$ of frequency $50 \mathrm{~Hz}$ is applied to a parallel plate capacitor. The separation between the plates is $2 \mathrm{~mm}$ and the area is $1 \mathrm{~m}^{2}$. The amplitude of the oscillating displacement current for the applied AC voltage is_________.
[Take $\varepsilon_{0}=8.85 \times 10^{-12} \mathrm{~F} / \mathrm{m}$ ]
Correct Option: , 3
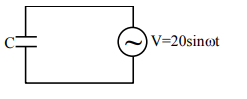
From the given information,
$\mathrm{C}=\frac{\epsilon_{0} \mathrm{~A}}{\mathrm{~d}}=\frac{\epsilon_{0} \times 1}{2 \times 10^{-3}} \mathrm{~F}$
$\therefore X_{C}=\frac{1}{\omega C}=\frac{2 \times 10^{-3}}{2 \times 50 \pi \times \epsilon_{0}}=\frac{2 \times 10^{-3}}{25 \times 4 \pi \epsilon_{0}} \Omega$
$\therefore X_{C}=\frac{2 \times 10^{-3}}{25} \times 9 \times 10^{9}=\frac{18}{25} \times 10^{6} \Omega$
$\therefore \mathrm{i}_{0}=\frac{\mathrm{V}_{0}}{\mathrm{X}_{\mathrm{C}}}=\frac{20 \times 25}{18} \times 10^{-6} \mathrm{~A}=27.47 \mu \mathrm{A}$
The value of amplitude of displacement current will be same as value of amplitude of conventional current.
Hence option 3 .
