Question:
ABCD is a parallelogram and E is a point on BC. If the diagonal BD intersects AE at F, prove that AF × FB = EF × FD.
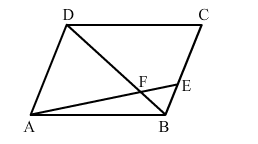
Solution:
We have:
$\angle A F D=\angle E F B \quad$ (Vertically Opposite angles)
$\because \mathrm{DA} \| \mathrm{BC}$
$\therefore \angle D A F=\angle B E F \quad$ (Alternate angles)
$\triangle D A F \sim \triangle B E F$ (AA similarity theorem)
$\Rightarrow \frac{A F}{E F}=\frac{F D}{F B}$
or, $A F \times F B=F D \times E F$
This completes the proof.
