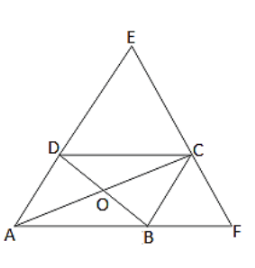
ABCD is a parallelogram, AD is produced to E so that DE = DC and EC produced meets AB produced in F.

Draw a parallelogram ABCD with AC and BD intersecting at O.

Produce AD to E such that DE = DC

Join EC and produce it to meet AB produced at F.

In ΔDCE,

∠DCE = ∠DEC ...  (i)  [In a triangle, equal sides have equal angles]

AB ∥ CD   [Opposite sides of the parallelogram are parallel]

∴ AE ∥ CD   [AB lies on AF]

AF∥CD and EF is the Transversal.

∠DCE = ∠BFC ... (ii)   [Pair of corresponding angles]

From (i) and (ii) we get

∠DEC = ∠BFC

In ΔAFE,

∠AFE = ∠AEF   [∠DEC = ∠BFC]

Therefore, AE = AF   [In a triangle, equal angles have equal sides opposite to them]

⟹ AD + DE = AB + BF

⟹ BC + AB = AB + BF   [Since, AD = BC, DE = CD and CD = AB, AB = DE]

⟹ BC = BF

Hence proved.