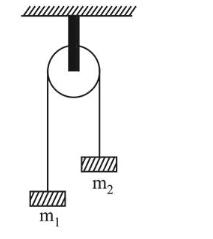
A uniformly thick wheel with moment of inertia I and radius $R$ is free to rotate about its centre of mass (see fig). A massless string is wrapped over its rim and two blocks of masses $m_{1}$ and $m_{2}\left(m_{1}>m_{2}\right)$ are attached to the ends of the string. The system is released from rest. The angular speed of the wheel when $m_{1}$ descents by a distance $h$ is:
Correct Option: 1
(1) Using principal of conservation of energy
$\left(m_{1}-m_{2}\right) g h=\frac{1}{2}\left(m_{1}+m_{2}\right) v^{2}+\frac{1}{2} I \omega^{2}$
$\Rightarrow \quad\left(m_{1}-m_{2}\right) g h=\frac{1}{2}\left(m_{1}+m_{2}\right)(\omega R)^{2}+\frac{1}{2} I \omega^{2}$
$(\because v=\omega R)$
$\Rightarrow \quad\left(m_{1}-m_{2}\right) g h=\frac{\omega^{2}}{2}\left[\left(m_{1}+m_{2}\right) R^{2}+I\right]$
$\Rightarrow \omega=\sqrt{\frac{2\left(m_{1}-m_{2}\right) g h}{\left(m_{1}+m_{2}\right) R^{2}+I}}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.