Question:
A rectangular plot is given for constructing a house having a measurement of 40 m long and 15 m in the front. According to the laws, a minimum of 3 m,
wide space should be left in the front and back each and 2 m wide space on each of other sides. Find the largest area where house can be constructed.
Solution:
Let ABCD is a rectangular plot having a measurement of 40 m long and 15 m front.
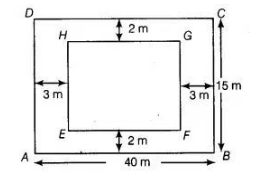
∴ Length of inner-rectangle, EF = 40 – 3 – 3 = 34 m and breadth of inner-rectangle, FG =15 – 2 – 2 = 11 m
∴ Another rectangle EFGH will be formed inside the rectangle ABCD
∴ Area of innerrectangle, EFGH = Length x Breadth
= EF x FG = 34 x 11 = 374 m2
[∴ area of a rectangle = length x breadth]
Hence, the largest area where the house can be constructed in 374 m2.
