Question:
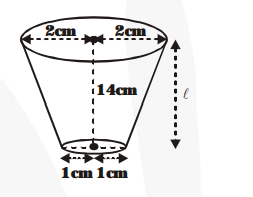
A drinking glass is in the shape of a frustum of a cone of height 14 cm. The diameters of its two circular ends are 4 cm and 2 cm. Find the capacity of the glass.
Solution:
R = 2 cm, r = 1 cm, h = 14 cm
Capacity of the glass = volume of the frustum with radii of ends as 2 cm and 1 cm and height 14 cm
$=\frac{\mathbf{1}}{\mathbf{3}} \pi \mathrm{h}\left\{\mathrm{R}^{2}+\mathrm{r}^{2}+\mathrm{Rr}\right\}$
$=\frac{\mathbf{1}}{\mathbf{3}} \pi \times 14 \times\left\{(2)^{2}\right.$$\left.+(1)^{2}+2(1)\right\} \mathrm{cm}^{3}$
$=\frac{\mathbf{1}}{\mathbf{3}} \times \frac{\mathbf{2 2}}{\mathbf{7}} \times 14 \times 7 \mathrm{~cm}^{3}$
$=\frac{308}{3}=102 \frac{2}{3} \mathrm{~cm}^{3}$
