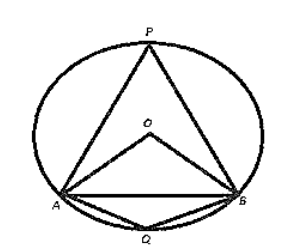
Question:
A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
Solution:
We have,
Radius OA = Chord AB
⟹ OA = OB = AB
Then triangle OAB is an equilateral triangle.
∴ ∠AOB = 60° [one angle of equilateral triangle]
By degree measure theorem
∠AOB = 2∠APB
⇒ 60° = 2∠APB
⇒ ∠APB = 60°/2 = 30°
Now, ∠APB + ∠AQB = 180° [opposite angles of cyclic quadrilateral]
⇒ 300 + ∠AQB = 180°
⇒ ∠AQB = 180° − 30° = 150°.
Therefore, Angle by chord AB at minor arc = 150°
Angle by chord AB at major arc = 30