A car accelerates from rest at a constant rate $\alpha$ for some time after which it decelerates at a constant rate $\beta$ to come to rest. If the total time elapsed is $t$ seconds, the total distance travelled is :
Correct Option: , 3
$\mathrm{v}_{0}=\alpha \mathrm{t}_{1}$ and $0=\mathrm{v}_{0}-\beta \mathrm{t}_{2} \Rightarrow \mathrm{v}_{0}=\beta \mathrm{t}_{2}$
$\mathrm{t}_{1}+\mathrm{t}_{2}=\mathrm{t}$
$\mathrm{v}_{0}\left(\frac{1}{\alpha}+\frac{1}{\beta}\right)=\mathrm{t}$
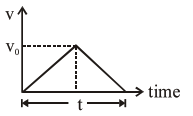
$\Rightarrow \mathrm{v}_{0}=\frac{\alpha \beta \mathrm{t}}{\alpha+\beta}$
Distance $=$ area of $v-t$ graph
$=\frac{1}{2} \times \mathrm{t} \times \mathrm{v}_{0}=\frac{1}{2} \times \mathrm{t} \times \frac{\alpha \beta \mathrm{t}}{\alpha+\beta}=\frac{\alpha \beta \mathrm{t}^{2}}{2(\alpha+\beta)}$
