A block of mass m slides on the wooden wedge, which in turn slides backward on the horizontal surface.

Let acceleration of wedge is $\mathrm{a}_{1}$ and acceleration of block w.r.t. wedge is $\mathrm{a}_{2}$

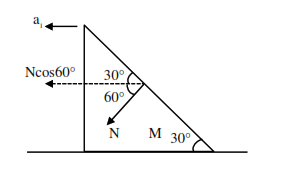
$N \cos 60^{\circ}=M a_{1}=16 a_{1}$

$\Rightarrow \mathrm{N}=32 \mathrm{a}_{1}$

F.B.D. of block w.r.t wedge

$\perp$ to incline

$\mathrm{N}=8 \mathrm{~g} \cos 30^{\circ}-8 \mathrm{a}_{1} \sin 30^{\circ} \Rightarrow 32 \mathrm{a}_{1}=4 \sqrt{3} \mathrm{~g}-4 \mathrm{a}_{1}$

$\Rightarrow \mathrm{a}_{1}=\frac{\sqrt{3}}{9} \mathrm{~g}$

Along incline

$8 g \sin 30^{\circ}+8 \mathrm{a}_{1} \cos 30^{\circ}=\mathrm{ma}_{2}=8 \mathrm{a}_{2}$

$\mathrm{a}_{2}=\mathrm{g} \times \frac{1}{2}+\frac{\sqrt{3}}{9} \mathrm{~g} \cdot \frac{\sqrt{3}}{2}=\frac{2 \mathrm{~g}}{3}$

Option (4)