Question:
A 2 m ladder leans against a vertical wall. If the top of the ladder begins to slide down the wall at the rate $25 \mathrm{~cm} / \mathrm{sec}$., then the rate (in $\mathrm{cm} / \mathrm{sec}$.) at which the bottom of the ladder slides away from the wall on the horizontal ground when the top of the ladder is $1 \mathrm{~m}$ above the ground is :
Correct Option: , 3
Solution:
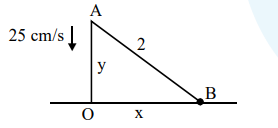
$x^{2}+y^{2}=4\left(\frac{d y}{d t}=-25\right)$
$x \frac{d x}{d t}+y \frac{d y}{d t}=0$
$\sqrt{3} \frac{d x}{d t}-1(25)=0$
$\frac{\mathrm{dx}}{\mathrm{dt}}=\frac{25}{\sqrt{3}} \mathrm{~cm} / \mathrm{sec}$
