JEE Advanced Previous Year Questions of Math with Solutions are available at eSaral. Practicing JEE Advanced Previous Year Papers Questions of mathematics will help the JEE aspirants in realizing the question pattern as well as help in analyzing weak & strong areas.
eSaral helps the students in clearing and understanding each topic in a better way. eSaral also provides complete chapter-wise notes of Class 11th and 12th both for all subjects.
Besides this, eSaral also offers NCERT Solutions, Previous year questions for JEE Main and Advance, Practice questions, Test Series for JEE Main, JEE Advanced and NEET, Important questions of Physics, Chemistry, Math, and Biology and many more.
Download eSaral app for free study material and video tutorials.
Paragraph for Question 5 and 6
Let $f(\mathrm{x})=(1-\mathrm{x})^{2} \sin ^{2} \mathrm{x}+\mathrm{x}^{2}$ for all $\mathrm{x} \in \mathbb{R},$ and let $\mathrm{g}(\mathrm{x})=\int_{1}^{\mathrm{x}}\left(\frac{2(\mathrm{t}-1)}{\mathrm{t}+1}-\ell \mathrm{nt}\right) f(\mathrm{t}) \mathrm{d} \mathrm{t}$ for all $\mathrm{x} \in$ $(1, \infty)$
Let $\mathrm{f}(\mathrm{x})=\mathrm{x}+\log _{\mathrm{e}} \mathrm{x}-\mathrm{x} \log _{\mathrm{e}} \mathrm{x}, \mathrm{x} \in(0,0)$
$*$ Column 1 contains information about zeros of $f(\mathrm{x}), f^{\prime}(\mathrm{x})$ and $f^{\prime \prime}(\mathrm{x})$
$*$ Column 2 contains information about the limiting behavior of $f(\mathrm{x}), f^{\prime}(\mathrm{x})$ and $$ f^{\prime \prime}(\mathrm{x}) \text { at infinity. } $$
$*$ Column 3 contains information about increasing/decreasing nature of $f(\mathrm{x})$ an
$$ f^{\prime}(\mathrm{x}) $$
Q. For the function $f(x)=x \cos \frac{1}{x}, x \geq 1$
(A) for at least one $x$ in the interval $[1, \infty), f(x+2)-f(x)<2$
(B) $\lim _{x \rightarrow \infty} f^{\prime}(x)=1$
(C) for all $x$ in the interval $[1, \infty), f(x+2)-f(x)>2$
(D) $f^{\prime}(x)$ is strictly decreasing in the interval $[1, \infty)$
[JEE 2009, 4]
Ans. (B,C,D)
$\mathrm{f}(\mathrm{x})=\mathrm{x} \cos \frac{1}{\mathrm{x}}, \mathrm{x} \geq 1$
$\mathrm{f}^{\prime}(\mathrm{x})=\cos \frac{1}{\mathrm{x}}+\frac{1}{\mathrm{x}} \sin \frac{1}{\mathrm{x}}$
$\lim _{x \rightarrow \infty} \mathrm{f}^{\prime}(\mathrm{x})=1$
$\mathrm{Now} \quad \mathrm{f}^{\prime \prime}(\mathrm{x})=-\sin \frac{1}{\mathrm{x}} \times-\frac{1}{\mathrm{x}^{2}}-\frac{1}{\mathrm{x}^{2}} \sin \frac{1}{\mathrm{x}}+\frac{1}{\mathrm{x}} \cos \frac{1}{\mathrm{x}} \times-\frac{1}{\mathrm{x}^{2}}$
$\therefore \mathrm{f}^{\prime}(\mathrm{x}+2)<\mathrm{f}^{\prime}(\mathrm{x})$
$\therefore \mathrm{f}^{\prime}(\mathrm{x}+2)<\mathrm{f}^{\prime}(\mathrm{x})$
Also $\lim _{\mathrm{x} \rightarrow \infty} \mathrm{f}(\mathrm{x}+2)-\mathrm{f}(\mathrm{x})$
$=\lim _{x \rightarrow \infty}\left[(x+2) \cos \frac{1}{(x+2)}-x \cos \frac{1}{x}\right]=2$
$\therefore f(x+2)-f(x)>2 \quad \forall x \geq 1$
Q. Let $f$ be a real-valued function defined on the interval $(0, \infty)$ by $\mathrm{f}(\mathrm{x})=\ell \mathrm{nx}+\int_{0}^{\mathrm{x}} \sqrt{1+\sin \mathrm{t} \mathrm{d} \mathrm{t}} \cdot$ Then which of the following statement (s) is/(are) true?
(A) $\mathrm{f}^{\prime \prime}(\mathrm{x})$ exists for all $\mathrm{x} \in(0, \infty)$
(B) $\mathrm{f}^{\prime}(\mathrm{x})$ exists for all $\mathrm{x} \in(0, \infty)$ and $\mathrm{f}^{\prime}$ is continuous on $(0, \infty),$ but not differentiable on $(0, \infty)$
(C) there exists $\alpha>1$ such that $\left|\mathrm{f}^{\prime}(\mathrm{x})\right|<|\mathrm{f}(\mathrm{x})|$ for all $\mathrm{x} \in(\alpha, \infty)$
(D) there exists $\beta>0$ such that $|\mathrm{f}(\mathrm{x})|+\left|\mathrm{f}^{\prime}(\mathrm{x})\right| \leq \beta$ for all $\mathrm{x} \in(0, \infty)$
[JEE 10, 3M]
Ans. (B,C)
$\mathrm{f}(\mathrm{x})=\ln \mathrm{x}+\int_{0}^{\mathrm{x}} \sqrt{1+\sin \mathrm{t}} \mathrm{d} \mathrm{t}$
$\mathrm{f}^{\prime}(\mathrm{x})=\frac{1}{\mathrm{x}}+\sqrt{1+\sin \mathrm{x}}$
$\mathrm{f}^{\prime}(\mathrm{x})=\frac{1}{\mathrm{x}}+\sqrt{2}\left|\cos \left(\frac{\mathrm{x}}{2}-\frac{\pi}{4}\right)\right|$
$\because\left|\cos \left(\frac{\mathrm{x}}{2}-\frac{\pi}{4}\right)\right|$ is non-derivable
$\therefore \mathrm{f}^{\prime}(\mathrm{x})$ is non-derivable but continuous.
hence option (A) is incorrect \& option (B) is correct.
For option $\mathrm{C}$
$\mathrm{f}(\mathrm{x})=(\ell \mathrm{nx})+\int_{0}^{\mathrm{x}}(\sqrt{1+\sin \mathrm{x}}) \mathrm{d} \mathrm{x}$
since $\mathrm{f}(\mathrm{x})$ is positive increasing function for all $\mathrm{x}>1$
$\Rightarrow|\mathrm{f}(\mathrm{x})|=\mathrm{f}(\mathrm{x}) \&\left|\mathrm{f}^{\prime}(\mathrm{x})\right|=\mathrm{f}^{\prime}(\mathrm{x})$
Let $\mathrm{f}(\mathrm{x})=\mathrm{y}$
$\mathrm{f}^{\prime}(\mathrm{x})-\mathrm{f}(\mathrm{x})=\frac{1}{\mathrm{x}}-\ell \mathrm{nx}+\sqrt{1+\sin \mathrm{x}}-\int_{0}^{\mathrm{x}} \sqrt{1+\sin \mathrm{t}} \mathrm{d} \mathrm{t}$
$\mathrm{f}^{(\mathrm{x})}-\mathrm{f}(\mathrm{x})=\frac{1}{\mathrm{x}}-\ln \mathrm{x}+\sqrt{1+\sin \mathrm{x}}-\sqrt{2} \int_{0}^{\mathrm{x}}\left(\frac{\mathrm{t}}{2}-\frac{\pi}{4}\right) \| \mathrm{dt}$
$\frac{1}{\mathrm{x}}-\ln \mathrm{x}<0 ;$ when $\alpha>\mathrm{e}$
$0 \leq \sqrt{1+\sin \mathrm{x}} \leq \sqrt{2}$
$\int_{0}^{\mathrm{x}}\left|\cos \left(\frac{\mathrm{t}}{2}-\frac{\pi}{4}\right)\right| \mathrm{dt}>\sqrt{2} \forall \alpha>\frac{3 \pi}{2}$
$\Rightarrow \mathrm{f}^{\prime}(\mathrm{x})-\mathrm{f}(\mathrm{x})<0 \quad \forall \quad \alpha>\frac{3 \pi}{2}>1$
Hence option (C) is correct.
For option (D) $|\mathrm{f}(\mathrm{x})|+\left|\mathrm{f}^{\prime}(\mathrm{x})\right| \rightarrow \infty$
when $\mathrm{x} \rightarrow \infty$
Therefore option (D) is incorrect.
Alternate :
$\mathrm{f}(\mathrm{x})=\ln \mathrm{x}+\int_{0}^{\mathrm{x}} \sqrt{1+\sin \mathrm{t}} \mathrm{dt}$
$\mathrm{f}^{\prime}(\mathrm{x})=\frac{1}{\mathrm{x}}+\sqrt{1+\sin \mathrm{x}}$ ........(i)
for $\mathrm{x}>1$
$\frac{1}{\mathrm{x}}+\sqrt{1+\sin \mathrm{x}}<1+\sqrt{2}$
but $\ell \mathrm{nx}+\int_{0}^{\mathrm{x}} \sqrt{1+\sin \mathrm{t}} \mathrm{dt}$ will always be more than $1+\sqrt{2}$ for some $\alpha>1$
$\because \int_{0}^{x} \sqrt{1+\sin t}>0 \quad \& \quad \ln x$ is increasing in $(1, \infty)$
$\Rightarrow \quad \mathrm{f}(\mathrm{x})>\mathrm{f}^{\prime}(\mathrm{x}) \forall \alpha>1$
$\therefore(\mathrm{C})$ is correct
$\mathrm{f}^{\prime \prime}(\mathrm{x})=-\frac{1}{\mathrm{x}^{2}}+\frac{\cos \mathrm{x}}{2 \sqrt{1+\sin \mathrm{x}}}$
$\Rightarrow \mathrm{f}$ is not derivable on $(0, \infty)$
at $\frac{3 \pi}{2}, \frac{7 \pi}{2}$
$\therefore$ (B) is also correct
$\mathrm{f}(\mathrm{x})$ is unbounded near $\mathrm{x}=0$ in $(0,1)$ hence $|\mathrm{f}(\mathrm{x})|$ can never be made less
than a finite number hence $|\mathrm{f}(\mathrm{x})|+\left|\mathrm{f}^{\prime}(\mathrm{x})\right|$ can never be less than $\beta$
Q. Let $f:(0,1) \rightarrow \mathrm{R}$ be defined by $f(\mathrm{x})=\frac{\mathrm{b}-\mathrm{x}}{1-\mathrm{bx}},$ where b is a constant such that $0<\mathrm{b}<1 .$ Then
(A) $f$ is not invertible on $(0,1)$
(B) $f \neq f^{-1}$ on $(0,1)$ and $f^{\prime}(b)=\frac{1}{f^{\prime}(0)}$
(C) $f=f^{-1}$ on $(0,1)$ and $f^{\prime}(b)=\frac{1}{f^{\prime}(0)}$
(D) $f^{-1}$ is differentiable on $(0,1)$
[JEE 2011, 4M]
Ans. (A)
$f:(0,1) \rightarrow \mathrm{R}$
$f(\mathrm{x})=\frac{\mathrm{b}-\mathrm{x}}{1-\mathrm{bx}}$
$\mathrm{b} \in(0,1)$
$\Rightarrow \quad f^{\prime}(x)=\frac{b^{2}-1}{(b x-1)^{2}}$
$\Rightarrow f^{\prime}(x)<0 \forall x \in(0,1)$
hence $f(x)$ is decreasing function
hence its range $(-1, b)$
$\Rightarrow$ co-domain $\neq$ range
$\Rightarrow f(x)$ is non-invertible function
Q. The number of distinct real roots of $x^{4}-4 x^{3}+12 x^{2}+x-1=0$ is
[JEE 2011, 4M]
Ans. 2
Let $f(x)=x^{4}-4 x^{3}+12 x^{2}+x-1$
$f^{\prime}(x)=4 x^{3}-12 x^{2}+24 x$
$f^{\prime \prime}(x)=12 x^{2}-24 x+24$
$=12\left(x^{2}-2 x+2\right)>0$
$\Rightarrow f^{\prime}(x)$ is strictly increasing function
$\because f^{\prime}(x)$ is cubic polynomial
hence number of roots of $f^{\prime}(x)=0$ is 1
$\Rightarrow$ Number of maximum roots of $f(x)=0$ are 2
Now $f(0)=-1, f(1)=9, f(-1)=15$
$\Rightarrow f(x)$ has exactly 2 distinct real roots.
Q. Consider the statements :
$\mathbf{P}:$ There exists some $\mathrm{x} \in \mathbb{R}$ such that $f(\mathrm{x})+2 \mathrm{x}=2\left(1+\mathrm{x}^{2}\right)$
$\mathrm{Q}:$ There exists some $\mathrm{x} \in \mathbb{R}$ such that $2 f(\mathrm{x})+1=2 \mathrm{x}(1+\mathrm{x})$ $\quad$ Then
(A) both P and Q are true
(B) P is true and Q is false
(C) P is false and Q is true
(D) both P and Q are false
[JEE 2012, 3M, –1M]
Ans. (C)
$f(x)=(1-x)^{2} \sin ^{2} x+x^{2}$
$P: f(x)+2 x=2\left(1+x^{2}\right)$
$\Rightarrow(1-x)^{2} \sin ^{2} x+x^{2}+2 x=2+2 x^{2}$
$\Rightarrow(1-x)^{2} \sin ^{2} x-x^{2}+2 x-2=0$
$(1-x)^{2} \cos ^{2} x+1=0$
which is not possible.
$\therefore \mathrm{P}$ is false.
$\mathrm{Q}: 2 f(\mathrm{x})+1=2 \mathrm{x}(1+\mathrm{x})$
$2 \mathrm{x}^{2}+2(1-\mathrm{x})^{2} \sin ^{2} \mathrm{x}+1=2 \mathrm{x}^{2}+2 \mathrm{x}$
$2(1-\mathrm{x})^{2} \sin ^{2} \mathrm{x}-2 \mathrm{x}+1=0$
Let $\mathrm{h}(\mathrm{x})=2(1-\mathrm{x})^{2} \sin ^{2} \mathrm{x}-2 \mathrm{x}+1$
clearly $\mathrm{h}(1)=-1$
and $\mathrm{h}(\mathrm{x})=2\left(\mathrm{x}^{2}-2 \mathrm{x}+1\right) \sin ^{2} \mathrm{x}-2 \mathrm{x}+1$
$=\mathrm{x}^{2}\left[2\left(1-\frac{2}{\mathrm{x}}+\frac{1}{\mathrm{x}^{2}}\right) \cdot \sin ^{2} \mathrm{x}-\frac{2}{\mathrm{x}}+\frac{1}{\mathrm{x}^{2}}\right]$
$\therefore \mathrm{h}(\mathrm{x}) \rightarrow \infty$ as $\mathrm{x} \rightarrow \infty$
$\therefore$ By intermediate value theorem
$\mathrm{h}(\mathrm{x})=0$ has a root which is greater than 1
Hence $\mathrm{Q}$ is true.
Q. Which of the following is true ?
(A) $\mathrm{g}$ is increasing on $(1, \infty)$
(B) $\mathrm{g}$ is decreasing on $(1, \infty)$
(C) $\mathrm{g}$ is increasing on $(1,2)$ and decreasing on $(2, \infty)$
(D) $\mathrm{g}$ is decreasing on $(1,2)$ and increasing on $(2, \infty)$
[JEE 2012, 3M, –1M]
Ans. (B)
$\mathrm{g}(\mathrm{x})=\int_{1}^{\mathrm{x}}\left(\frac{2(\mathrm{t}-1)}{(\mathrm{t}+1)}-\ell \mathrm{nt}\right) f(\mathrm{t}) \mathrm{dt}$
$g^{\prime}(x)=\left(\frac{2(x-1)}{x+1}-\ln x\right) f(x)$
$f(\mathrm{x})>0 \quad \forall \mathrm{x} \in \mathrm{R}$
Suppose.
$h(x)=\frac{2(x-1)}{x+1}-\ln x$
$h(x)=2-\left(\frac{4}{x+1}+\ln x\right)$
$h^{\prime}(x)=\frac{4}{(x+1)^{2}}-\frac{1}{x}$
$h^{\prime}(x)=-\frac{(x-1)^{2}}{x(x+1)^{2}}$
$h^{\prime}(x)<0$
So h(x) is decreasing
so h(x) is decreasing
so h(x) $<0 \quad$ (1). $\quad \forall x>1$
h(x) $<0 \quad \forall x>1$
$\{h(1)=0\}$
So $g^{\prime}(x)=h(x) f(x)$
$g^{\prime}(x)<0 \quad \forall x>1$
$\mathrm{g}(\mathrm{x})$ is decreasing in $(1, \infty)$
Q. If $f(\mathrm{x})=\int_{0}^{\mathrm{x}} \mathrm{e}^{\mathrm{t}^{2}}(\mathrm{t}-2)(\mathrm{t}-3) \mathrm{dt}$ for all $\mathrm{x} \in(0, \infty),$ then $-$
(A) $f$ has a local maximum at $x=2$
(B) $f$ is decreasing on $(2,3)$
(C) there exists some $c \in(0, \infty)$ such that $f^{\prime \prime}(c)=0$
(D) $f$ has a local minimum at $x=3$
[JEE 2012, 4M]
Ans. (A,B,C,D)
$f(\mathrm{x})=\int_{0}^{\mathrm{x}} e^{\mathrm{t}^{2}}(\mathrm{t}-2)(\mathrm{t}-3) \mathrm{dt}$
$\Rightarrow f^{\prime}(x)=e^{x^{2}}(x-2)(x-3)$
$\therefore f^{\prime}(2)=f^{\prime}(3)=0$
$\Rightarrow f^{\prime \prime}(\mathrm{c})=0$ for same $\mathrm{c} \in(2,3)$ (by Rolle's theorem)
$\Rightarrow f^{\prime}(x)=e^{x^{2}}(x-2)(x-3)$
$\therefore f^{\prime}(2)=f^{\prime}(3)=0$
$\Rightarrow f^{\prime \prime}(\mathrm{c})=0$ for same $\mathrm{c} \in(2,3)$ (by Rolle's theorem)
Q. The number of points in $(-\infty, \infty),$ for which $\mathrm{x}^{2}-\mathrm{x} \sin \mathrm{x}-\cos \mathrm{x}=0,$ is
(A) 6 (B) 4 (C) 2 (D) 0
[JEE 2013, 2M]
Ans. (C)
$f(x)=x^{2}-x \sin x-\cos x$
$\quad f^{\prime}(x)=2 x-x \cos x-\sin x+\sin x$
$\quad=x(2-\cos x)$
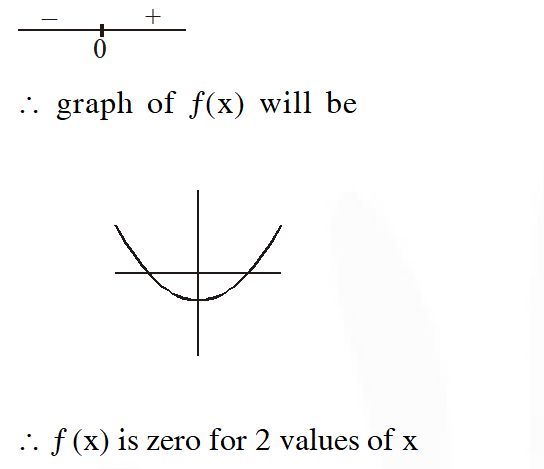
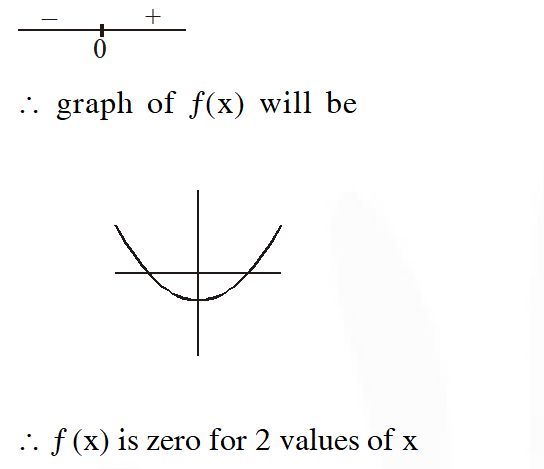
Q. Let $f(\mathrm{x})=\mathrm{x} \sin \pi \mathrm{x}, \mathrm{x}>0 .$ Then for all natural numbers $\mathrm{n}, f^{\prime}(\mathrm{x})$ vanishes at –
(A) a unique point in the interval $\left(\mathrm{n}, \mathrm{n}+\frac{1}{2}\right)$
(B) a unique point in the interval $\left(\mathrm{n}+\frac{1}{2}, \mathrm{n}+1\right)$
(C) a unique point in the interval $(\mathrm{n}, \mathrm{n}+1)$
(D) two points in the interval $(\mathrm{n}, \mathrm{n}+1)$
[JEE 2013, 4M, –1M]
Ans. (B,C)
$f^{\prime}(\mathrm{x})=\sin \pi \mathrm{x}+\pi \mathrm{x} \cos \pi \mathrm{x}=0$
$\tan \pi \mathrm{x}=-\pi \mathrm{x}$
$\mathrm{y}=\tan \mathrm{x} \pi \& \mathrm{y}=-\pi \mathrm{x}$
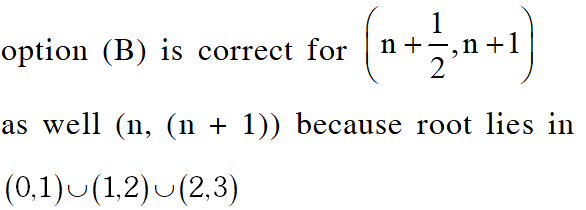
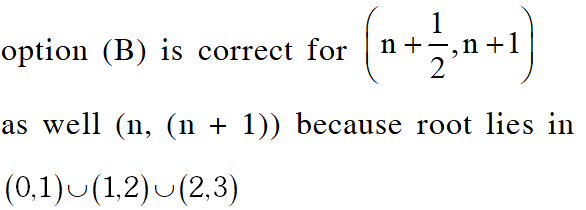
Q. Let $f, \mathrm{g}:[-1,2] \rightarrow \square$ be continuous function which are twice differentiable on the interval $(-1,2) .$ Let the values of $f$ and $\mathrm{g}$ at the points $-1,0$ and 2 be as given in the following table :
In each of the intervals (–1, 0) and (0, 2) the function (ƒ – 3g)'' never vanishes. Then the correct statement(s) is(are)
(A) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly three solutions in $(-1,0) \cup(0,2)$
(B) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly one solution in $(-1,0)$
(C) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly one solutions in $(0,2)$
(D) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly two solutions in $(-1,0)$ and exactly two
solutions in $(0,2)$
[JEE 2015, 4M, –2M]
In each of the intervals (–1, 0) and (0, 2) the function (ƒ – 3g)'' never vanishes. Then the correct statement(s) is(are)
(A) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly three solutions in $(-1,0) \cup(0,2)$
(B) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly one solution in $(-1,0)$
(C) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly one solutions in $(0,2)$
(D) $f^{\prime}(x)-3 g^{\prime}(x)=0$ has exactly two solutions in $(-1,0)$ and exactly two
solutions in $(0,2)$
[JEE 2015, 4M, –2M]
Ans. (B,C)
Let $F(x)=f(x)-3 g(x)$
$\therefore F(-1)=3 ; F(0)=3 \& F(2)=3$
$\therefore F^{\prime}(x)$ will vanish at least twice in $(-1,0) \cup(0,2)$
$\because F^{\prime \prime}(x)>0$ or $<0 \forall x \in(-1,0) \cup(0,2)$
so there will be exactly one solution in $(-1,0)$ and one in $(0,2)$
Alter
Let $\mathrm{H}(\mathrm{x})=\mathrm{f}(\mathrm{x})-3 \mathrm{g}(\mathrm{x})$
$\mathrm{H}(-1)=\mathrm{H}(0)=\mathrm{H}(2)=3$
applying Rolle's theorem in interval $[-1,0]$
$\mathrm{H}(\mathrm{x})=\mathrm{f}(\mathrm{x})-3 \mathrm{g}^{\prime}(\mathrm{x})=0$ for at least one c $\mathrm{c} \in(-1,0)$
As $\mathrm{H}^{\prime \prime}(\mathrm{x})$ never vanises in the interval
$\Rightarrow$ exactly one $\mathrm{c} \in(-1,0)$ for which $\mathrm{H}^{\prime}(\mathrm{x})=0$
similarily apply rolle's theorems in the interval $[0,2]$
$\mathrm{H}^{\prime}(\mathrm{x})=0$ has exactly one solution in $(0,2)$
Q. The total number of distinct $\mathrm{x} \in[0,1]$ for which $\int_{0}^{x} \frac{\mathrm{t}^{2}}{1+\mathrm{t}^{4}} \mathrm{dt}=2 \mathrm{x}-1$ is
[JEE(Advanced) 2016]
Ans. 1
Let $f(\mathrm{x})=\int_{0}^{\mathrm{x}} \frac{\mathrm{t}^{2}}{1+\mathrm{t}^{4}} \mathrm{dt}-2 \mathrm{x}+1$
$\Rightarrow f^{\prime}(x)=\frac{x^{2}}{1+x^{4}}-2$
as $\quad \frac{1+x^{4}}{x^{2}} \geq 2$
$\Rightarrow \frac{\mathrm{x}^{2}}{1+\mathrm{x}^{4}} \leq \frac{1}{2}$
$\Rightarrow f^{\prime}(x) \leq-\frac{3}{2}$
$\Rightarrow f(\mathrm{x})$ is continuous and decreasing
$f(0)=1$ and $f(1)=\int_{0}^{1} \frac{t^{2}}{1+t^{4}} d t-2 \leq-\frac{3}{2}$
by IVT $f(x)=0$ possesses exactly one solution in $[0,1]$
Q. Let $f(\mathrm{x})=\lim _{\mathrm{n} \rightarrow \infty}\left(\frac{\mathrm{n}^{\mathrm{n}}(\mathrm{x}+\mathrm{n})\left(\mathrm{x}+\frac{\mathrm{n}}{2}\right) \ldots .\left(\mathrm{x}+\frac{\mathrm{n}}{\mathrm{n}}\right)}{\mathrm{n} !\left(\mathrm{x}^{2}+\mathrm{n}^{2}\right)\left(\mathrm{x}^{2}+\frac{\mathrm{n}^{2}}{4}\right) \dots .\left(\mathrm{x}^{2}+\frac{\mathrm{n}^{2}}{\mathrm{n}^{2}}\right)}\right)^{\mathrm{x} / \mathrm{n}},$ for all $\mathrm{x}>0 .$ Then
(A) $f\left(\frac{1}{2}\right) \geq f(1)$
(B) $f\left(\frac{1}{3}\right) \leq f\left(\frac{2}{3}\right)$
(C) $f^{\prime}(2) \leq 0$
(D) $\frac{f^{\prime}(3)}{f(3)} \geq \frac{f^{\prime}(2)}{f(2)}$
[JEE(Advanced) 2016]
Ans. (B,C)
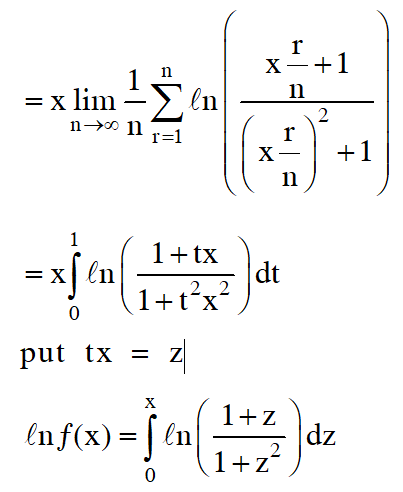
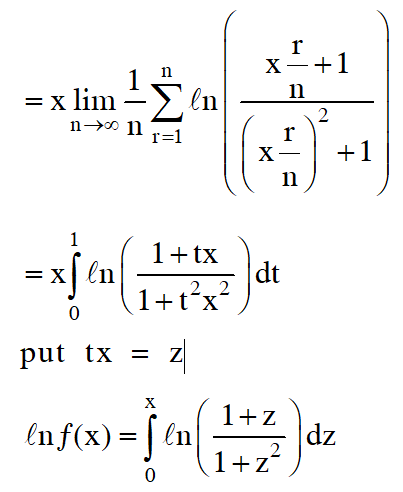
Q. Let $f: \square \rightarrow(0,1)$ be a continuous function. Then, which of the following function(s) has (have) the value zero at some point in the interval $(0,1) ?$
(A) $\mathrm{e}^{\mathrm{x}}-\int_{0}^{\mathrm{x}} f(\mathrm{t}) \sin \mathrm{td} \mathrm{t}$
(B) $\mathrm{x}^{9}-f(\mathrm{x})$
(C) $f(\mathrm{x})+\int_{0}^{\frac{\pi}{2}} f(\mathrm{t}) \sin \mathrm{td} \mathrm{t}$
(D) $\quad x-\int_{0}^{\frac{\pi}{2}-x} f(t) \cos t d t$
[JEE(Advanced) 2017]
Ans. (B,D)
For option (A),
Let $g(x)=e^{x}-\int_{0}^{x} f(t) \sin t d t$
$\therefore g^{\prime}(x)=e^{x}-(f(x) \cdot \sin x)>0 \forall x \in(0,1)$
$\Rightarrow g(x)=e^{x}-(f(x) \cdot \sin x)>0 \forall x \in(0,1)$
$\Rightarrow g(x)$ is strictly incrasing function.
Also, $g(0)=1$
$\Rightarrow \mathrm{g}(\mathrm{x})>1 \forall \mathrm{x} \in(0,1)$
$\therefore$ option $(\mathrm{A})$ is not possible.
For option $(\mathrm{B}),$ let
$\mathrm{k}(\mathrm{x})=\mathrm{x}^{9}-f(\mathrm{x})$
Now, $\mathrm{k}(0)=-f(0)<0(\mathrm{As} f \in(0,1))$
Also, $\mathrm{k}(1)=1-f(1)>0(\mathrm{As} f \in(0,1))$
$\Rightarrow \mathrm{k}(0) . \mathrm{k}(1)<0$
So, option(B) is correct.
For option (C), let
$\mathrm{T}(\mathrm{x})=f(\mathrm{x})+\int_{0}^{\frac{\pi}{2}} f(\mathrm{t}) \cdot \sin \mathrm{t} \mathrm{dt}$
$\Rightarrow \mathrm{T}(\mathrm{x})>0 \forall \mathrm{x} \in(0,1) \text { (As } f \in(0,1))$
so, option(C) is not possible.
For option (D)
Let $\mathrm{M}(\mathrm{x})=\mathrm{x}-\int_{0}^{\frac{\pi}{2}-\mathrm{x}} f(\mathrm{t}) \cos \mathrm{t} \mathrm{dt}$
$\therefore \mathrm{M}(0)=0-\int_{0}^{\pi / 2} f(\mathrm{t}) \cdot \cos \mathrm{t} \mathrm{dt}<0$
Also, $\mathrm{M}(1)=1-\int_{0}^{\frac{\pi}{2}-1} f(\mathrm{t}) \cdot \cos \mathrm{td} \mathrm{t}>0$
$\Rightarrow \mathrm{M}(0) \cdot \mathrm{M}(1)<0$
$\therefore$ option (D) is correct.
Q. Which of the following options is the only CORRECT combination ?
(A) (IV) (i) (S)
(B) (I) (ii) (R)
(C) (III) (iv) (P)
(D) (II) (iii) (S)
[JEE(Advanced) 2017]
Ans. (D)
Q. Which of the following options is the only CORRECT combination ?
(A) (III) (iii) (R)
(B) (I) (i) (P)
(C) (IV) (iv) (S)
(D) (II) (ii) (Q)
[JEE(Advanced) 2017]
Ans. (D)
Q. Which of the following options is the only INCORRECT combination ?
(A) (II) (iii) (P)
(B) (II) (iv) (Q)
(C) (I) (iii) (P)
(D) (III) (i) (R)
[JEE(Advanced) 2017]
Ans. (D)
Q. If $f: \square \rightarrow \square$ is a twice differentiable function such that $f^{\prime \prime}(x)>0$ for all $x \in \square,$ and $f\left(\frac{1}{2}\right)=\frac{1}{2}, f(1)=1,$ then
(A) $0
(B) $f^{\prime}(1) \leq 0$
(C) $f^{\prime}(1)>1$
(D) $\frac{1}{2}
[JEE(Advanced) 2017]
Ans. (C)
