JEE Advanced Previous Year Questions of Math with Solutions are available at eSaral. Practicing JEE Advanced Previous Year Papers Questions of mathematics will help the JEE aspirants in realizing the question pattern as well as help in analyzing weak & strong areas.
eSaral helps the students in clearing and understanding each topic in a better way. eSaral also provides complete chapter-wise notes of Class 11th and 12th both for all subjects.
Besides this, eSaral also offers NCERT Solutions, Previous year questions for JEE Main and Advance, Practice questions, Test Series for JEE Main, JEE Advanced and NEET, Important questions of Physics, Chemistry, Math, and Biology and many more.
Download eSaral app for free study material and video tutorials.
Q. Let A be a 2 × 2 matrix
Statement- $1: \operatorname{adj}(\operatorname{adj} A)=A$
Statement-2: $|$ adj $A|=| A |$
(1) Statement–1 is true, Statement–2 is false.
(2) Statement–1 is false, Statement–2 is true.
(3) Statement–1 is true, Statement–2 is true;Statement–2 is a correct explanation for Statement–1.
(4) Statement–1 is true, Statement–2 is true; Statement–2 is not a correct explanation for statement–1.
[AIEEE- 2009]
Ans. (4)
Let $A=\left(\begin{array}{ll}{a} & {b} \\ {c} & {d}\end{array}\right)$
$\operatorname{adj}(\mathrm{A})=\left(\begin{array}{cc}{\mathrm{d}} & {-\mathrm{b}} \\ {-\mathrm{c}} & {\mathrm{a}}\end{array}\right)$
$\operatorname{adj}(\operatorname{adj} A)=\left(\begin{array}{ll}{a} & {b} \\ {c} & {d}\end{array}\right)=A$ statement is right
Statement 2 We know $\mathrm{A}$ adj $(\mathrm{A})=|\mathrm{A}| \mathrm{I}_{\mathrm{n}}$ taking determinant $|\mathrm{A} \cdot \operatorname{adj}(\mathrm{A})|=\| \mathrm{A}\left|\mathrm{I}_{\mathrm{n}}\right|$
$\Rightarrow|\operatorname{adj}(\mathrm{A})|=|\mathrm{A}|^{\mathrm{n}-1}$
Here $\mathrm{n}=2$ (order)
so $|\operatorname{adj} \mathrm{A}||\mathrm{A}|^{2-1}=|\mathrm{A}|$
so statement 2 is also true and 2 is not explanation for statement 1
Q. The number of 3× 3 non-singular matrices, with four entries as 1 and all other entries as 0, is :-
(1) Less than 4 (2) 5 (3) 6 (4) At least 7
[AIEEE-2010]
Ans. (4)
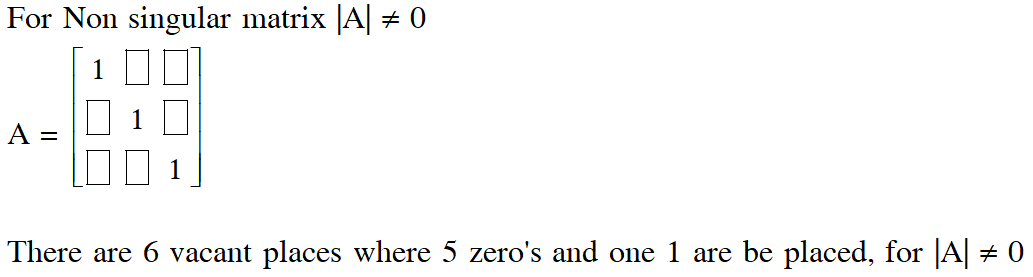
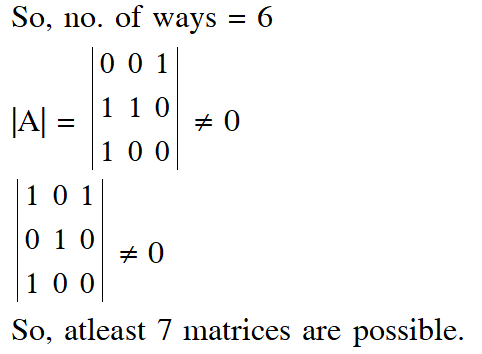
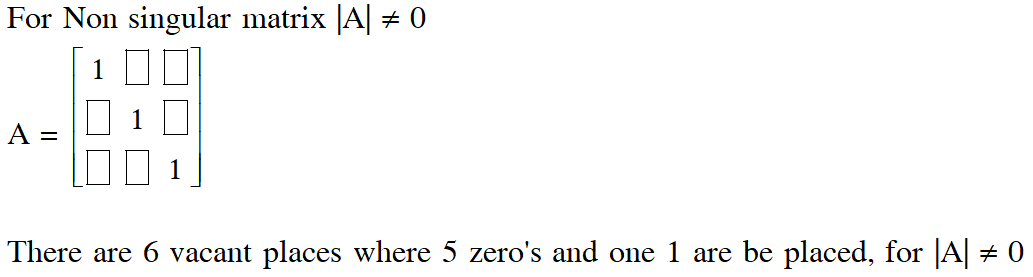
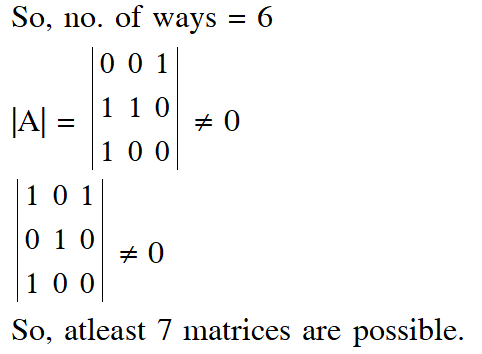
Q. Let A be a $2 \times 2$ matrix with non-zero entries and let $\mathrm{A}^{2}=\mathrm{I},$ where I is $2 \times 2$ identity matrix. Define $\operatorname{Tr}(\mathrm{A})=$ sum of diagonal elements of $\mathrm{A}$ and $|\mathrm{A}|=$ determinant of matrix $\mathrm{A}$. Statement- $1: \operatorname{Tr}(\mathrm{A})=0$
Statement-2: $|\mathrm{A}|=1$
(1) Statement–1 is true, Statement–2 is true; Statement–2 is a correct explanation for
Statement–1.
(2) Statement–1 is true, Statement–2 is true; Statement–2 is not a correct explanation for
statement–1.
(3) Statement–1 is true, Statement–2 is false.
(4) Statement–1 is false, Statement–2 is true.
[AIEEE-2010]
Ans. (3)
Statement 1:
Let $\mathrm{A}=\left(\begin{array}{ll}{\mathrm{a}} & {\mathrm{b}} \\ {\mathrm{c}} & {\mathrm{d}}\end{array}\right) \mathrm{a}, \mathrm{b}, \mathrm{c}, \mathrm{d},$ are non zero
$A^{2}=\left(\begin{array}{ll}{a} & {b} \\ {c} & {d}\end{array}\right)\left(\begin{array}{ll}{a} & {b} \\ {c} & {d}\end{array}\right)=\left(\begin{array}{ll}{1} & {0} \\ {0} & {1}\end{array}\right)$
$\Rightarrow a^{2}+b c=1$
$\Rightarrow \mathrm{ab}+\mathrm{bd}=0 \Rightarrow \mathrm{b}(\mathrm{a}+\mathrm{d})=0$
so $\mathrm{b} \neq 0,(\mathrm{a}+\mathrm{d})=0$
$a+d=0 \Rightarrow \operatorname{tr}(A)=0$
Statement 2:
\[ |A|=a d-b c=-a^{2}-b c=-\left(a^{2}+b c\right)=-1 \]
So Statement 2 is false.
Q. Let A and B be two symmetric matrices of order 3.
Statement-1 : A(BA) and (AB)A are symmetric matrices.
Statement-2 : AB is symmetric matrix if matrix multiplication of A with B is commutative.
(1) Statement-1 is true, Statement-2 is false.
(2) Statement-1 is false, Statement-2 is true
(3) Statement-1 is true, Statement-2 is true; Statement-2 is a correct explanation for Statement-1
(4) Statement-1 is true, Statement-2 is true; Statement-2 is not a correct explanation for Statement-1.
[AIEEE-2011]
Ans. (4)
$A^{T}=A$
$\mathrm{B}^{\mathrm{T}}=\mathrm{B}$
Statement- 1 $(\mathrm{A}(\mathrm{BA}))^{\mathrm{T}}=(\mathrm{BA})^{\mathrm{T}} \mathrm{A}^{\mathrm{T}}$ \[ =\mathrm{A}^{\mathrm{T}} \mathrm{B}^{\mathrm{T}} \mathrm{A}^{\mathrm{T}}=\mathrm{A}(\mathrm{BA}) \rightarrow \text { symmetric } \]
$((\mathrm{AB}) \mathrm{A}))^{\mathrm{T}}=\mathrm{A}^{\mathrm{T}} \mathrm{B}^{\mathrm{T}} \mathrm{A}^{\mathrm{T}}=(\mathrm{AB}) \mathrm{A} \rightarrow$ symmetric
Statement - 1 is true
Statement- 2:
$(\mathrm{AB})^{\mathrm{T}}=\mathrm{B}^{\mathrm{T}} \mathrm{A}^{\mathrm{T}}=\mathrm{B} \mathrm{A}$
if $\mathrm{AB}=\mathrm{B} \mathrm{A}$ then
$(\mathrm{AB})^{\mathrm{T}}=\mathrm{B} \mathrm{A}=\mathrm{AB}$
Statement- 2 is true
but Not a correct expalnation.
Q. Statement-1 : Determinant of a skew-symmetric matrix of order 3 is zero.
Statement-1 : For any matrix A, det(AT) = det(A) and det(–A) = –det(A).
Where det(B) denotes the determinant of matrix B. Then :
(1) Statement-1 is true and statement-2 is false
(2) Both statements are true
(3) Both statements are false
(4) Statement-1 is false and statement-2 is true.
[AIEEE-2011]
Ans. (1)
Statement- 1: The value of determinant of skew symmetric matrix of odd order is always zero. So Statement-I. is true. Statement-II : This Statement is not always true depends on the order of matrix. $|-A|=-|A|$ if order is odd, so Statement--II is wrong. Statement-I is true and Statement-II is false.
Q. Let $\mathrm{A}=\left(\begin{array}{lll}{1} & {0} & {0} \\ {2} & {1} & {0} \\ {3} & {2} & {1}\end{array}\right) .$ If $\mathrm{u}_{1}$ and $\mathrm{u}_{2}$ are column matrices such that $\mathrm{Au}_{1}=\left(\begin{array}{l}{1} \\ {0} \\ {0}\end{array}\right)$ and $\mathrm{Au}_{2}=\left(\begin{array}{l}{0} \\ {1} \\ {0}\end{array}\right),$ then $\mathrm{u}_{1}+\mathrm{u}_{2}$ is equal to :
[AIEEE-2012]
[AIEEE-2012]
Ans. (1)
Q. If $P=\left[\begin{array}{lll}{1} & {\alpha} & {3} \\ {1} & {3} & {3} \\ {2} & {4} & {4}\end{array}\right]$ is the adjoint of a $3 \times 3$ matrix $A$ and $|A|=4,$ then $\alpha$ is equal to
(1) 4 (2) 11 (3) 5 (4) 0
[JEE(Main) - 2013]
Ans. (2)
P = adj (A)
taking determinant
Q. If $\mathrm{A}$ is an $3 \times 3 \times 3$ non-singular matrix such that $\mathrm{AA}^{\prime}=\mathrm{A}^{\prime} \mathrm{A}$ and $\mathrm{B}=\mathrm{A}^{-1} \mathrm{A}^{\prime},$ the BB' equals :
(1) I + B (2) I (3) $\mathrm{B}^{-1}$ (4) $\left(B^{-1}\right)^{\prime}$
[JEE(Main) - 2014]
Ans. (2)
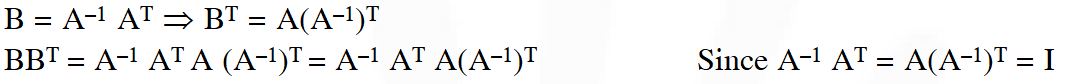
Q. If $A=\left[\begin{array}{ccc}{1} & {2} & {2} \\ {2} & {1} & {-2} \\ {a} & {2} & {b}\end{array}\right]$ is a matrix satisfying the equation $A A^{T}=9$, where I is $3 \times 3$ identity matrix, then the ordered pair $(\mathrm{a}, \mathrm{b})$ is equal to :
(1) (2, 1) (2) (–2, –1) (3) (2, –1) (4) (–2, 1)
[JEE(Main)-2015]
Ans. (2)
Q. If $\mathrm{A}=\left[\begin{array}{cc}{5 \mathrm{a}} & {-\mathrm{b}} \\ {3} & {2}\end{array}\right]$ and $\mathrm{A}$ adj $\mathrm{A}=\mathrm{A} \mathrm{A}^{\mathrm{T}},$ then $5 \mathrm{a}+\mathrm{b}$ is equal to :
(1) 13 (2) –1 (3) 5 (4) 4
[JEE(Main)-2016]
Ans. (3)
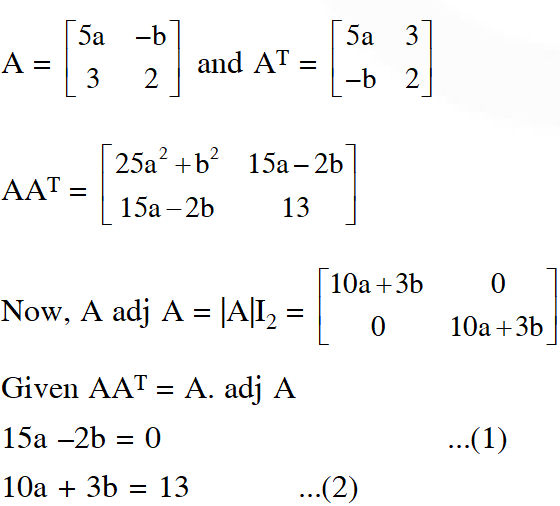
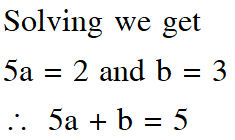
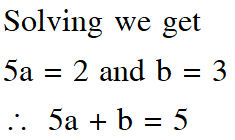
Q. If $A=\left[\begin{array}{cc}{2} & {-3} \\ {-4} & {1}\end{array}\right],$ then adj $\left(3 A^{2}+12 A\right)$ is equal to :-
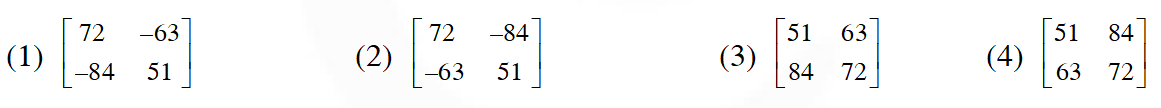[JEE(Main)-2017]
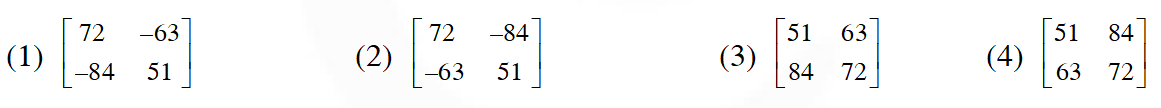[JEE(Main)-2017]
Ans. (3)
Q. Let $\mathrm{A}=\left[\begin{array}{lll}{1} & {0} & {0} \\ {1} & {1} & {0} \\ {1} & {1} & {1}\end{array}\right]$ and $\mathrm{B}=\mathrm{A}^{20} .$ Then the sum of the elements of the first column of $\mathrm{B}$ is :
(1) 211 (2) 251 (3) 231 (4) 210
[JEE(Main)-2018]
Ans. (3)
Q. Let A be a matrix such that A. $\left[\begin{array}{ll}{1} & {2} \\ {0} & {3}\end{array}\right]$ is a scalar matrix and $|3 \mathrm{A}|=108 .$ Then $\mathrm{A}^{2}$ equals :
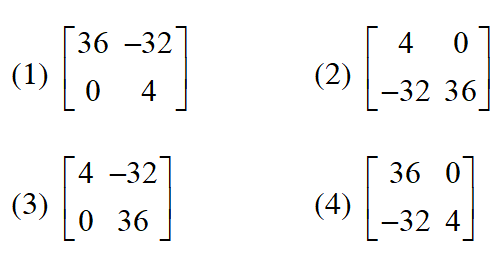[JEE(Main)-2018]
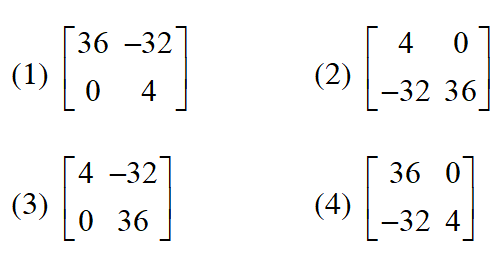[JEE(Main)-2018]
Ans. (1)
Q. Suppose $\mathrm{A}$ is any $3 \times 3$ non-singular matrix and $(\mathrm{A}-3 \mathrm{I})(\mathrm{A}-5 \mathrm{I})=0,$ where $\mathrm{I}=\mathrm{I}_{3}$ and $\mathrm{O}$ $=\mathrm{O}_{3} .$ If $\alpha \mathrm{A}+\beta \mathrm{A}^{-1}=4 \mathrm{I},$ then $\alpha+\beta$ is equal to :
(1) 13 (2) 7 (3) 12 (4) 8
[JEE(Main)-2018]
Ans. (4)
Comments
Aditya sonwani
June 3, 2024, 6:35 a.m.
Sir(prateek sir or saransh sir)plz recheck the error and fixed it show student are abusing in the comment section
E saral Lund
April 16, 2022, 12:52 a.m.
Abbey bsdk JEE ADV ke question ki jagah mains ke de raha hai lodu E SARAL
esaral jhatu
Sept. 10, 2021, 7:23 p.m.
Terii maa ki chut E-saral, bhosdi ke ye jee main ke question hain na ki advanced, lund e-saral gandu
mr, non iitian
Sept. 8, 2021, 10:53 a.m.
ye sare milke humko pagal bana rahe hain madhachod ke bachhe..............
Ninganagoud ayyappagol
Oct. 15, 2020, 10:32 a.m.
I need jee advance questions but hear is jee main quetuons
Munna
Sept. 21, 2020, 7:47 a.m.
Are bevkuf Insan JEE advanced ke question paper chahie Jee main kah de rakha hai yahan par.
Dj
Sept. 19, 2020, 7:49 p.m.
Jee mains ke question de rekhe h aur jee advance ka tag lga rakh h. WoooooooW!
Sanjay
Sept. 7, 2020, 7:07 p.m.
Here jee mains -questions...title is jee advanced..please correct it