JEE Advanced Previous Year Questions of Math with Solutions are available at eSaral. Practicing JEE Advanced Previous Year Papers Questions of mathematics will help the JEE aspirants in realizing the question pattern as well as help in analyzing weak & strong areas.
eSaral helps the students in clearing and understanding each topic in a better
Let $f:[0,1] \rightarrow \mathbb{R}$ (the set of all real numbers) be a function. Suppose the function $f$ is twice differentiable, $f(0)=f(1)=0$ and satisfies $f^{\prime \prime}(\mathrm{x})-2 f^{\prime}(\mathrm{x})+f(\mathrm{x}) \geq \mathrm{e}^{\mathrm{x}}, \mathrm{x} \in[0,1]$b
way. eSaral also provides complete chapter-wise notes of Class 11th and 12th both for all subjects.
Besides this, eSaral also offers NCERT Solutions, Previous year questions for JEE Main and Advance, Practice questions, Test Series for JEE Main, JEE Advanced and NEET, Important questions of Physics, Chemistry, Math, and Biology and many more.
Download eSaral app for free study material and video tutorials.
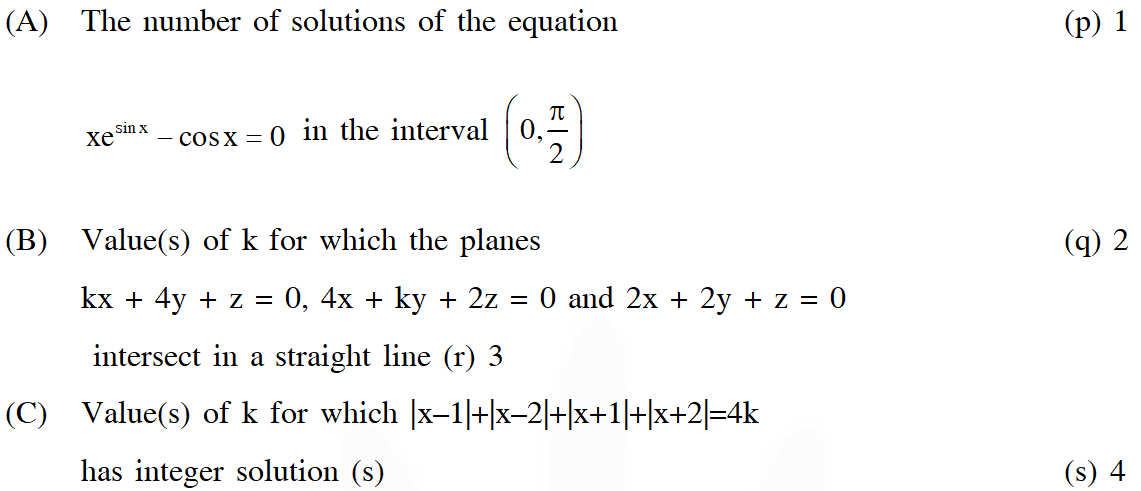
Q. Match the statements/expressions in Column-I with the open intervals in Column-II.
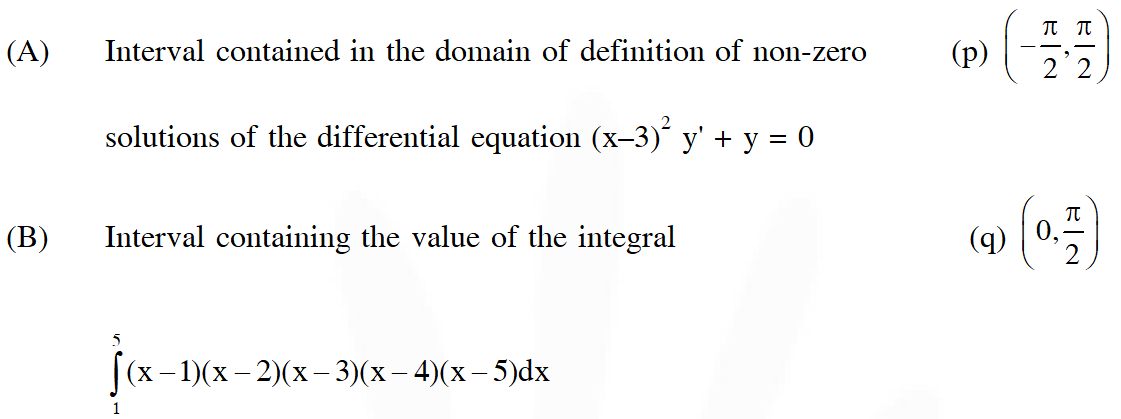
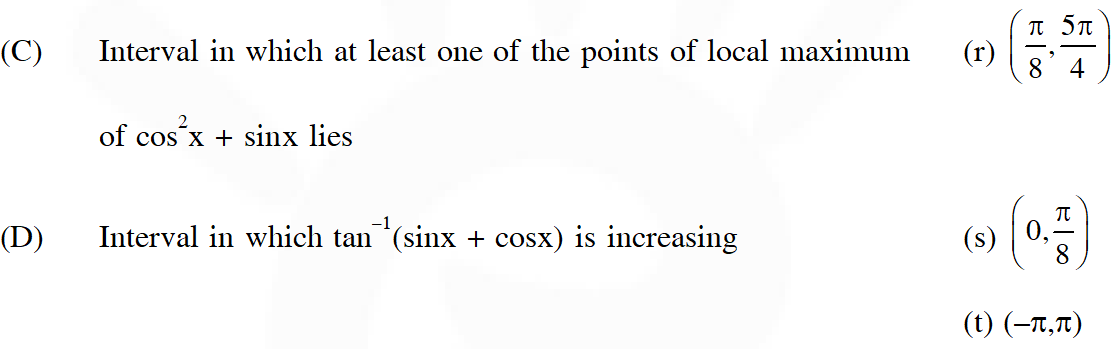

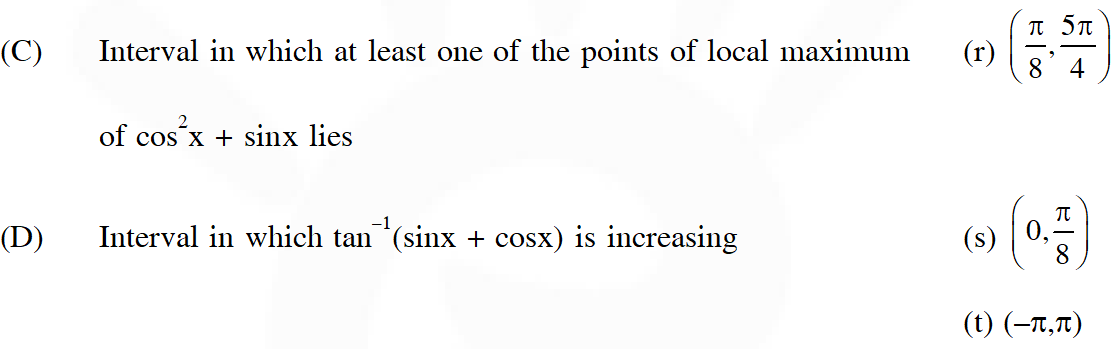
Ans. ( (A) p,q,s (B) p,t (C) p,q,r,t (D) S)
(i) $(\mathrm{A}) \frac{\mathrm{dy}}{\mathrm{dx}}=-\frac{\mathrm{y}}{(\mathrm{x}-3)^{2}}$ $\Rightarrow \ln y=\frac{1}{x-3}+c$ $\Rightarrow \mathrm{y}=\frac{1}{\mathrm{e}^{x-3}}, \mathrm{x} \neq 3$
(B) $\mathrm{I}=\int_{1}^{5}(\mathrm{x}-1)(\mathrm{x}-2)(\mathrm{x}-3)(\mathrm{x} 4)(\mathrm{x}-5) \mathrm{d} \mathrm{x}$
Applying king $\mathrm{x} \rightarrow 6-\mathrm{x}$ $\mathrm{I}=\int_{1}^{5}(5-\mathrm{x})(4-\mathrm{x})(3-\mathrm{x})(2-\mathrm{x})(1-\mathrm{x}) \mathrm{d} \mathrm{x}=-\mathrm{I}$ $\Rightarrow \mathrm{I}=0$
(C) $f(x)=\cos ^{2} x+\sin x$ $f^{\prime}(x)=-2 \cos x \sin x+\cos x$ $\Rightarrow \cos x(-2 \sin x+1)=0$ $\cos x=0$ or $\sin x=\frac{1}{2}$
sign of $f^{\prime}(\mathrm{x})$ changes from -ve to +ve while $f(\mathrm{x})$ passes through $\mathrm{x}=\frac{\pi}{6}, \frac{5 \pi}{6}$
(D) $f(\mathrm{x})=\tan ^{-1}(\sin \mathrm{x}+\cos \mathrm{x})$ $f(x)=\frac{\cos x-\sin x}{1+(\sin x+\cos )^{2}}>0$
 $\mathrm{x} \in(-3 \pi / 4, \pi / 4)$
(ii) $(\mathrm{A}) f(\mathrm{x})=\mathrm{xe}^{\sin \mathrm{x}}-\cos \mathrm{x}$
$f(0)=-1$
$f(\pi / 2)=\frac{\pi}{2} \mathrm{e}$
$f^{\prime}(x)=x e^{\sin x} \cos x+e^{\sin x}>0$
$\mathrm{x} \in(-3 \pi / 4, \pi / 4)$
(ii) $(\mathrm{A}) f(\mathrm{x})=\mathrm{xe}^{\sin \mathrm{x}}-\cos \mathrm{x}$
$f(0)=-1$
$f(\pi / 2)=\frac{\pi}{2} \mathrm{e}$
$f^{\prime}(x)=x e^{\sin x} \cos x+e^{\sin x}>0$
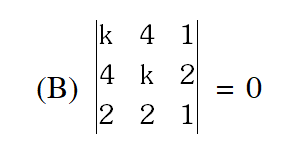 $\Rightarrow k(k-4)-4 c+8-2 k=0$
$\Rightarrow \mathrm{k}^{2}-4 \mathrm{k}+8-2 \mathrm{k}=0$
$\Rightarrow \mathrm{k}^{2}-6 \mathrm{k}+8=0$
$\Rightarrow \mathrm{k}=2,4$
(C) $|x-1|+|x-2|+|x+1|+|x+2|=4 k$
$4 k=8,12,16,20$$\quad\left\{\begin{array}{l}{\text { modulus denotes the }} \\ {\text { distance of from }} \\ {-2,-1,2}\end{array}\right.$
$\therefore \mathrm{k}=2,3,4,5$
(D) $\frac{d y}{y+1}=d x$
$\ln (\mathrm{y}+1)=\mathrm{ke}^{\mathrm{x}}$
$y+1=k e^{x}$
$y+1=2=k$
$y+1=2 e^{x}$
$y=\left(2 e^{x}-1\right)$
$y(\ln 2)=3$
$\Rightarrow k(k-4)-4 c+8-2 k=0$
$\Rightarrow \mathrm{k}^{2}-4 \mathrm{k}+8-2 \mathrm{k}=0$
$\Rightarrow \mathrm{k}^{2}-6 \mathrm{k}+8=0$
$\Rightarrow \mathrm{k}=2,4$
(C) $|x-1|+|x-2|+|x+1|+|x+2|=4 k$
$4 k=8,12,16,20$$\quad\left\{\begin{array}{l}{\text { modulus denotes the }} \\ {\text { distance of from }} \\ {-2,-1,2}\end{array}\right.$
$\therefore \mathrm{k}=2,3,4,5$
(D) $\frac{d y}{y+1}=d x$
$\ln (\mathrm{y}+1)=\mathrm{ke}^{\mathrm{x}}$
$y+1=k e^{x}$
$y+1=2=k$
$y+1=2 e^{x}$
$y=\left(2 e^{x}-1\right)$
$y(\ln 2)=3$
 $\mathrm{x} \in(-3 \pi / 4, \pi / 4)$
(ii) $(\mathrm{A}) f(\mathrm{x})=\mathrm{xe}^{\sin \mathrm{x}}-\cos \mathrm{x}$
$f(0)=-1$
$f(\pi / 2)=\frac{\pi}{2} \mathrm{e}$
$f^{\prime}(x)=x e^{\sin x} \cos x+e^{\sin x}>0$
$\mathrm{x} \in(-3 \pi / 4, \pi / 4)$
(ii) $(\mathrm{A}) f(\mathrm{x})=\mathrm{xe}^{\sin \mathrm{x}}-\cos \mathrm{x}$
$f(0)=-1$
$f(\pi / 2)=\frac{\pi}{2} \mathrm{e}$
$f^{\prime}(x)=x e^{\sin x} \cos x+e^{\sin x}>0$
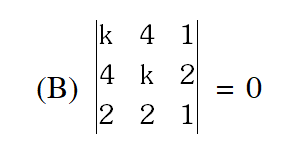 $\Rightarrow k(k-4)-4 c+8-2 k=0$
$\Rightarrow \mathrm{k}^{2}-4 \mathrm{k}+8-2 \mathrm{k}=0$
$\Rightarrow \mathrm{k}^{2}-6 \mathrm{k}+8=0$
$\Rightarrow \mathrm{k}=2,4$
(C) $|x-1|+|x-2|+|x+1|+|x+2|=4 k$
$4 k=8,12,16,20$$\quad\left\{\begin{array}{l}{\text { modulus denotes the }} \\ {\text { distance of from }} \\ {-2,-1,2}\end{array}\right.$
$\therefore \mathrm{k}=2,3,4,5$
(D) $\frac{d y}{y+1}=d x$
$\ln (\mathrm{y}+1)=\mathrm{ke}^{\mathrm{x}}$
$y+1=k e^{x}$
$y+1=2=k$
$y+1=2 e^{x}$
$y=\left(2 e^{x}-1\right)$
$y(\ln 2)=3$
$\Rightarrow k(k-4)-4 c+8-2 k=0$
$\Rightarrow \mathrm{k}^{2}-4 \mathrm{k}+8-2 \mathrm{k}=0$
$\Rightarrow \mathrm{k}^{2}-6 \mathrm{k}+8=0$
$\Rightarrow \mathrm{k}=2,4$
(C) $|x-1|+|x-2|+|x+1|+|x+2|=4 k$
$4 k=8,12,16,20$$\quad\left\{\begin{array}{l}{\text { modulus denotes the }} \\ {\text { distance of from }} \\ {-2,-1,2}\end{array}\right.$
$\therefore \mathrm{k}=2,3,4,5$
(D) $\frac{d y}{y+1}=d x$
$\ln (\mathrm{y}+1)=\mathrm{ke}^{\mathrm{x}}$
$y+1=k e^{x}$
$y+1=2=k$
$y+1=2 e^{x}$
$y=\left(2 e^{x}-1\right)$
$y(\ln 2)=3$
Q. Match the statements/expressions in Column-I with the values given in Column-II.
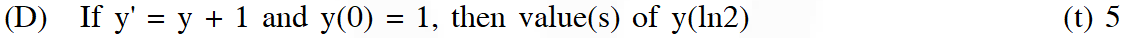 [JEE 2009, (2+2+2+2) × 2]
[JEE 2009, (2+2+2+2) × 2]

Ans. ( (A) P (B) q,s (C) q,r,s,t (D) r )
Given $\mathrm{y}=f(\mathrm{x})$
Tangent at point $\mathrm{P}(\mathrm{x}, \mathrm{y})$
$\mathrm{Y}-\mathrm{y}=\left(\frac{\mathrm{d} y}{\mathrm{dx}}\right)_{(\mathrm{x}, \mathrm{y})}(\mathrm{X}-\mathrm{x})$
Now y-intercept $\Rightarrow Y=y-x \frac{d y}{d x}$
Given that, $\quad \mathrm{y}-\mathrm{x} \frac{\mathrm{d} y}{\mathrm{dx}}=\mathrm{x}^{3}$
$\Rightarrow \frac{d y}{d x}-\frac{y}{x}=-x^{2}$ is a linear differential equation
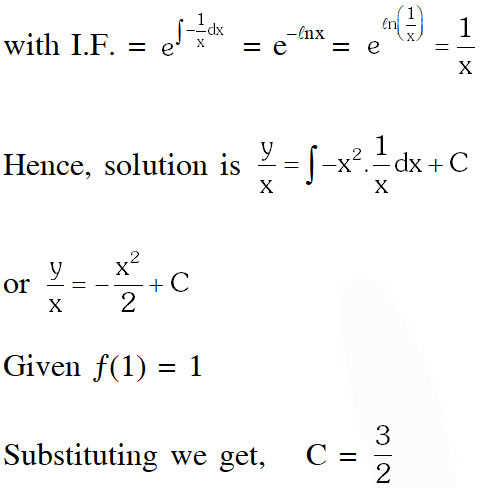
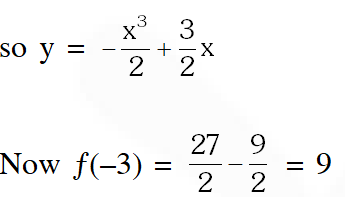
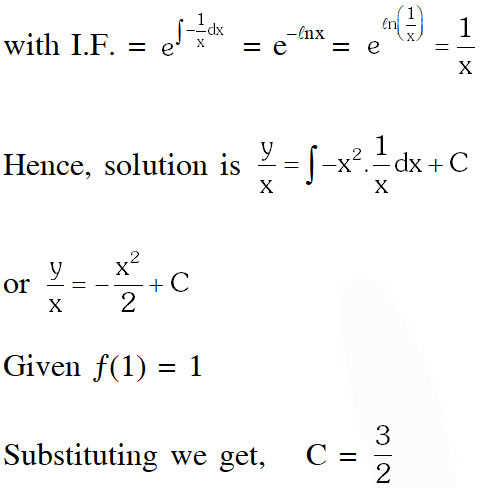
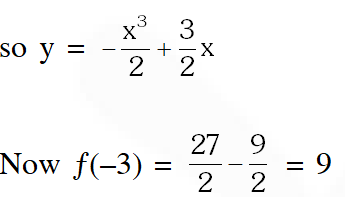
Q. Let f be a real valued differentiable function on R (the set of all real numbers) such that f(1) = 1. If the y-intercept of the tangent at any point P(x,y) on the curve y =f(x) is equal to the cube of the abscissa of P, then the value of f(–3) is equal to
[JEE 2010, 3]
Ans. 9


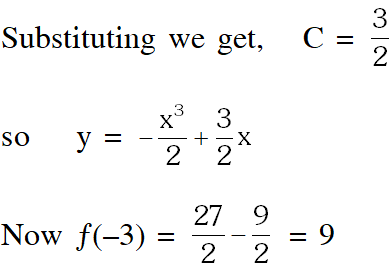

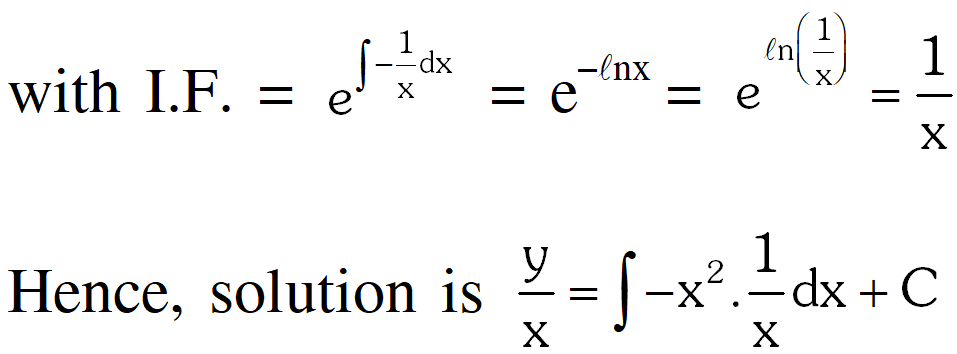

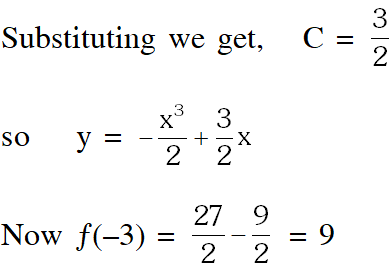
Q. (A) Let $f:[1, \infty) \rightarrow[2, \infty)$ be a differentiable function such that $f(1)=2 .$ If $6 \int_{1}^{x} f(t) d t=3 x f(x)-x^{3}$ for all $x \geq 1,$ then the value of $f(2)$ is
(B) Let $y^{\prime}(x)+y(x) g^{\prime}(x)=g(x) g^{\prime}(x), y(0)=0, x \in R,$ where $f^{\prime}(x)$ denotes $\frac{d f(x)}{d x}$ and $g(x)$ is a given non-constant differentiable function on $\mathrm{R}$ with $\mathrm{g}(0)=\mathrm{g}(2)=0 .$ Then the value of $\mathrm{y}(2)$ is
[JEE 2011, 4]
Ans. ( (A) Bonus (B) 0 )
(a) (Bonus)
(Comment : The given relation does not hold for x =1, therefore it is not an identity. Hence there is an error in given question. The correct identity must be-)
$6 \int_{1}^{x} f(t) d t=3 x f(x)-x^{3}-5, \forall x \geq 1$
Now applying Newton Leibnitz theorem
$6 f(x)=3 x f^{\prime}(x)-3 x^{2}+3 f(x)$
$\Rightarrow 3 f(x)=3 x f^{\prime}(x)-3 x^{2}$
Let $y=f(x)$
$\Rightarrow x \frac{d y}{d x}-y=x^{2}$
$\Rightarrow \frac{x d y-y d x}{x^{2}}=d x$
$\Rightarrow \quad \int d\left(\frac{y}{x}\right)=\int d x$
$\Rightarrow \quad \frac{y}{x}=x+C \quad$ (where $C$ is constant)
$\Rightarrow \quad y=x^{2}+C x$
$\therefore f(\mathrm{x})=\mathrm{x}^{2}+\mathrm{Cx}$
Given $f(1)=2$
$\Rightarrow \mathrm{C}=1$
$\therefore f(2)=2^{2}+2=6$
(B) Given $\mathrm{y}(0)=0, \mathrm{g}(0)=\mathrm{g}(2)=0$
\[
\begin{array}{l}
{\text { Let } y^{\prime}(x)+y(x) \cdot g^{\prime}(x)=g(x) g^{\prime}(x)} \\
{\Rightarrow y^{\prime}(x)+(y(x)-g(x)) g^{\prime}(x)=0} \\
{\Rightarrow \quad \frac{y^{\prime}(x)}{g^{\prime}(x)}+y(x)=g(x)} \end{array}
$\Rightarrow \quad \frac{d y(x)}{d g(x)}+y(x)=g(x)$\]
$\Rightarrow \quad$ I.F. $=e^{\int d(g(x))}=e^{g(x)}$
$\Rightarrow \quad y(x) \cdot e^{g(x)}=\int e^{g(x)} g(x) \cdot d g(x)$
$y(x) \cdot e^{g(x)}=g(x) \cdot e^{g(x)}-e^{g(x)}+c$
put $\mathrm{x}=0$
$\Rightarrow 0=0-1+c \Rightarrow c=1$
$\Rightarrow \mathrm{y}(2) \cdot \mathrm{e}^{\mathrm{g}(2)}=\mathrm{g}(2) \mathrm{e}^{\mathrm{g}(2)}-\mathrm{e}^{\mathrm{g}(2)}+1$
$\Rightarrow \mathrm{y}(2)=0-\mathrm{e}^{0}+1$
$\Rightarrow \quad \mathrm{y}(2)=0$
Q. If y(x) satisfies the differential equation y' – ytanx = 2x sec x and y(0) = 0, then
(A) $\mathrm{y}\left(\frac{\pi}{4}\right)=\frac{\pi^{2}}{8 \sqrt{2}}$
(B) $y^{\prime}\left(\frac{\pi}{4}\right)=\frac{\pi^{2}}{18}$
(C) $\mathrm{y}\left(\frac{\pi}{4}\right)=\frac{\pi^{2}}{9}$
(D) $\mathrm{y}^{\prime}\left(\frac{\pi}{3}\right)=\frac{4 \pi}{3}+\frac{2 \pi^{2}}{3 \sqrt{3}}$
[JEE 2012, 4M]
Ans. (A,D)
$\frac{\mathrm{dy}}{\mathrm{dx}}-y \tan \mathrm{x}=2 \mathrm{x} \sec \mathrm{x}$
$\mathrm{I.F.}=\mathrm{e}^{\int-\tan \mathrm{xdx}}=\cos \mathrm{x}$
$\therefore$ Equation reduces to
y. $\cos x=\int 2 x \cdot \sec x \cdot \cos x d x$
$\Rightarrow \mathrm{y} \cos \mathrm{x}=\mathrm{x}^{2}+\mathrm{C}$
$\therefore y \cos x=x^{2}$
$\Rightarrow \mathrm{y}(\mathrm{x})=\mathrm{x}^{2} \mathrm{secx}$
$\therefore y\left(\frac{\pi}{4}\right)=\frac{\pi^{2}}{16} \sqrt{2}=\frac{\pi^{2}}{8 \sqrt{2}}(\therefore(\mathrm{A}) \text { is correct })$
$y\left(\frac{\pi}{3}\right)=\frac{\pi^{2}}{9} \cdot 2=\frac{2 \pi^{2}}{9}(\therefore(\mathrm{C}) \text { is wrong })$
Also $\mathrm{y}^{\prime}(\mathrm{x})=2 \mathrm{x} \sec \mathrm{x}+\mathrm{x}^{2} \sec \mathrm{x} \tan \mathrm{x}$
$\Rightarrow y^{\prime}\left(\frac{\pi}{4}\right)=\frac{\pi}{2} \cdot \sqrt{2}+\frac{\pi^{2} \sqrt{2}}{16}(\therefore(\mathrm{B}) \text { is wrong })$
and $y^{\prime}\left(\frac{\pi}{3}\right)=2 \cdot \frac{\pi}{3} \cdot 2+\frac{\pi^{2}}{9} \cdot 2 \cdot \sqrt{3}$
$=\frac{4 \pi}{3}+\frac{2 \pi^{2}}{3 \sqrt{3}} \quad(\therefore(\mathrm{D}) \text { is correct })$
Q. Let $f:\left[\frac{1}{2}, 1\right] \rightarrow \mathrm{R}$ (the set of all real numbers) be a positive, non-constant and differentiable function such that $f^{\prime}(\mathrm{x})<2 f(\mathrm{x})$ and $f\left(\frac{1}{2}\right)=1 .$ Then the value of $\int_{1 / 2}^{1} f(\mathrm{x}) \mathrm{d} \mathrm{x}$ lies in the interval
(A) (2e – 1, 2e)
(B) (e – 1, 2e – 1)
(C) $\left(\frac{\mathrm{e}-1}{2}, \mathrm{e}-1\right)$
$(D)\left(0, \frac{e-1}{2}\right)$
[JEE(Advanced) 2013, 2M]
Ans. (D)
$f^{\prime}(x)-2 f(x)<0$
Multiply both side by e $^{-2 x}$
$e^{-2 x} f^{\prime}(x)-2 e^{-2 x} f(x)<0$
$\frac{d}{d x}\left(e^{-2 x} f(x)\right)<0$
$\mathrm{Now}, \mathrm{g}(\mathrm{x})=\mathrm{e}^{-2 \mathrm{x}} f(\mathrm{x})$
$g(x)$ is a decreasing function.
$x>\frac{1}{2}$
$g(x)
$\Rightarrow e^{-2 x} f(x)<\frac{1}{e}$
$\Rightarrow f(\mathrm{x})<\mathrm{e}^{2 \mathrm{x}-1}$
$\Rightarrow \int_{1 / 2}^{1} f(\mathrm{x}) \mathrm{d} \mathrm{x}<\frac{1}{\mathrm{e}} \int_{1 / 2}^{1} \mathrm{e}^{2 \mathrm{x}} \mathrm{d} \mathrm{x}$
$=\left[\frac{1}{2 e} e^{2 x}\right]_{1 / 2}^{1}=\frac{1}{2 e}\left(e^{2}-e\right)=\frac{1}{2}(e-1)$


Q. curve passes through the point $\left(1, \frac{\pi}{6}\right) .$ Let the slope of the curve at each point $(\mathrm{x}, \mathrm{y})$ be $\frac{y}{x}+\sec \left(\frac{y}{x}\right), x>0 .$ Then the equation of the curve is
(A) $\sin \left(\frac{y}{x}\right)=\log x+\frac{1}{2}$
(B) $\csc \left(\frac{y}{x}\right)=\log x+2$
(C) $\sec \left(\frac{2 \mathrm{y}}{\mathrm{x}}\right)=\log \mathrm{x}+2$
(D) $\cos \left(\frac{2 y}{x}\right)=\log x+\frac{1}{2}$
[JEE(Advanced) 2013, 2M]
Ans. (A)
$\frac{d y}{d x}=\frac{y}{x}+\sec \frac{y}{x}$
Let $y=v x$
$\frac{d y}{d x}=v+x \frac{d v}{d x}$
$\mathrm{v}+\frac{\mathrm{xdv}}{\mathrm{dx}}=\mathrm{v}+\sec \mathrm{v}$
$\cos v d v=\frac{d x}{x}$
$\sin \mathrm{v}=\ell \mathrm{nx}+\mathrm{c}$
$\sin \left(\frac{y}{x}\right)=\ell n x+c$
$\because$ passing through $\left(1, \frac{\pi}{6}\right)$
$\Rightarrow \sin \frac{\pi}{6}=c \Rightarrow c=\frac{1}{2}$
$\therefore \sin \frac{y}{x}=\ell n x+\frac{1}{2}$
Q. If the function $\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})$ assumes its minimum in the interval $[0,1]$ at $\mathrm{x}=\frac{1}{4},$ which of the following is true?
(A) $f^{\prime}(x)
(B) $f^{\prime}(x)>f(x), 0
(C) $f^{\prime}(x)
(D) $f^{\prime}(x)
[JEE(Advanced) 2013, 3, (–1)]
Ans. (C)
$\mathrm{e}^{-\mathrm{x}}\left(f^{\prime \prime}(\mathrm{x})-2 f^{\prime}(\mathrm{x})+f(\mathrm{x})\right) \geq 1$
$\mathrm{D}\left(\left(f^{\prime}(\mathrm{x})-f(\mathrm{x})\right) \mathrm{e}^{-\mathrm{x}}\right) \geq 1$
$\Rightarrow \mathrm{D}\left(\left(f^{\prime}(\mathrm{x})-f(\mathrm{x}) \mathrm{e}^{-\mathrm{x}}\right) \geq 0\right.$
$\Rightarrow\left(f^{\prime}(\mathrm{x})-f(\mathrm{x})\right) \mathrm{e}^{-\mathrm{x}}$ is an increasing function.
As we know that $\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})$ has local minima at $\mathrm{x}=\frac{1}{4}$
$\mathrm{e}^{-\mathrm{x}}\left(f^{\prime}(\mathrm{x})-f(\mathrm{x})\right)=0$ at $\mathrm{x}=\frac{1}{4}$
Let $\mathrm{F}(\mathrm{x})=\mathrm{e}^{-\mathrm{x}}\left(f^{\prime}(\mathrm{x})-f(\mathrm{x})\right)$
$\mathrm{F}(\mathrm{x})<0$ in $\left(0, \frac{1}{4}\right)$
$\mathrm{e}^{-\mathrm{x}}\left(f^{\prime}(\mathrm{x})-f(\mathrm{x})<0 \text { in }\left(0, \frac{1}{4}\right)\right.$
$f^{\prime}(x)
option $\mathrm{C}$
Q. Which of the following is true for 0 < x < 1 ?
(A) $0
(B) $-\frac{1}{2}
(C) $-\frac{1}{4}
(D) $-\infty
[JEE(Advanced) 2013, 3, (–1)]
Ans. (D)
$\mathrm{D}\left(\mathrm{e}^{-\mathrm{x}}\left(f^{\prime}(\mathrm{x})-f(\mathrm{x})\right) \geq 0 \quad \forall \mathrm{x} \in(0,1)\right.$
$\mathrm{D}\left(\mathrm{D}\left(\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})\right) \geq 0 \forall \mathrm{x} \in(0,1)\right.$
$\mathrm{D}^{2}\left(\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})\right) \geq 0$
Let $\mathrm{F}(\mathrm{x})=\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})$
$\mathrm{F}^{\prime \prime}(\mathrm{x})>0$ means it is concave upward.
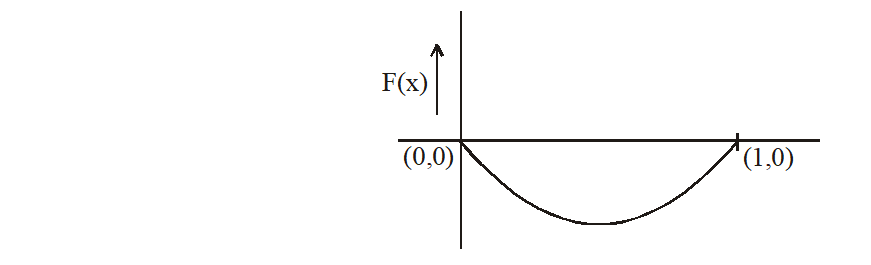 $\mathrm{F}(0)=\mathrm{F}(1)=0$
$\mathrm{F}(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$f(x)<0$
Option D is possible
$\mathrm{F}(0)=\mathrm{F}(1)=0$
$\mathrm{F}(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$f(x)<0$
Option D is possible
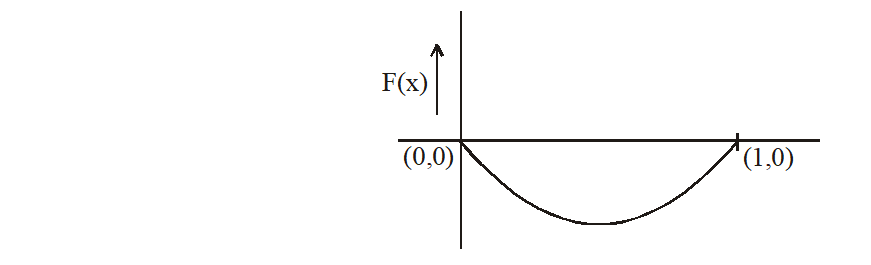 $\mathrm{F}(0)=\mathrm{F}(1)=0$
$\mathrm{F}(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$f(x)<0$
Option D is possible
$\mathrm{F}(0)=\mathrm{F}(1)=0$
$\mathrm{F}(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$\mathrm{e}^{-\mathrm{x}} f(\mathrm{x})<0 \forall \mathrm{x} \in(0,1)$
$f(x)<0$
Option D is possible
Q. The function y = ƒ(x) is the solution of the differential equation \frac{d y}{d x}+\frac{x y}{x^{2}-1}=\frac{x^{4}+2 x}{\sqrt{1-x^{2}}} in (–1, 1) satisfying ƒ(0) = 0. Then \int_{-\frac{\sqrt{3}}{2}}^{\frac{\sqrt{3}}{2}} f(\mathrm{x}) \mathrm{d} \mathrm{x} \text { is }
(A) $\frac{\pi}{3}-\frac{\sqrt{3}}{2}$
(B) $\frac{\pi}{3}-\frac{\sqrt{3}}{4}$
(C) $\frac{\pi}{6}-\frac{\sqrt{3}}{4}$
(D) $\frac{\pi}{6}-\frac{\sqrt{3}}{2}$
[JEE(Advanced)-2014, 3(–1)]
Ans. (B)
$\left(\frac{d y}{d x}-\frac{x y}{\left(1-x^{2}\right)}\right) \sqrt{1-x^{2}}=x^{4}+2 x$
$\Rightarrow \sqrt{1-x^{2}} d y+d(\sqrt{1-x^{2}}) y=\left(x^{4}+2 x\right) d x$
$\Rightarrow y \sqrt{1-x^{2}}=\frac{x^{5}}{5}+x^{2}+c$
by $(0,0) \mathrm{c}=0$
$y=\frac{x^{5}}{5 \sqrt{1-x^{2}}}+\frac{x^{2}}{\sqrt{1-x^{2}}}$
$=\int_{-\sqrt{3} / 2}^{\sqrt{3} / 2}\left(\frac{x^{5}}{5 \sqrt{1-x^{2}}}+\frac{x^{2}}{\sqrt{1-x^{2}}}\right) d x$
$=2 \int_{0}^{\sqrt{3} / 2} \frac{x^{2}}{\sqrt{1-x^{2}}} d x$ put $x=\sin \theta$
$=2 \int_{0}^{\pi / 3} \sin ^{2} \theta \mathrm{d} \theta=\int_{0}^{\pi / 3}(1-\cos 2 \theta) \mathrm{d} \theta$
$=\left(\theta-\frac{\sin 2 \theta}{2}\right)_{0}^{\pi / 3}=\frac{\pi}{3}-\frac{\sqrt{3}}{4}$
Q. Let $\mathrm{y}(\mathrm{x})$ be a solution of the differential equation $\left(1+\mathrm{e}^{\mathrm{x}}\right) \mathrm{y}^{\prime}+\mathrm{ye}^{\mathrm{x}}=1 .$ If $\mathrm{y}(0)=2,$ then which of the following statements is(are) true ?
(A) y(–4) = 0
(B) y(–2) = 0
(C) y(x) has a critical point in the interval (–1,0)
(D) y(x) has no critical point in the interval (–1,0)
[JEE 2015, 4M, –2M]
Ans. (A,C)
$y^{\prime}+e^{x} y^{\prime}+y e^{x}=1$
$\Rightarrow \mathrm{dy}+\mathrm{d}\left(\mathrm{e}^{\mathrm{x}} \mathrm{y}\right)=\mathrm{d} \mathrm{x}$
$\Rightarrow \mathrm{y}+\mathrm{e}^{\mathrm{x}} \mathrm{y}=\mathrm{x}+\mathrm{c}$
$\because \mathrm{y}(0)=2$
$\Rightarrow \mathrm{c}=4$
$\Rightarrow y=\frac{x+4}{1+e^{x}}$
$\therefore \mathrm{y}(-4)=0$
for critical point given
$\frac{d y}{d x}=\frac{1-y e^{x}}{1+e^{x}}=\frac{1-\left(\frac{x+4}{1+e^{x}}\right) e^{x}}{1+e^{x}}=\frac{1-(x+3) e^{x}}{\left(1+e^{x}\right)^{2}}$
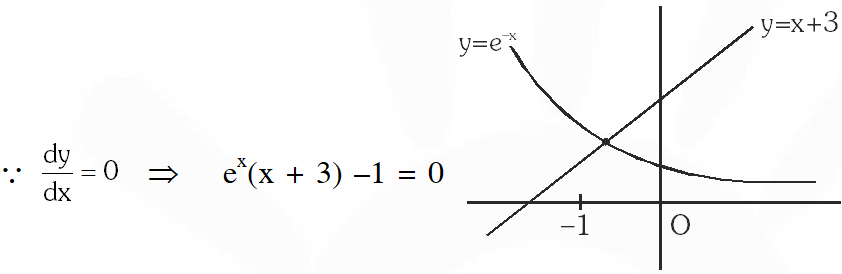 $\Rightarrow \quad x+3=e^{-x}$
$\mathrm{y}(\mathrm{x})$ has a critical point in the interval $(-1,0)$
$\Rightarrow \quad x+3=e^{-x}$
$\mathrm{y}(\mathrm{x})$ has a critical point in the interval $(-1,0)$
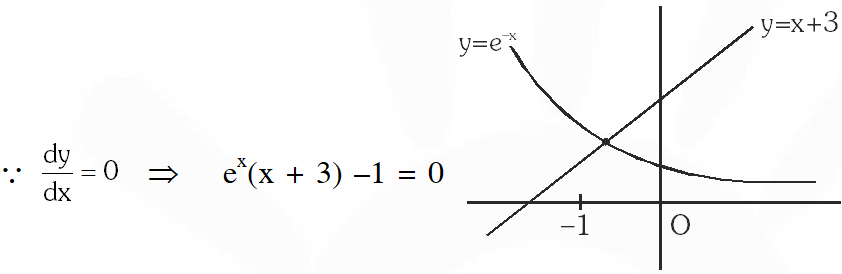 $\Rightarrow \quad x+3=e^{-x}$
$\mathrm{y}(\mathrm{x})$ has a critical point in the interval $(-1,0)$
$\Rightarrow \quad x+3=e^{-x}$
$\mathrm{y}(\mathrm{x})$ has a critical point in the interval $(-1,0)$
Q. Consider the family of all circles whose centers lie on the straight line y = x. If this family of circles is represented by the differential equation Py" + Qy' + 1 =
0, where P,Q are functions of x,y and y' (here $y^{\prime}=\frac{d y}{d x}, y^{\prime \prime}=\frac{d^{2} y}{d x^{2}},$ then which of the following statements is (are) true?
(A) P = y + x
(B) P = y – x
(C) $\mathrm{P}+\mathrm{Q}=1-\mathrm{x}+\mathrm{y}+\mathrm{y}^{\prime}+\left(\mathrm{y}^{\prime}\right)^{2}$
(D) P – Q = x + y – y' – $\left(y^{\prime}\right)^{2}$
[JEE 2015, 4M, –2M]
Ans. (B,C)
Let Circle $\mathrm{x}^{2}+\mathrm{y}^{2}-2 \mathrm{ax}-2 \mathrm{ay}+\mathrm{c}=0$
On differentiation
$2 \mathrm{x}+2 \mathrm{yy}^{\prime}-2 \mathrm{a}-2 \mathrm{ay}^{\prime}=0$
$\Rightarrow \mathrm{x}+\mathrm{yy}^{\prime}-\mathrm{a}\left(1+\mathrm{y}^{\prime}\right)=0$
$\Rightarrow a=\frac{x+y y^{\prime}}{1+y^{\prime}}$
again differentiation
$\frac{\left(1+(y)^{2}+y y^{\prime \prime}(1+y)-(x+y y)\left(y^{\prime \prime}\right)\right.}{(1+y)^{2}}=0$
$\Rightarrow 1+\mathrm{y}^{\prime}\left(\left(\mathrm{y}^{\prime}\right)^{2}+\mathrm{y}^{\prime}+1\right)+\mathrm{y}^{\prime \prime}(\mathrm{y}-\mathrm{x})=0$
$\therefore P=y-x$
$\mathrm{Q}=1+\mathrm{y}^{\prime}+\left(\mathrm{y}^{\prime}\right)^{2}$
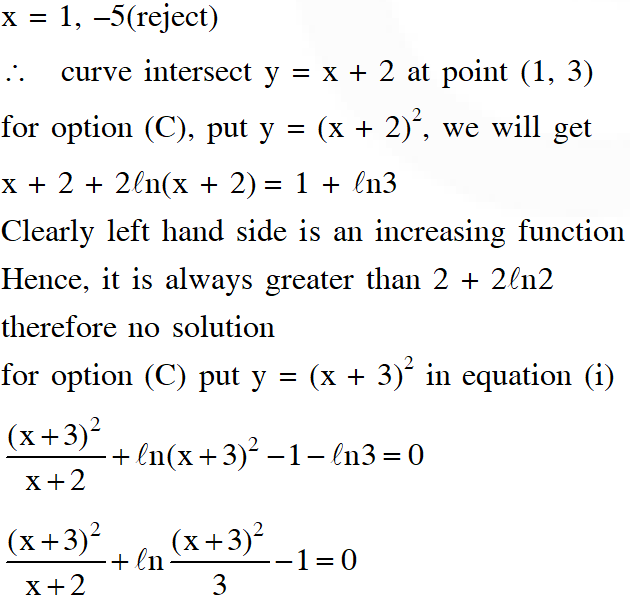
Q. A solution curve of the differential equation $\left(\mathrm{x}^{2}+\mathrm{xy}+4 \mathrm{x}+2 \mathrm{y}+4\right) \frac{\mathrm{dy}}{\mathrm{dx}}-\mathrm{y}^{2}=0, \mathrm{x}>0$ passes through the point $(1,3) .$ The the solution curve-
(A) intersects y = x + 2 exactly at one point
(B) intersects y = x + 2 exactly at two points
(C) intersects $y=(x+2)^{2}$
(D) does NOT intersect $y=(x+3)^{2}$
[JEE(Advanced)-2016]
Ans. (A,D)
$\left(\mathrm{x}^{2}+\mathrm{xy}+4 \mathrm{x}+2 \mathrm{y}+4\right) \frac{\mathrm{dy}}{\mathrm{dx}}-\mathrm{y}^{2}=0$
$\left((x+2)^{2}+y(x+2)\right) \frac{d y}{d x}=y^{2}$
Let $\mathrm{x}+2=\mathrm{X}, \mathrm{y}=\mathrm{Y}$
$(\mathrm{X})(\mathrm{X}+\mathrm{Y}) \frac{\mathrm{d} \mathrm{Y}}{\mathrm{dX}}=\mathrm{Y}^{2}$
$-\mathrm{X}^{2} \mathrm{d} \mathrm{Y}=\mathrm{X} \mathrm{YdY}-\mathrm{Y}^{2} \mathrm{d} \mathrm{X}$
$-\mathrm{X}^{2} \mathrm{d} \mathrm{Y}=\mathrm{Y}(\mathrm{XdY}-\mathrm{YdX})$
$-\frac{\mathrm{d} \mathrm{Y}}{\mathrm{Y}}=\frac{\mathrm{XdY}-\mathrm{YdX}}{\mathrm{X}^{2}}$

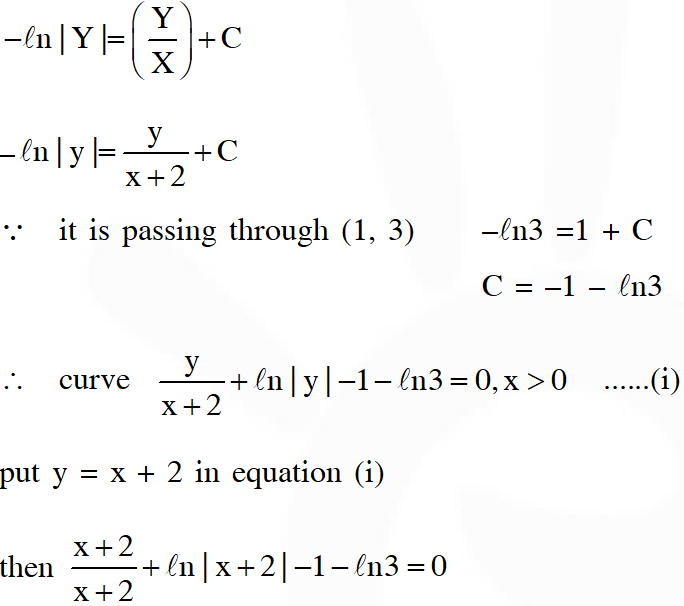


Q. Let $f:(0, \infty) \rightarrow \mathrm{R}$ be a differentiable function such that $f^{\prime}(\mathrm{x})=2-\frac{f(\mathrm{x})}{\mathrm{x}}$ for all $\mathrm{x} \in$ $(0, \infty)$ and $f(1) \neq 1 .$ Then
(A) $\lim _{x \rightarrow 0^{+}} f^{\prime}\left(\frac{1}{x}\right)=1$
(B) $\lim _{x \rightarrow 0^{+}} x f\left(\frac{1}{x}\right)=2$
(C) $\lim _{x \rightarrow 0^{+}} x^{2} f^{\prime}(x)=0$
(D) $|f(x)| \leq 2$ for all $x \in(0,2)$
[JEE(Advanced)-2016]
Ans. (A)
Let $\mathrm{y}=f(\mathrm{x})$
$\frac{d y}{d x}+\frac{y}{x}=2$ (linear differential equation)
$\therefore \mathrm{y.e}^{\int \frac{\mathrm{dx}}{\mathrm{x}}}=2 \int \mathrm{e}^{\int \frac{\mathrm{dx}}{\mathrm{x}}=2 \int \mathrm{e}^{\int \frac{\mathrm{dx}}{\mathrm{x}}} \mathrm{dx}+\mathrm{c}}$
$\Rightarrow \mathrm{yx}=2 \int \mathrm{xdx}+\mathrm{c}$
$\therefore \mathrm{yx}=\mathrm{x}^{2}+\mathrm{c}$
$\Rightarrow f(x)=x+\frac{c}{x} ;$ As $f(1) \neq 1 \Rightarrow c \neq 0$
$\Rightarrow f^{\prime}(x)=1-\frac{c}{x^{2}}, c \neq 0$
(A) $\lim _{x \rightarrow 0^{+}} f^{\prime}\left(\frac{1}{x}\right)=\lim _{x \rightarrow 0^{+}}\left(1-c x^{2}\right)=1$
(B) $\lim _{x \rightarrow 0^{+}} x f\left(\frac{1}{x}\right)=\lim _{x \rightarrow 0^{+}} x\left(\frac{1}{x}+c x\right)=\lim _{x \rightarrow 0^{+}}\left(1+c x^{2}\right)=1$
(C) $\lim _{x \rightarrow 0^{+}} x^{2} f^{\prime}(x)=\lim _{x \rightarrow 0^{+}} x^{2}\left(1-\frac{c}{x^{2}}\right)=\lim _{x \rightarrow 0^{+}}\left(x^{2}-c\right)=-c$
(D) $f(x)=x+\frac{c}{x}, c \neq 0$
for $\mathrm{c}>0$
$\therefore \lim _{x \rightarrow 0^{+}} f(x)=\infty \Rightarrow$ function is not bounded in $(0,2)$
Q. If y = y(x) satisfies the differential equation
$8 \sqrt{x}(\sqrt{9+\sqrt{x}}) d y=(\sqrt{4+\sqrt{9+\sqrt{x}}})^{-1} d x, \quad x>0$ and $y(0)=\sqrt{7},$ then $y(256)=$
(A) 80 (B) 3 (C) 16 (D) 9
[JEE(Advanced)-2016]
Ans. (B)
$y=\frac{1}{8} \int \frac{d x}{\sqrt{4+\sqrt{9+x} \cdot \sqrt{x} \cdot \sqrt{9+\sqrt{x}}}}$
put $\sqrt{9+\sqrt{\mathrm{x}}}=\mathrm{t}$
$\Rightarrow \frac{d x}{\sqrt{x} \cdot \sqrt{9+\sqrt{x}}}=4 d t$
$\therefore \mathrm{y}=\frac{4}{8} \int \frac{\mathrm{dt}}{\sqrt{4+\mathrm{t}}}$
$\Rightarrow \quad y=\sqrt{4+t}+C$
$\Rightarrow \mathrm{y}(\mathrm{x})=\sqrt{4+\sqrt{9+\sqrt{\mathrm{x}}}}+\mathrm{C}$
at $x=0: y(0)=\sqrt{7}$
$\Rightarrow \mathrm{C}=0$
$\therefore \mathrm{y}(\mathrm{x})=\sqrt{4+\sqrt{9+\sqrt{\mathrm{x}}}}$
$\Rightarrow \mathrm{y}(256)=3$
Q. If $f: \square \rightarrow \square$ is a differentiable function such that $f^{\prime}(\mathrm{x})>2 f(\mathrm{x})$ for all $\mathrm{x} \in \mathbb{U},$ and $f(0)$ $=1,$ then
(A) $f(\mathrm{x})>\mathrm{e}^{2 \mathrm{x}}$ in $(0, \infty)$
(B) $f(x)$ is decreasing in $(0, \infty)$
(C) $f(x)$ is increasing in $(0, \infty)$
(D) $f^{\prime}(x)
[JEE(Advanced)-2017]
Ans. (A,C)
Given that,
$\mathrm{f}^{\prime}(\mathrm{x})>2 \mathrm{f}(\mathrm{x}) \forall \mathrm{x} \in \mathrm{R}$
$\Rightarrow \mathrm{f}^{\prime}(\mathrm{x})-2 \mathrm{f}(\mathrm{x})>0$
$\forall \mathrm{x} \in \mathrm{R}$
$\therefore \mathrm{e}^{-2 \mathrm{x}}\left(\mathrm{f}^{\prime}(\mathrm{x})-2 \mathrm{f}(\mathrm{x})\right)>0 \forall \mathrm{x} \in \mathrm{R}$
$\Rightarrow \frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{e}^{-2 \mathrm{x}} \mathrm{f}(\mathrm{x})\right)>0 \forall \mathrm{x} \in \mathrm{R}$
Let $\mathrm{g}(\mathrm{x})=\mathrm{e}^{-2 \mathrm{x}} \mathrm{f}(\mathrm{x})$
Now, $g^{\prime}(x)>0 \forall x \in R$
$\Rightarrow \mathrm{g}(\mathrm{x})$ is strictly increasing $\forall \mathrm{x} \in \mathrm{R}$
Also, $\mathrm{g}(0)=1$
$\therefore \forall \mathrm{x}>0$
$\Rightarrow \mathrm{g}(\mathrm{x})>\mathrm{g}(0)=1$
$\therefore \mathrm{e}^{-2 \mathrm{x}} \cdot \mathrm{f}(\mathrm{x})>1 \forall \mathrm{x} \in(0, \infty)$
$\Rightarrow f(\mathrm{x})>\mathrm{e}^{2 \mathrm{x}} \forall \mathrm{x} \in(0, \infty)$
$\therefore$ option $(\mathrm{A})$ is correct
As, $\mathrm{f}^{\prime}(\mathrm{x})>2 \mathrm{f}(\mathrm{x})>2 \mathrm{e}^{2 \mathrm{x}}>2 \forall \mathrm{x} \in(0, \infty)$
$\Rightarrow \mathrm{f}(\mathrm{x})$ is strictly increasing on $\mathrm{x} \in(0, \infty)$
$\Rightarrow$ option $(\mathrm{C})$ is correct
As, we have proved above that $\mathrm{f}^{\prime}(\mathrm{x})>2 \cdot \mathrm{e}^{2 \mathrm{x}} \forall \mathrm{x} \in(0, \infty)$
$\Rightarrow$ option $(\mathrm{D})$ is incorrect
options $(\mathrm{A})$ and $(\mathrm{C})$ are correct
Q. Let $f: \square \rightarrow \square$ and $g: \square \rightarrow \square$ be two non-constant differentiable functions. If $f^{\prime}(x)=\left(e^{(f(x)}\right)$ $-g(x)) g^{\prime}(x)$ for all $x \in \square,$ and $f(1)=g(2)=1,$ then which of the following statement(s) is (are) TRUE?
(A) $f(2)<1-\log _{e} 2$
(B) $f(2)>1-\log _{e} 2$
(C) $\mathrm{g}(1)>1-\log _{\mathrm{e}} 2$
(D) $\mathrm{g}(1)<1-\log _{\mathrm{e}} 2$
[JEE(Advanced)-2018,4(-2)]
Ans. (B,C)
$f^{\prime}(x)=e^{(f(x)-g(x))} g^{\prime}(x) \forall x \in \square$
$\Rightarrow \quad \mathrm{e}^{-f(\mathrm{x})} \cdot f^{\prime}(\mathrm{x})-\mathrm{e}^{-\mathrm{g}(\mathrm{x})} \mathrm{g}^{\prime}(\mathrm{x})=0$
$\Rightarrow \int\left(\mathrm{e}^{-f(x)} f^{\prime}(x)-e^{-g(x)} \cdot g^{\prime}(x)\right) d x=C$
$\Rightarrow \quad-\mathrm{e}^{-f(\mathrm{x})}+\mathrm{e}^{-\mathrm{g}(\mathrm{x})}=\mathrm{C}$
$\Rightarrow \quad-\mathrm{e}^{-f(1)}+\mathrm{e}^{-\mathrm{g}(1)}=-\mathrm{e}^{-f(2)}+\mathrm{e}^{-\mathrm{g}(2)}$
$\Rightarrow \quad-\frac{1}{\mathrm{e}}+\mathrm{e}^{-\mathrm{g}(1)}=-\mathrm{e}^{-f(2)}+\frac{1}{\mathrm{e}}$
$\Rightarrow \quad \mathrm{e}^{-f(2)}+\mathrm{e}^{-\mathrm{g}(1)}=\frac{2}{\mathrm{e}}$
$\therefore \quad \mathrm{e}^{-f(2)}<\frac{2}{\mathrm{e}}$ and $\mathrm{e}^{-\mathrm{g}(1)}<\frac{2}{\mathrm{e}}$
$\Rightarrow \quad-f(2)<\ln 2-1$ and $-\mathrm{g}(1)<\ln 2-1$
$\Rightarrow f(2)>1-\ln 2$ and $g(1)>1-\ln 2$
Q. Let $f:(0, \pi) \rightarrow \square$ be a twice differentiable function such that $\lim _{t \rightarrow \pi} \frac{f(x) \sin t-f(t) \sin x}{t-x}=\sin ^{2} x$ for all $\mathrm{x} \in(0, \pi) .$ If $f\left(\frac{\pi}{6}\right)=-\frac{\pi}{12},$ then which of the following statement(s) is (are) TRUE?
(A) $f\left(\frac{\pi}{4}\right)=\frac{\pi}{4 \sqrt{2}}$
(B) $f(\mathrm{x})<\frac{\mathrm{x}^{4}}{6}-\mathrm{x}^{2}$ for all $\mathrm{x} \in(0, \pi)$
(C) There exists $\alpha \in(0, \pi)$ such that $f^{\prime}(\alpha)=0$
(D) $f^{\prime \prime}\left(\frac{\pi}{2}\right)+f\left(\frac{\pi}{2}\right)=0$
[JEE(Advanced)-2018,4(-2)]
Ans. (B,C,D)
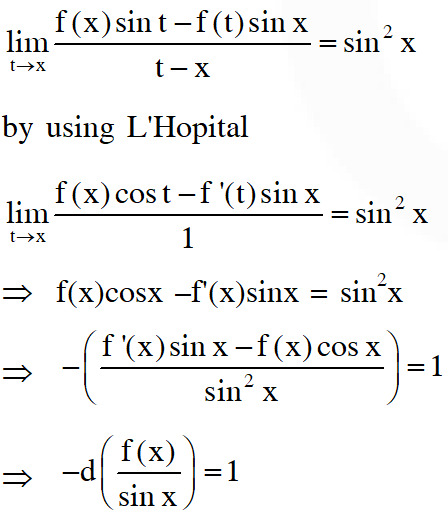


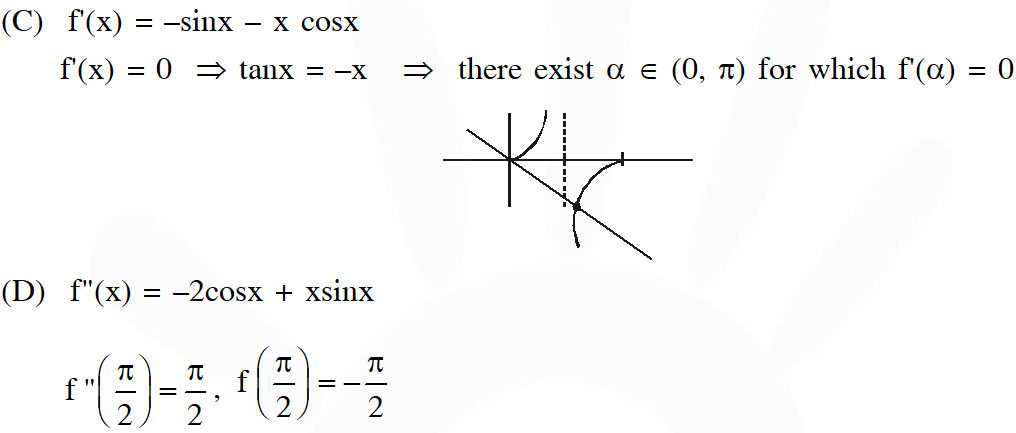

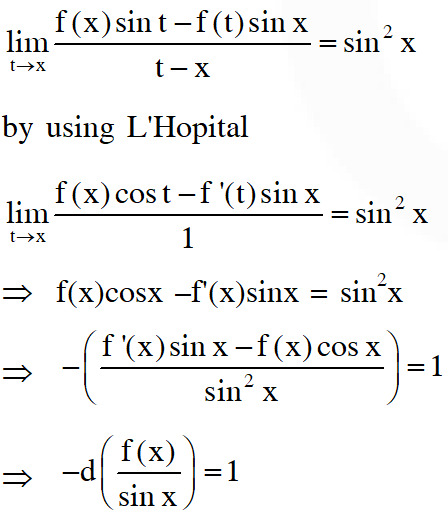

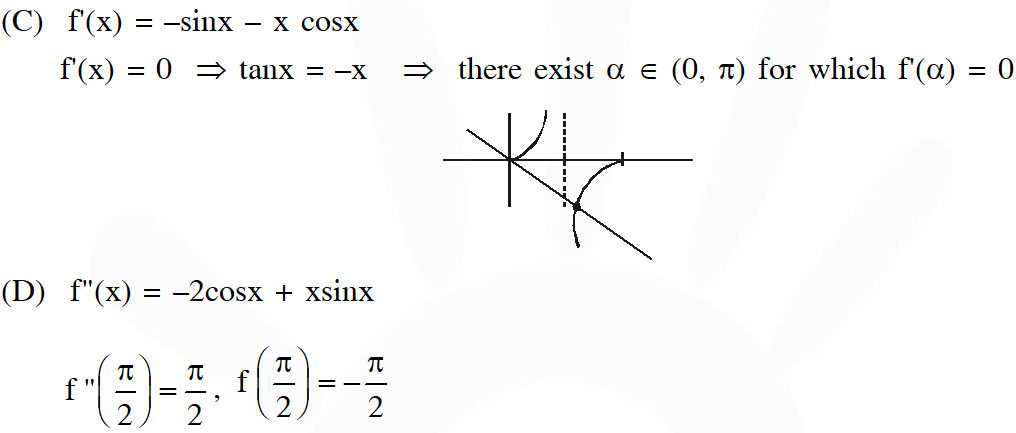
Q. Let $f: \square \rightarrow \square$ be a differentiable function with $f(0)=0 .$ If $\mathrm{y}=f(\mathrm{x})$ satisfies the differential equation $\frac{\mathrm{dy}}{\mathrm{dx}}=(2+5 \mathrm{y})(5 \mathrm{y}-2),$ then the value of $\lim _{x \rightarrow-\infty} f(\mathrm{x})$ is
[JEE(Advanced)-2018,3(0)]
Ans. 0.4




