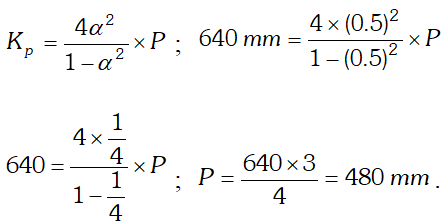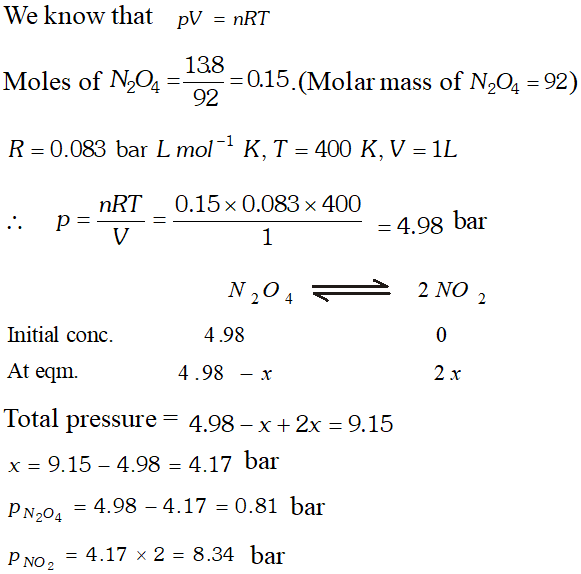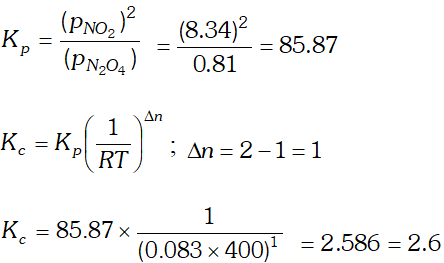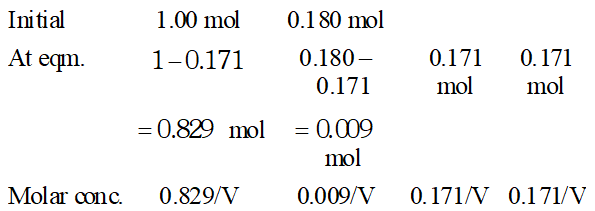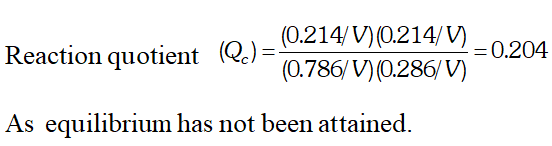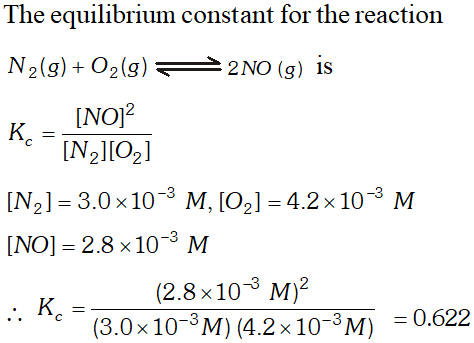Get Chemical Equilibrium important questions for Boards exams. Download or View the Important Question bank for Class 11 Chemistry. These important questions will play significant role in clearing concepts of Chemistry. This question bank is designed by NCERT keeping in mind and the questions are updated with respect to upcoming Board exams. You will get here all the important questions for class 11 chemistry chapter wise CBSE. Click Here for Detailed Chapter-wise Notes of Chemistry for Class 11th, JEE & NEET. You can access free study material for all three subject’s Physics, Chemistry and Mathematics. Click Here for Detailed Notes of any chapter.
eSaral provides you complete edge to prepare for Board and Competitive Exams like JEE, NEET, BITSAT, etc. We have transformed classroom in such a way that a student can study anytime anywhere. With the help of AI we have made the learning Personalized, adaptive and accessible for each and every one. Visit eSaral Website to download or view free study material for JEE & NEET. Also get to know about the strategies to Crack Exam in limited time period. Q. Give one example of everyday life in which there is gas $\rightleftharpoons$ solution equilibrium.
Ans. Soda-water bottle.
Q. Why gas fizzes out when soda water bottle is opened ?
Ans. The amount of the gas dissolved is very high due to high pressure. On opening the bottle, the pressure tends to decrease to atmospheric pressure. So the solubility decreases, i.e., the dissolved gas escapes out.
Q. The equilibrium constant for gas reaction is $K_{c}=\frac{\left[N H_{3}\right]^{4}\left[O_{2}\right]^{5}}{[N O]^{4}\left[H_{2} O\right]^{6}}$ Write the balance chemical reaction to this expression.
Ans. $4 \mathrm{NO}+6 \mathrm{H}_{2} \mathrm{O} \rightleftharpoons 4 \mathrm{NH}_{3}+5 \mathrm{O}_{2}$
Q. At equilibrium, the mass of each of the reactants and products remains constant. Does it mean that the reaction has stopped ? Explain.
Ans. No, the reaction does not stop. It continues to take place in the forward as well as backward direction but at equal speed.
Q. Some sugar is added into a saturated solution of sugar in a beaker. What process/processes if any, do you expect to happen with the passage of time? What is this state called?
Ans. Two processes, namely, dissolution and precipitation will continue to take place at equal rates. It is called a state of equilibrium.
Q. Give reasons in brief, indicate whether the following statement is TRUE or FALSE : The rate of an exothermic reaction increases with increasing temperature.
Ans. False because in an exothermic reaction, heat is evolved. Increase of temperature will shift the equilibrium in the backward direction, i.e., the rate of reaction decreases.
Q. What happens to a reversible reaction if a catalyst is added to it?
Ans. The state of equilibrium is not disturbed but it attained quickly because both the rate of forward and backward reaction increases to the same extent.
Q. For the following equilibrium, $K_{c}^{\prime}=6.3 \times 10^{14}$ at $1000 \mathrm{K}$ $N O(g)+O_{3}(g) \rightleftharpoons N O_{2}(g)+O_{2}(g)$ Both the forward and reverse reactions in the equilibrium are elementary bimolecular reactions. What is $K_{c}$ for the reverse reaction?
Ans. For the reverse reaction $K_{c}^{\prime}=\frac{1}{K_{c}}=\frac{1}{6.3 \times 10^{14}}$ $=1.59 \times 10^{-15}$
Q. What is meant by reaction quotient ?
Ans. It is defined as product of molar concentration of reaction products each raised to power equal to the respective stoichiometric coefficient in balance chemical equation divided by the product of concentration of the reactants raised to power equal to their individual stoichiometric coefficients at any stage of reaction, e.g., $a A+b B \rightleftharpoons c C+d D$ $Q_{C}=\frac{[C]^{c}[D]^{d}}{[A]^{a}[B]^{b}}$
Q. $\mathrm{H}_{2}(\mathrm{g})+\mathrm{I}_{2}(\mathrm{g}) \rightleftharpoons 2 \mathrm{HI}(\mathrm{g})$ What is the relationship between $\mathrm{K}_{\mathrm{p}}$ and $\mathrm{K}_{\mathrm{c}} ?$
Ans. $K_{p}=K_{c}$
Q. Predict the effect of compression on the following equilibrium reaction: $\mathrm{CH}_{4}(\mathrm{g})+\mathrm{H}_{2} \mathrm{O}(\mathrm{g}) \rightleftharpoons \mathrm{CO}(g)+3 \mathrm{H}_{2}(\mathrm{g})$
Ans. The increase of pressure i.e. compression will favour the formation of reactants because reactants have less number of moles than products.
Q. How does a catalyst affect the equilibrium constant ?
Ans. The equilibrium constant is not affected by catalyst.
Q. Vapour pressure of water, acetone and ethanol at 298 K are 2.34, 12.36 and 5.85 kPa respectively. Which of these has the lowest and the highest boiling point ? At 293 K, which of these evaporates least in a sealed container before equilibrium is established?
Ans. Acetone has highest vapour pressure, therefore has lowest boiling point. Water has lowest vapour pressure, therefore has highest boiling point. At $293 K$, water will evaporates least in a sealed container before equilibrium is established.
Q. The concentration quotient of a reversible reaction is $Q$ and the equilibrium constant is $K .$ What do you conclude if $(\text { i }) Q=K$ (ii) $Q>K$ (iii) $Q
Ans. (i) If $Q=K,$ the reaction is in equilibrium. (ii) If $Q>K, Q$ will tend to decrease so as to become equal to $K .$ As a result, the reaction will proceed in the backward direction. (iii) If $Q
Q. Explain why pure liquids and solids are ignored while writing the equilibrium constant expression.
Ans. (Pure liquid) or (Pure solid) $=\frac{\text { No. of moles }}{\text { Volume of } \mathrm{L}}=\frac{\text { Mass/mol. mass }}{\text { Volume }}$ $=\frac{\text { Mass }}{\text { Volume }} \times \frac{1}{\text { Mol. mass }}=\frac{\text { Density }}{\text { Mol. mass }}$ As density of a pure liquid or pure solid is constant constant temperature and molecular mass is also. constant, therefore, their molar concentrations are constant and included into the equilibrium constant.
Q. At a certain temperature and a total pressure of $10^{5} \mathrm{Pa}$ iodine vapour contain $40 \%$ by volume of iodine atoms $\left[\mathrm{I}_{2}(\mathrm{g}) \rightleftharpoons 2 \mathrm{I}(\mathrm{g})\right]$ Calculate $\mathrm{K}_{\mathrm{p}}$ for the equilibrium.
Ans. Partial pressure of $I$ atoms $\left(p_{1}\right)=\frac{40}{100} \times 10^{5}$ $P a=0.4 \times 10^{5} \mathrm{Pa}$ Partial pressure of $I_{2}\left(p_{I_{2}}\right)=\frac{60}{100} \times 10^{5} P a=0.60 \times 10^{5} P a$ $K_{p}=\frac{p_{I}^{2}}{P_{I_{2}}}=\frac{\left(0.4 \times 10^{5}\right)^{2}}{0.60 \times 10^{5}}=2.67 \times 10^{4}$
Q. The value of for the reaction $3 \mathrm{O}_{2}(g) \rightleftharpoons 2 \mathrm{O}_{3}(\mathrm{g})$ is $2.0 \times 10^{-50}$ at $25^{\circ} \mathrm{C}$. If the equilibrium concentration of $\mathrm{O}_{2}$ in air at $25^{\circ} \mathrm{C}$ is $1.6 \times 10^{-2},$ what is the concentration of $\mathrm{O}_{3} ?$
Ans. $K_{c}=\frac{\left[O_{3}\right]^{2}}{\left[O_{2}\right]^{3}} \therefore 2.0 \times 10^{-50}=\frac{\left[O_{3}\right]^{2}}{\left(1.6 \times 10^{-2}\right)^{3}}$ or $\left[\mathrm{O}_{3}\right]^{2}=\left(2.0 \times 10^{-50}\right)\left(1.6 \times 10^{-2}\right)^{3}=8.192 \times 10^{-56}$ or $\left[O_{3}\right]=2.86 \times 10^{-28} M$
Q. The value $\Delta \mathrm{G}^{\circ}$ of for the phosphorylation of glucose in glycolysis is $13.8 \mathrm{kJ} \mathrm{mol}^{-1} .$ Find the value of $\mathrm{K}_{\mathrm{c}}$ at $298 \mathrm{K}$
Ans. $\Delta G=13.8 \mathrm{kJ} \mathrm{mol}^{-1}=13.8 \times 10^{3} \mathrm{J} \mathrm{mol}^{-1}$ $\Delta G^{\circ}=-2.303 R T \log K$ $\log K=-\frac{\Delta G^{\circ}}{2.303 R T}=-\frac{13.8 \times 10^{3}}{2.303 \times 8.314 \times 298}=-2.418$ $K=3.82 \times 10^{-3}$
Q. Calculate (a) $\Delta \mathrm{G}^{\circ}$ and (b) the equilibrium constant for the formation of $\mathrm{NO}_{2}$ from $\mathrm{NO}$ and $\mathrm{O}_{2}$ at $298 \mathrm{K}$.
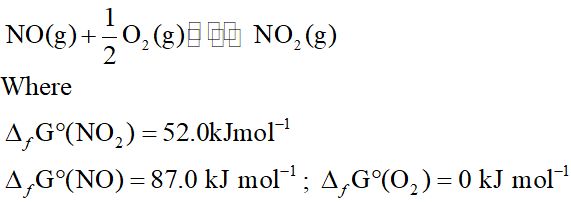
Ans. $N O(g)+\frac{1}{2} O_{2}(g) \rightleftharpoons N O_{2}(g)$ $\Delta G^{\circ}=\Delta_{f} G^{\circ}\left(N O_{2}\right)-\left[\Delta_{f} G^{\circ}(N O)+\frac{1}{2} \Delta_{f} G^{\circ}\left(O_{2}\right)\right]$ $=52.0-87.0-\frac{1}{2} \times 0=-35 \mathrm{kJ} \mathrm{mol}^{-1}$ $\mathrm{Now}, \log K=-\frac{\Delta G^{\circ}}{2.303 R T}$ $=-\frac{-35 \times 10^{3} \mathrm{J} \mathrm{mol}^{-1}}{2.303 \times 8.314 \mathrm{JK}^{-1} \mathrm{mol}^{-1} \times 298 \mathrm{K}}=6.314$ or $K=1.362 \times 10^{6}$
Q. At $450 K, \mathrm{K}_{\mathrm{p}}=2.0 \times 10^{10} /$ bar for the given reaction at equilibrium $: 2 \mathrm{SO}_{2}(\mathrm{g})+\mathrm{O}_{2}(\mathrm{g}) \rightleftharpoons 2 \mathrm{SO}_{2}(\mathrm{g})$ What is the $\mathrm{K}_{\mathrm{c}}$ at this temperature?
Ans. $K_{p}=K_{c}(R T)^{\Delta n}$ or $K_{c}=\frac{K_{p}}{(R T)^{\Delta n}}$ $\Delta n=2-(2+1)=-1, T=450 K, R=0.083$ bar $K^{-1} \mathrm{mol}^{-1}$ $\therefore K_{C}=\frac{2.0 \times 10^{10}}{(0.083 \times 450)^{-1}}=2.0 \times 10^{10} \times(0.083 \times 450)$ $=7.47 \times 10^{11} M^{-1}$
Q. Two moles of $\mathrm{NH}_{3}$ are introduced in a previously evacuated container of capacity one litre which is partially dissociated at high temperature as $2 \mathrm{NH}_{3}(\mathrm{g}) \rightleftharpoons \mathrm{N}_{2}(\mathrm{g})+3 \mathrm{H}_{2}(\mathrm{g}),$ At equilibrium one mole of remains. What is the value of $\mathrm{k}_{\mathrm{C}} ?$
Ans. 
Q. 1 mole of $\mathrm{H}_{2} \mathrm{O}$ and 1 mole of $\mathrm{CO}$ are taken in a 10 litre vessel and heated to $725 \mathrm{K}$. At equilibrium, $40 \%$ of $\mathrm{H}_{2} \mathrm{O}$ (by mass) reacts with CO according to the equation: $\mathrm{H}_{2} \mathrm{O}(\mathrm{g})+\mathrm{CO}(\mathrm{g}) \rightleftharpoons \mathrm{H}_{2}(\mathrm{g})+\mathrm{CO}_{2}(\mathrm{g})$
Ans. 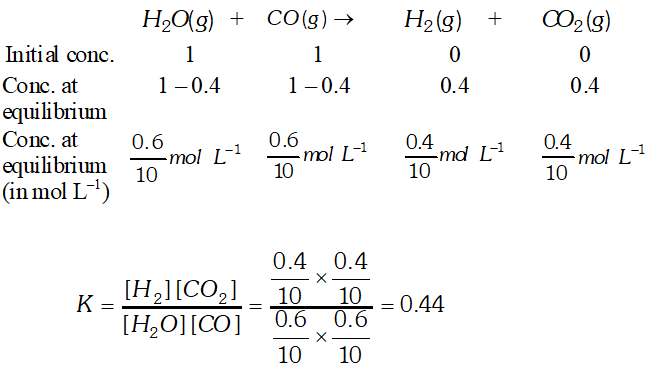
Q. At $700 K$ the equilibrium constant for the reaction $\mathrm{H}_{2}(\mathrm{g})+\mathrm{I}_{2}(\mathrm{g}) \rightleftharpoons 2 \mathrm{HI}(\mathrm{g})$ is $54.8 .$ If $0.5 \mathrm{mol} \mathrm{L}^{-1}$ of $\mathrm{HI}(\mathrm{g})$ is present at equilibrium at $700 K,$ what are the concentrations of $\mathrm{H}_{2}(\mathrm{g})$ and $\mathrm{I}_{2}(\mathrm{g})$ assuming that we initially started with HI (g) and allowed it to reach equilibrium at $700 K ?$
Ans. 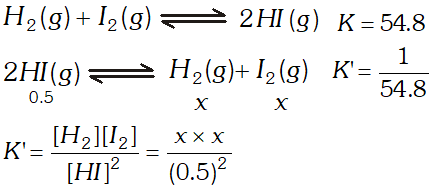 $\frac{1}{54.8}=\frac{x^{2}}{(0.5)^{2}} \Rightarrow x^{2}=\frac{0.5 \times 0.5}{54.8} \Rightarrow x=0.0675 \mathrm{mol} L^{-1}$
$\frac{1}{54.8}=\frac{x^{2}}{(0.5)^{2}} \Rightarrow x^{2}=\frac{0.5 \times 0.5}{54.8} \Rightarrow x=0.0675 \mathrm{mol} L^{-1}$ Q. Reaction between nitrogen and oxygen takes place as following $: 2 \mathrm{N}_{2}(\mathrm{g})+\mathrm{O}_{2}(\mathrm{g}) \rightleftharpoons 2 \mathrm{NO}_{2}(\mathrm{g})$ If a mixture of $0.482 \mathrm{mol}$ of $\mathrm{N}_{2}$ and $0.933 \mathrm{mol}$ of $\mathrm{O}_{2}$ is placed in a reaction vessel of volume $10 L$ and allowed to form $\mathrm{N}_{2} \mathrm{O}$ at a temperature for which $\mathrm{K}_{\mathrm{c}}=2.0 \times 10^{-37}$ determine the composition of equilibrium mixture
Ans. $2 N_{2}(g)+O_{2}(g) \rightleftharpoons 2 N_{2} O(g)$ Concentration of $N_{2}=0.482 \mathrm{mol}$ Molar concentration of $N_{2}$ at equilibrium $=\frac{0.482}{10 L}=0.0482 \mathrm{mol} \mathrm{L}^{-1}$ $=\frac{0.482}{10 L}=0.0482 \mathrm{mol} L^{-1}$ Molar concentration of $O_{2}$ at equilibrium $=\frac{0.953}{10 L}=0.0933 \mathrm{mol} L^{-1}$ $K_{c}=\frac{\left[N_{2} \mathrm{O}\right]^{2}}{\left[N_{2}\right]^{2}\left[\mathrm{O}_{2}\right]^{1}} \Rightarrow 2.0 \times 10^{-37}=\frac{\left[N_{2} \mathrm{O}\right]^{2}}{[0.0482]^{2}[0.0933]}$ $\left[N_{2} \mathrm{O}\right]=6.6 \times 10^{-21} \mathrm{mol} L^{-1}$
Q. A sample of pure $\mathrm{PCl}_{5}$ was introduced into an evacuated vessel at $473 \mathrm{K.}$ After equilibrium was attained, concentration of $\mathrm{PCl}_{5}$ was found to be $0.5 \times 10^{-1} .$ If value of $\mathrm{K}$ is $8.3 \times 10^{-3},$ what are the concentrations of $\mathrm{PCl}_{3}$ and $\mathrm{Cl}_{2}$ at equilibrium?
Ans. 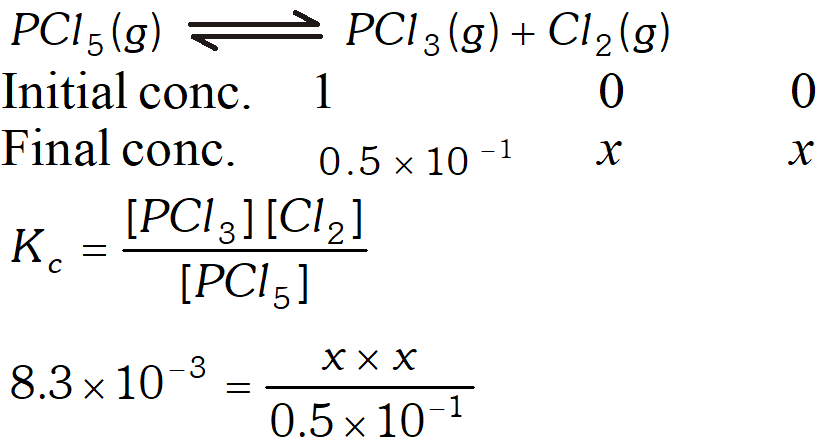
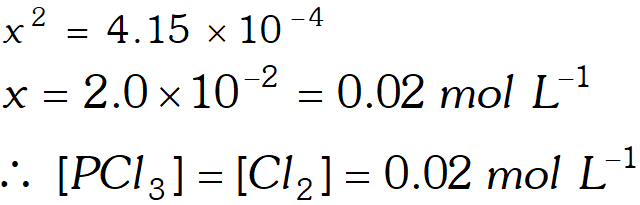
Q. For the reaction $C H_{4}(g)+2 H_{2} S(g) \rightleftharpoons C S_{2}(g)+4 H_{2}(g)$ at $1173 K$ the magnitude of the equilibrium constant, $K_{c}$ is $3.6 .$ For each of the following compositions, decide whether reaction mixture is at equilibrium. If it is not, decide which direction the reaction should go: (i) $\left[\mathrm{CH}_{4}\right]=1.07 \mathrm{M},\left[\mathrm{H}_{2} \mathrm{S}\right]=1.20 \mathrm{M},\left[\mathrm{CS}_{2}\right]=0.90 \mathrm{M},\left[\mathrm{H}_{2}\right]=1.78 \mathrm{M}$ (ii) $\left[C H_{4}\right]=1.45 M,\left[H_{2} S\right]=1.29 M,\left[C S_{2}\right]=1.25 M,\left[H_{2}\right]=1.75 M$
Ans. $C H_{4}(g)+2 H_{2} S(g) \rightleftharpoons C S_{2}(g)+4 H_{2}(g)$ (i) $\quad Q_{c}=\frac{\left[C S_{2}\right]\left[H_{2}\right]^{4}}{\left[C H_{4}\right]\left[H_{2} S\right]^{2}}=\frac{[0.90][1.78]^{4}}{[1.07][1.20]^{2}}=\frac{10.03 \times 0.9}{1.44 \times 1.07}$ $K_{c}=3.6 \quad Q_{c}=\frac{9.27}{1.54}=6.02$ since $Q_{c}>K_{c},$ the equilibrium will shift in backward direction. (ii) $\quad Q_{c}=\frac{[1.25][1.75]^{4}}{[1.45][1.29]^{2}}=\frac{9.38 \times 1.25}{1.45 \times 1.66}=\frac{11.725}{2.407}=4.86$ Since , the equilibrium will shift in backward direction.
Q. Consider the following equilibrium: $P C l_{5}(g) \rightleftharpoons P C l_{3}(g)+C l_{2}(g)$ Initially, $0.0646 \mathrm{M} \mathrm{PCl}_{5}$ is introduced into a reaction vessel $350 \mathrm{K} .$ At equilibrium, $0.0444 \mathrm{M} \mathrm{Cl}_{2}$ is found. Calculate $K_{c}$ and $K_{p}$
Ans. 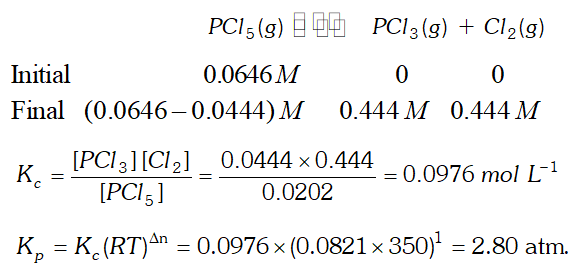
Q. A mixture of $1.57 \mathrm{mol}$ of $N_{2}, 1.92$ mol of $\mathrm{H}_{2}$ and $8.13 \mathrm{mol}$ of $N H_{3}$ is introduced into a $20 L$ reaction vessel at 500 K. At this temperature, the equilibrium constant, $K_{c}$ for the reaction, $N_{2}(g)+3 H_{2}(g) \rightleftharpoons 2 N H_{3}(g)$ is $1.7 \times 10^{2}$ Is the reaction mixture at equilibrium ? If not, what is the direction of the net reaction?
Ans. 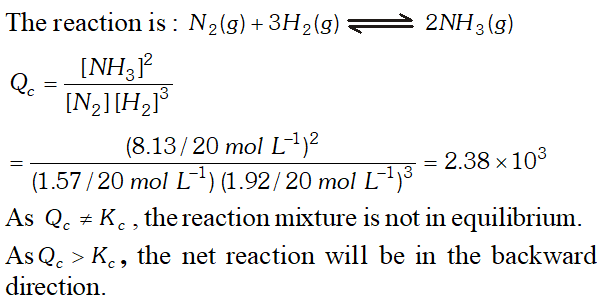
Q. Equilibrium constant, $K_{c}$ for the reaction $N_{2}(g)+3 H_{2}(g) \rightleftharpoons 2 N H_{3}(g)$ at $500 K$ is 0.061 At a panticular time, the analysis shows that composition of the reaction mixture is $30 \mathrm{mol}^{-1} N_{2}, 20 \mathrm{moL}^{-1} \mathrm{H}_{2}$ and $0.5 \mathrm{mol} L^{-1} \mathrm{NH}_{3} .$ Is the reaction at equilibrium? If not, in which direction does the reaction tend to proceed to reach equilibrium?
Ans. $Q_{c}=\frac{\left[N H_{3}\right]^{2}}{\left[N_{2}\right]\left[H_{2}\right]^{3}}=\frac{(0.5)^{2}}{(3.0)(2.0)^{3}}=0.0104$ As $Q_{c} \neq K_{c},$ reaction is not in equilibrium. As $Q_{c}
Q. Hydrogen gas used in Haber's process is produced by reacting methane from natural gas with high temperature steam. The first stage of two stage reaction involves the formation of $\mathrm{CO}$ and $\mathrm{H}_{2} .$ In second stage, $\mathrm{CO}$ formed in first stage is reacted with more steam in water gas shift reaction, $C O(g)+H_{2} O(g) \rightleftharpoons C O_{2}(g)+H_{2}(g) .$ If a reaction vessel at $400^{\circ} \mathrm{C}$ is charged with an equimolar mixture of $\mathrm{CO}$ and steam such that $p_{\mathrm{CO}}=p_{H_{2} O}=4.0$ bar, what will be the partial pressure of $H_{2}$ at equilibrium? $K_{p}=0.1$ at $400^{\circ} \mathrm{C}$
Ans. 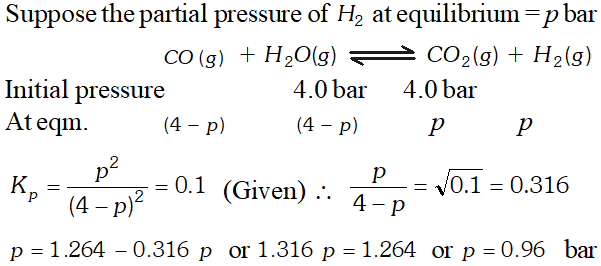
Q. Predict which of the following reaction will have appreciable concentration of reactants and products :
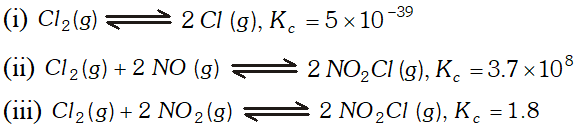
Ans. For reaction (iii), as $\mathrm{K}_{\mathrm{c}}$ neither high nor very low, reactants and products will be present in comparable amounts.
Q. At equilibrium the concentration of $N_{2}, O_{2}$ and $N O$ in a sealed vessel at $800 K$ are: $N_{2}=3.0 \times 10^{3} M, O_{2}=4.2 \times 10^{3} M \&$ $N O=2.8 \times 10^{-3} \mathrm{M} \cdot$ Calculate the equilibrium constant $K_{c}$ for the reaction $: N_{2}(g)+O_{2}(g) \rightleftharpoons 2 N O(g)$
Ans. 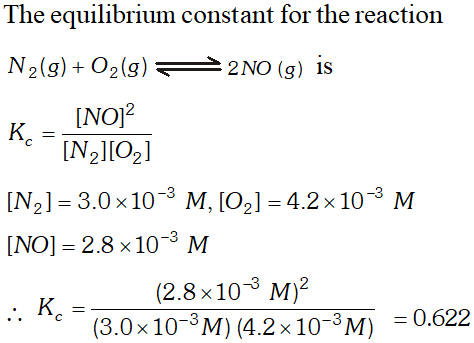
Q. $P C l_{5}, P C l_{3}$ and $C l_{2}$ are at equilibrium of $500 K$ and having concentration $1.59 M P C l_{3}, 1.59 M C l_{2}$ and $1.41 M P C l_{5} .$ Calculate $K_{c}$ for the reaction: $P C l_{5} \rightleftharpoons P C l_{3}+C l_{2}$
Ans. The equilibrium constant for the reaction may be written as $K_{c}=\frac{\left[P C l_{3}\right]\left[C l_{2}\right]}{\left[P C l_{5}\right]}$ $\left[P C l_{5}\right]=1.41 M,\left[P C l_{3}\right]=1.59 M,\left[C l_{2}\right]=1.59 M$ $=\frac{(1.59)(1.59)}{1.41}=1.79 M$
Q. Which of the following reaction will get effected by increasing the pressure ? Also mention whether change will cause the reaction to go into forward or backward direction ? (i) $\mathrm{COCl}_{2}(g) \rightleftharpoons \mathrm{CO}(g)+\mathrm{Cl}_{2}(g)$ (ii) $\mathrm{CH}_{4}(\mathrm{g})+2 \mathrm{S}_{2}(\mathrm{g}) \rightleftharpoons \mathrm{CS}_{2}(l)+2 \mathrm{H}_{2} \mathrm{S}(g)$ (iii) $\mathrm{CO}_{2}(g)+C(s) \rightleftharpoons 2 \mathrm{CO}(g)$ (iv) $2 H_{2}(g)+C O(g) \rightleftharpoons C H_{3} O H(g)$ (v) $\mathrm{CaCO}_{3}(\mathrm{s}) \rightleftharpoons \mathrm{CaO}(\mathrm{s})+\mathrm{CO}_{2}(\mathrm{g})$ (vi) $4 N H_{3}(g)+5 O_{2}(g) \rightleftharpoons 4 N O(g)+6 H_{2} O(g)$
Ans. (i) Backward direction (ii) Forward direction (iii) Backward direction (iv) Forward direction (v) Backward direction. (vi) Backward direction.
Q. For the reaction $N_{2}(g)+3 H_{2}(g) \rightleftharpoons 2 N H_{3}(g)$ at $400 \mathrm{K}, \mathrm{K}_{\mathrm{p}}=41 .$ Find the value of $\mathrm{K}_{\mathrm{p}}$ for each of the following reactions at the same temperature: (i) $2 N H_{3}(g) \rightleftharpoons N_{2}(g)+3 H_{2}(g)$ (ii) $\frac{1}{2} N_{2}(g)+\frac{3}{2} H_{2}(g) \rightleftharpoons N H_{3}(g)$ (iii) $2 N_{2}(g)+6 H_{2}(g) \rightleftharpoons 4 N H_{3}(g)$
Ans. (i) $\quad K_{p}=\frac{\left[N H_{3}\right]^{2}}{\left[N_{2}\right]\left[H_{2}\right]^{3}}=41$ $2 N H_{3}(g) \rightleftharpoons N_{2}(g)+3 H_{2}(g)$ $K_{p}^{\prime}=\frac{\left[N_{2}\right]\left[H_{2}\right]^{3}}{\left[N H_{3}\right]^{2}}=\frac{1}{K_{p}}=\frac{1}{41}=0.024$ (ii) $\frac{1}{2} N_{2}(g)+\frac{3}{2} H_{2}(g) \rightleftharpoons N H_{3}(g)$ $K_{p}^{\prime \prime}=\frac{\left[N H_{3}\right]}{\left[N_{2}\right]^{1 / 2}\left[H_{2}\right]^{3 / 2}}=\sqrt{K_{p}}=\sqrt{41}=6.4$ (iii) $2 N_{2}(g)+6 H_{2}(g) ! 4 N H_{3}(g)$ $K_{p}^{\mathrm{n}}=\frac{\left[N H_{3}\right]^{4}}{\left[N_{2}\right]^{2}\left[H_{2}\right]^{6}}=\left(K_{p}\right)^{2}=(41)^{2}=1681$ $K_{p}^{\prime \prime}=1.68 \times 10^{3}$
Q. The value of $K_{c}=4.24$ at $800 K$ for the reaction $\mathrm{CO}(g)+H_{2} \mathrm{O}(g) \rightleftharpoons \mathrm{CO}_{2}(g)+H_{2}(g) \quad$ Calculate equilibrium concentrations of $\mathrm{CO}_{2}, \mathrm{H}_{2}, \mathrm{CO}$ and $\mathrm{H}_{2} \mathrm{O}$ at $800 K,$ if only $\mathrm{CO}$ and $\mathrm{H}_{2} \mathrm{O}$ are present initially at concentration of $0.10 \mathrm{Meach} ?$
Ans. 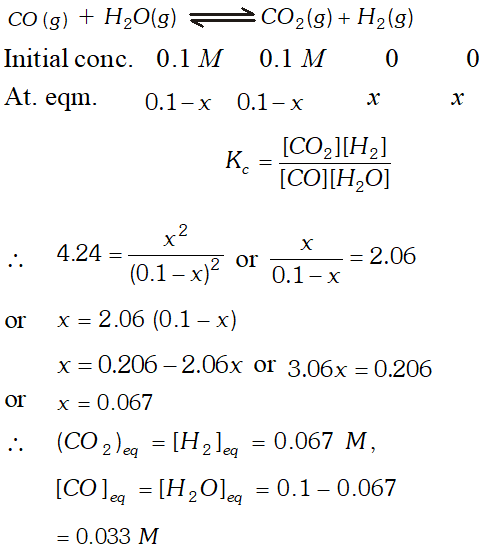
Q. What is the equilibrium concentration of each of the substances in the equilibrium when the initial concentration of ICI was 0.78 M ? $2 I C l(g) \rightleftharpoons I_{2}(g)+C l_{2}(g), K_{c}=0.14$
Ans. 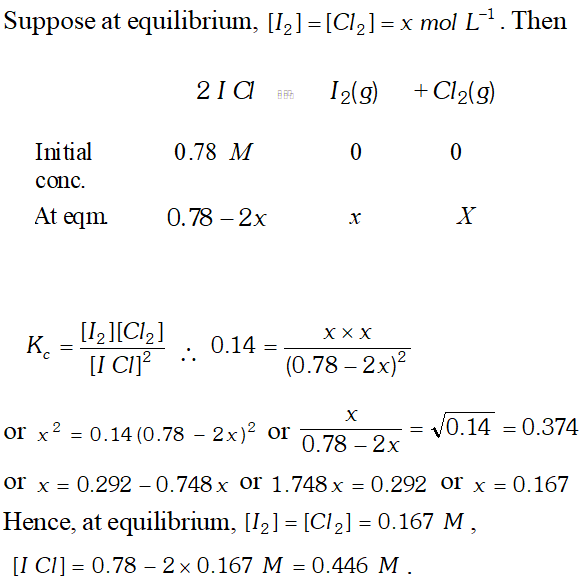
Q. $K_{p}=0.04$ atm at $899 \mathrm{K}$ for the equilibrium shown below. What is the equilibrium concentration of $C_{2} H_{6}$ when it is placed in a flask at 4.0 atm. Pressure and allowed to come in equilibrium? $C_{2} H_{6}(g) \rightleftharpoons \mathrm{C}_{2} \mathrm{H}_{4}(\mathrm{g})+\mathrm{H}_{2}(\mathrm{g})$
Ans. 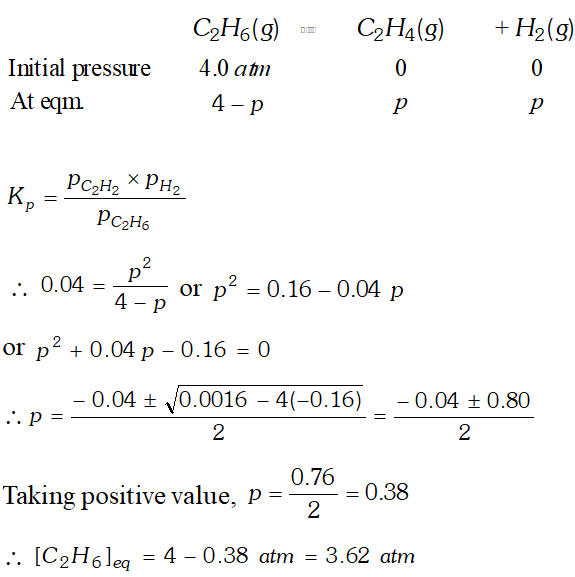
Q. Find out the value of $K_{c}$ for each of the following equilibrium from the value of $K_{p}$ (i) $2 N O C l(g) \rightleftharpoons 2 N O(g)+C l_{2}(g), K_{p}=1.8 \times 10^{-2}$ at 500 $$ \begin{array}{l}{K} \\ { \text { (ii) }\left.C a C O_{3}(s) \Longrightarrow C a Q_{s}\right)+C O_{2}(g), K_{p}=167 \text { at } 1073 K .}\end{array} $$
Ans. (i) $\quad 2 \mathrm{NOCl}(g) \rightleftharpoons 2 \mathrm{NO}(g)+\mathrm{Cl}_{2}(g)$ $K_{p}=1.8 \times 10^{-2} \Rightarrow \Delta n_{g}=3-2=1$ $K_{p}=K_{c}(R T)^{\Delta n}=K_{c}(R T)$ $K_{c}=\frac{K_{p}}{R T}=\frac{1.8 \times 10^{-2}}{0.0831 \times 500}=4.33 \times 10^{-4}$ (ii) $\quad \mathrm{CaCO}_{3}(s) \rightleftharpoons \mathrm{CaO}(s)+\mathrm{CO}_{2}(g)$ $K_{p}=167$ $\Delta n_{g}=1$ $K_{p}=K_{c}(R T)^{\Delta n}=K_{c}(R T)$ $K_{c}=\frac{K_{p}}{R T}=\frac{167}{0.0831 \times 1073}=1.88$
Q. At 1127 and 1 atm pressure, a gaseous mixture of $C O$ and $\mathrm{CO}_{2}$ in equilibrium with solid carbon has $90.55 \%$ $\mathrm{CO}$ by mass, $\mathrm{C}(s)+\mathrm{CO}_{2} \rightleftharpoons 2 \mathrm{CO}(\mathrm{g})$ Calculate $K_{c}$ for this reaction at the above temperature.
Ans. Let total mass of the gaseous mixture be $=100 \mathrm{g}$ Mass of $C O=90.55 \mathrm{g}$ Mass of $C O_{2}=9.45 g$ Moles of $C O=\frac{90.55}{28}=3.234$ Moles of $C O_{2}=\frac{9.45}{44}=0.215$ Total number of moles of gases $=3.234+0.215=3.449$ $p_{C O}=\frac{3.234}{3.449} \times 1=0.938 \mathrm{atm}$ $p_{C O_{2}}=\frac{0.215}{3.449} \times 1=0.0623 \mathrm{atm}$ $K_{p}=\frac{p_{C O}^{2}}{p_{C O_{2}}}=\frac{(0.938)^{2}}{0.0623}=14.12$ $K_{p}=K_{c}(R T)^{\Delta n}=K_{c}(R T) \quad \therefore \Delta n=(2-1)=1$ $K_{c}=\frac{K_{p}}{R T}=\frac{14.12}{0.0821 \times 1127}=0.153$
Q. The equilibrium constant for the following reaction is $1.6 \times 10^{5}$ at $1024 \mathrm{K}$ $H_{2}(g)+B r_{2}(g) \rightleftharpoons 2 H B r(g)$ Find the equilibrium pressure of all gases if 10.0 bar $\mathrm{HBr}$ is introduced into a sealed container at $1024 K$
Ans. 
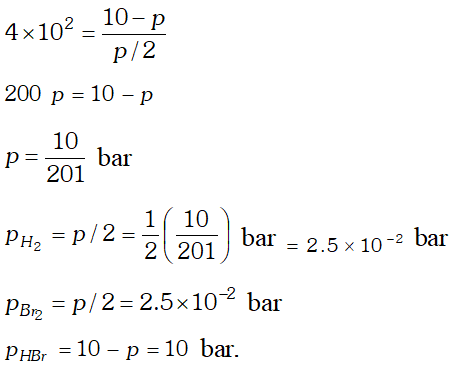
Q. What is $K_{c}$ for the following equilibrium, when the equilibrium concentration of each substance is : $\left[\mathrm{SO}_{2}\right]=0.60 \mathrm{M},\left[\mathrm{O}_{2}\right]=0.82 \mathrm{M}$ and $\left[\mathrm{SO}_{3}\right]=1.90 \mathrm{M}$ $2 S O_{2}(g)+O_{2}(g) \rightleftharpoons 2 \mathrm{SO}_{3}(\mathrm{g})$
Ans. The equilibrium constant, $K_{c}$ is $K_{c}=\frac{\left[S O_{3}\right]^{2}}{\left[S O_{2}\right]^{2}\left[O^{2}\right]}$ $\left[\mathrm{SO}_{2}\right]=0.60 \mathrm{M},\left[\mathrm{O}_{2}\right]=0.82 \mathrm{M}$ and $\left[\mathrm{SO}_{3}\right]=1.90 \mathrm{M}$ $K_{c}=\frac{(1.90 M)^{2}}{(0.60 M)^{2}(0.82 M)}$ $=12.229 M^{-1}$ or $12.229 \mathrm{mol}^{-1} L$
Q. Nitric oxide reacts with bromine and gives nitrosyl bromide according to the reaction: $2 \mathrm{NO}(g)+B r_{2}(g) \rightleftharpoons 2 \mathrm{NOBr}(g)$ When $0.087 \mathrm{mol}$ of $\mathrm{NO}$ and $0.0437 \mathrm{mol}$ of $\mathrm{Br}_{2}$ are mixed in a closed container at constant temperature $0.518 \mathrm{mol}$ of $\mathrm{NOBr}$ is obtained at equilibrium. Calculate (i) the equilibrium amount of nitric oxide and bromine (ii) equilibrium constant.
Ans. $2 \mathrm{NO}(g)+B r_{2}(g) \rightleftharpoons 2 \mathrm{NOBr}(g)$ (i) According to the above equation 0.0518 mol of $\mathrm{NOBr}$ are formed from 0.0518 mol of $N O$ $\therefore$ Amount of nitric oxide at equilibrium $=0.087-0.0518$ $=0.0352 \mathrm{mol}$ Similarly, $0.0518 \mathrm{mol}$ of $\mathrm{NOBr}$ are formed from $\frac{0.0518}{2} \mathrm{mol}$ of $\mathrm{Br}_{2}$ $\therefore$ Amount of bromine at equilibrium $=0.0437-\frac{0.0518}{2}=0.0178 \mathrm{mol}$ (ii) $\quad K_{c}=\frac{[N O B r]^{2}}{\left[N O^{2}\right]\left[B r_{2}\right]}$ At equilibrium $[\mathrm{NOBr}]=0.0518 \mathrm{mol},[\mathrm{NO}]=0.0352 \mathrm{mol}$ $\left[B r_{2}\right]=0.0178 \mathrm{mol}$ $\therefore \quad K_{c}=\frac{(0.0518)^{2}}{(0.0352)^{2} \times(0.0178)}=121.66$
Q. Which of the following reactions involve homogeneous equilibria and which involve heterogeneous equilibria ? (i) $2 N_{2} O(g) \longrightarrow 2 N_{2}(g)+O_{2}(g)$ (ii) $2 N H_{3}(g) \longrightarrow N_{2}(g)+3 H_{2}(g)$ (iii) $2 C u\left(N O_{3}\right)_{2}(s) \longrightarrow 2 C u O(s)+4 N O_{2}(g)+O_{2}(g)$ (iv) $\mathrm{CH}_{3} \mathrm{COOC}_{2} \mathrm{H}_{5}(a q)+\mathrm{H}_{2} \mathrm{O}(l) \longrightarrow$ $\mathrm{CH}_{3} \mathrm{COOH}(a q)+\mathrm{C}_{2} \mathrm{H}_{5} \mathrm{OH}(a q)$ (v) $\mathrm{Fe}^{3+}(a q)+3 \mathrm{OH}(a q) \longrightarrow \mathrm{Fe}(\mathrm{OH})_{3}(s) .3$
Ans. (i) Homogeneous Equilibria (ii) Homogeneous Equilibria (iii) Heterogeneous Equilibria (iv) Homogeneous Equilibria (v) Heterogeneous Equilibria
Q. Hydrogen gas is obtained from natural gas by partial oxidation with steam as per following endothermic reaction: $C H_{4}(g)+H_{2} O(g) \rightleftharpoons C O(g)+3 H_{2}(g)$ (i) Write an expression of $K_{p}$ for the above reaction. (ii) How will the value of $K_{p}$ and composition of equilibrium mixture be affected by (a) increasing the pressure; $(b)$ increasing the temperature; (c) using a catalyst?
Ans. $C H_{4}(g)+H_{2} O(g) \rightleftharpoons C O(g)+3 H_{2}$ (i) $\quad K_{p}=\frac{\left(P_{\mathrm{CO}}\right)\left(P_{\mathrm{H}_{2}}\right)^{3}}{\left(P_{\mathrm{CH}_{4}}\right)\left(P_{\mathrm{H}_{2} \mathrm{O}}\right)}$ (ii) $\quad K_{p}$ is not affected by increasing pressure (b) will increase with increase in temperature because it is endothermic process. (c) is not affected by catalyst.
Q. Reaction between nitrogen and oxygen takes place as follows : $2 N_{2}(g)+O_{2}(g) \rightleftharpoons 2 N_{2} O(g)$ If mixture of $0.482 \mathrm{mol}$ of $N_{2}$ and $0.933 \mathrm{mol}$ of $\mathrm{O}_{2}$ is placed in a reaction vessel of volume $10 L$ and allowed to from $N_{2} O$ at a temperature for which $K_{c}=2.0 \times 10^{-37}$ Determine the composition of the equilibrium mixture.
Ans. 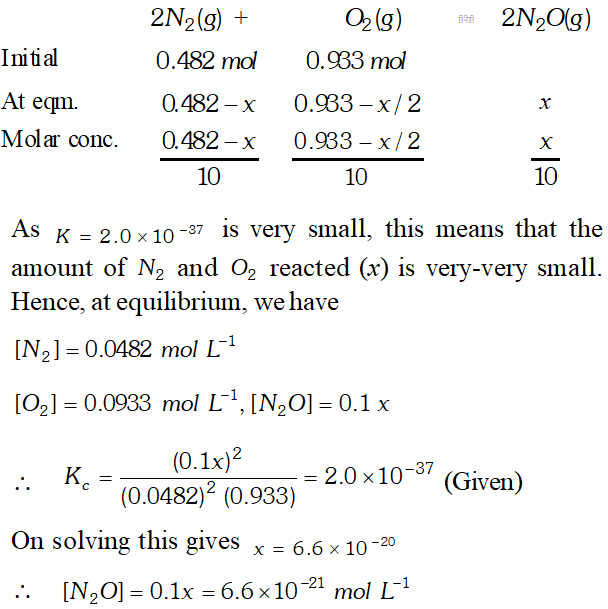
Q. One of the reactions that takes place in producing steel from iron ore is the reduction of iron (II) oxide by carbon monoxide to given iron metal and $C O_{2}$ $F e O(s)+C O(g) \rightleftharpoons F e(s)+C O_{2}(g) ; K_{p}=0.265 \quad$ at $1050 K$ What are the equilibrium partial pressures of $\mathrm{CO}$ and $\mathrm{CO}_{2}$ at $1050 \mathrm{K}$ if the initial pressure are : $p_{\mathrm{CO}}=1.4$ atm and $p_{C O_{2}}=0.80$ atm ?
Ans. 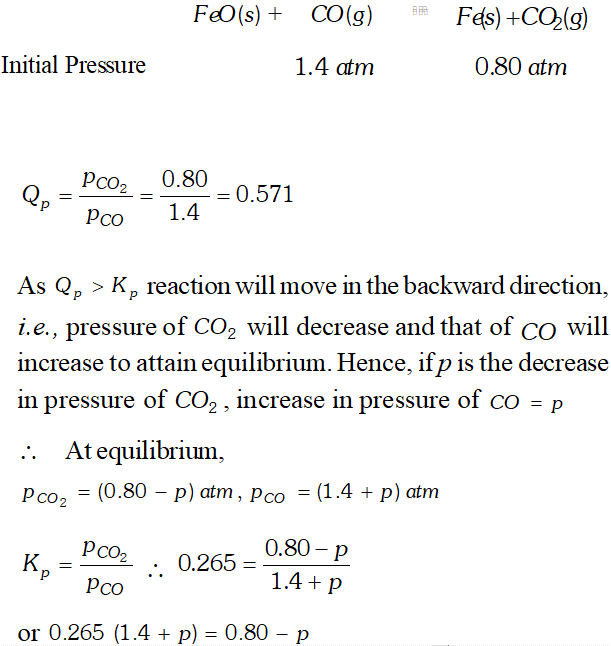
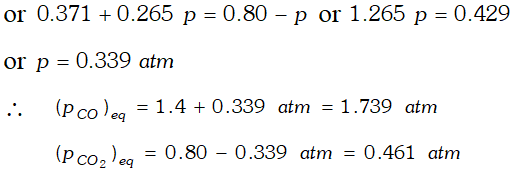
Q. Equilibrium constant, $K_{c}$ for the reaction, $N_{2}(g)+3 H_{2}(g) \rightleftharpoons 2 N H_{3}(g)$ at $500 K$ is $0.061 .$ At a particular time, the analysis shows that composition, of the reaction mixture is 3.00 $m o l$ $L^{-1} N_{2}, 2.00$ mol $L^{-1} H_{2}, 0.500 \mathrm{mol} \mathrm{L}^{-1} \mathrm{NH}_{3} .$ Is the reaction at equilibrium ? If not, in which direction does the reaction tend to proceed to reach equilibrium?
Ans.  since $Q_{c}$ is not equal to $K_{c},$ the reaction is not at equilibrium. since is less than, the reaction would tend to proceed in forward direction to attain equilibrium
since $Q_{c}$ is not equal to $K_{c},$ the reaction is not at equilibrium. since is less than, the reaction would tend to proceed in forward direction to attain equilibrium Q. Describe the effect of: (i) addition of $H_{2}$ (ii) addition of $C H_{3} O H$ (iii) removal of $\mathrm{CO}$ (iv) removal of $\mathrm{CH}_{3} \mathrm{OH}$ on the equilibrium of the reaction: $2 H_{2}(g)+C O(g) \rightleftharpoons C_{H_{3}} O H(g)$
Ans. (i) Equilibrium will shift in the forward direction. (ii) Equilibrium will shift in the backward direction. (iii) Equilibrium will shift in the backward direction. (iv) Equilibrium will shift in the forward direction.
Q. Which of the following reactions will be affected by increase of pressure ? Also mention whether change will cause the reaction to go into the right or left direction. (i) $\mathrm{CH}_{4}(\mathrm{g})+2 \mathrm{S}_{2}(\mathrm{g}) \rightleftharpoons \mathrm{CS}_{2}(\mathrm{g})+2 \mathrm{H}_{2} \mathrm{S}(g)$ (ii) $\mathrm{CO}_{2}(g)+C(g) \rightleftharpoons 2 \mathrm{CO}(g)$ (iii) $4 N H_{3}(g)+5 O_{2}(g) \rightleftharpoons 4 N O(g)+6 H_{2} O(g)$ (iv) $C_{2} H_{4}(g)+H_{2}(g) \rightleftharpoons C_{2} H_{6}(g)$
Ans. $\Delta n=$ number of moles of gaseous products - number of moles of gaseous reactants (i) $\quad \Delta n=3-3=0 \quad:$ Therefore, no effect of pressure because number of moles are equal on both sides. (ii) $\quad \Delta n=2-2=0:$ Therefore, no effect of pressure because number of moles are equal on both sides. (iii) $\quad \Delta n=10-9=1:$ since there is increase in number of moles on product side. Therefore, increase in pressure will favour backward reaction $i . e .,$ the reaction will go into left direction. (iv) $C_{2} H_{4}(g)+H_{2}(g) \rightleftharpoons C_{2} H_{6}(g)$ $\Delta n=1-2=-1 .$ since there is decrease in number of moles on product side. Increase in pressure will favour forward reaction, i.e., the reaction will go towards right direction.
Q. Does the number of moles of reaction products increase, decrease or remain same when each of the following equilibria is subjected to a decrease in pressure by increasing the volume ? (i) $P C l_{5}(g) \rightleftharpoons P C l_{3}(g)+C l_{2}(g)$ (ii) $\mathrm{CaO}(\mathrm{s})+\mathrm{CO}_{2}(\mathrm{g}) \rightleftharpoons \mathrm{CaCO}_{3}(\mathrm{s})$ (iii) 3 Fe $(s)+4 H_{2} O(g) \rightleftharpoons F e_{3} O_{4}(s)+4 H_{2}(g)$
Ans. Applying LeChatelier’s principle, on decreasing the pressure, moles of reaction products will, (i) Increase (ii) Decrease (iii) Remain same $\left(\because n_{p}=n_{r} \text { gaseous }\right)$
Q. In reaction, $\mathrm{CO}(g)+2 \mathrm{H}_{2}(g) \rightleftharpoons \mathrm{CH}_{3} \mathrm{OH}(g) ; \Delta_{r} H^{\circ}=-92.0 \mathrm{kJ} \mathrm{mol}^{-1}$ Concentration of hydrogen, carbon monoxide and methanol become constant at equilibrium. What will happen if: (i) volume of the reaction vessel in which reactants and products are contained is suddenly reduced by half? (ii) the partial pressure of hydrogen is suddenly doubled? (iii) an inert gas is added to the system?
Ans. $C O(g)+2 H_{2}(g) \rightleftharpoons C H_{3} O H(g)$ (i) The equilibrium will shift towards reactants because pressure will become double when volume is reduced to half. High pressure will favour forward reaction because decrease in number of moles are taking place on product side. (ii) When partial pressure of hydrogen is doubled, the equilibrium will shift in forward direction. (iii) When inert gas is added to the system, the equilibrium will shift in backward direction.
Q. The solubility of iodine in water is $1.1 \times 10^{-3} \mathrm{mol} L^{-1}$ at $288 K .$ When $0.200 \mathrm{g}$ of iodine is stirred in $100 \mathrm{g}$ of water till equilibrium is reached, what will be the mass of iodine found in solution and the mass that is left undissolve. After equilibrium is reached with $0.200 \mathrm{g}$ of iodine and 100 of water, we add $150 g$ of water to the system. How much iodine will be dissolved and how much will be left undissolved and what will be the concentration of iodine in solution?
Ans. Solubility of iodine $=1.1 \times 10^{-3} \mathrm{mol} L^{-1}$ This means that $1.1 \times 10^{-3} \mathrm{mol}$ of iodine is present in $1 \mathrm{L}$ of water. Molar mass of iodine $=254$ $\therefore$ Amount of iodine dissolved per litre of water $=1.1 \times 10^{-3} \times 254=0.279 g$ The amount of iodine dissolved per $100 g$ of water $=1.1 \times 10^{-3} \times 254=0.279 g$ The amount of iodine dissolved per $100 \mathrm{g}$ of water $=\frac{0.279}{1000} \times 100=0.0279 g$ Amount of iodine added $=0.200 \mathrm{g}$ Amount of undissolved iodine in of water $=0.2-0.0279=0.172 \mathrm{g}$ When $150 \mathrm{cm}^{3}$ of water is added after the equilibrium, the total volume becomes $100+150=250 \mathrm{cm}^{3}$ Now iodine dissolved in $250 \mathrm{g}$ of water $=\frac{0.279 \times 250}{1000}=$ $0.07 \mathrm{g}$ Amount of undissolved iodine $=0.2-0.07=0.130 \mathrm{g}$ Molar concentration of iodine $=\frac{0.070(\mathrm{g})}{254 \mathrm{g} \mathrm{mol}^{-1} \times 250}\left(\frac{1000}{1 \mathrm{L}}\right)=0.0011 \mathrm{mol} \mathrm{L}^{-1}$
Q. (i) Derive the relationship between $K_{p}$ and $K_{c} .$ (ii) The $K_{p}$ for the reaction $N_{2} O_{4} \rightleftharpoons 2 N O_{2}$ is 640 $m m$ at $775 K .$ Calculate the $\%$ dissociation of $N_{2} O_{4}$ at equilibrium pressure of $160 \mathrm{mm} .$ At which pressure the dissociation will be $50 \%$
Q. $13.8 \mathrm{g}$ of $\mathrm{N}_{2} \mathrm{O}$ was placed in a $1 \mathrm{L}$ reaction vessel at 400 $K$ and allowed to attain equilibrium : $N_{2} O_{4}(g) \rightleftharpoons$ $2 \mathrm{NO}_{2}(\mathrm{g}) .$ The total pressure at equilibrium was found to 9.15 bar. Calculate $K_{c}, K_{p}$ and partial pressure at equilibrium.
Ans. 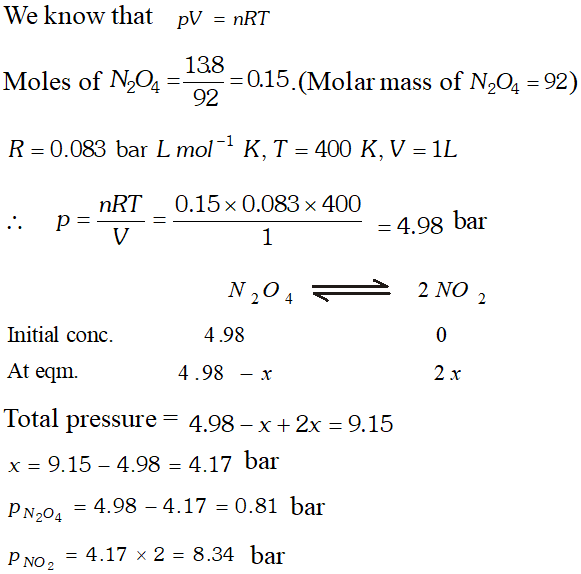
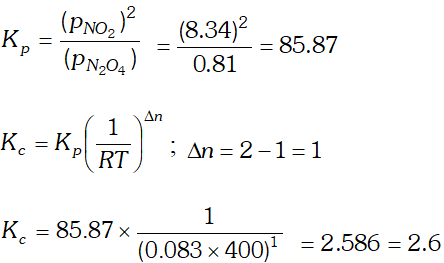
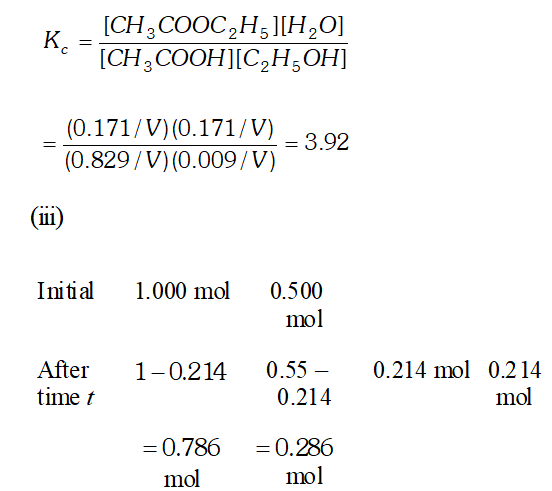
Q. Ethyl acetate is formed by the reaction between ethanol and acetic acid and the equilibrium is represented as: $\left.\mathrm{CH}_{3} \mathrm{COOH} / \mathrm{l}\right)+\mathrm{C}_{2} \mathrm{H}_{5} \mathrm{OH}(\mathrm{l}) \rightleftharpoons \mathrm{CH}_{3} \mathrm{COO}_{4} \mathrm{f}_{5}(\mathrm{l})+\mathrm{H}_{2} \mathrm{O}(\mathrm{l})$ (i) Write the concentration ratio (reaction quotient), $Q_{c},$ for this reaction (Note: water is not in excess and is not a solvent in this reaction). (ii) At $293 K,$ if one starts with 1.00 mol of acetic acid and 0.18 mol of ethanol, there is $0.171 \mathrm{mol}$ of ethyl acetate in the final equilibrium mixture. Calculate the equilibrium constant. (iii) Starting with 0.5 mol of ethanol and 1.0 mol of acetic acid and maintaining it at $293 \mathrm{K}, 0.214 \mathrm{mol}$ of ethyl acetate is found after some time. Has equilibrium been reached ?
Q. At $473 K,$ the equilibrium constant $K_{c}$ for decomposition of Phosphorus Pentachloride is $8.3 \times 10^{-3} .$ If decomposition is represented by: $P C l_{5}(g) \rightleftharpoons P C l_{3}(g)+C l_{2}(g)$ $\Delta H_{f}^{\circ}=+124.8 k_{\mathrm{d}} \mathrm{mol}^{-1}$ (i) Write expression $K_{c}$ for the reaction. (ii) What would be the effect on $K_{c}$ if : (a) more $P C l_{5}$ is added (b) pressure is increased (c) temperature is increased (e) catalyst is added. (iii) What is the value of Kc for the reverse reaction at the same temperature
Ans. 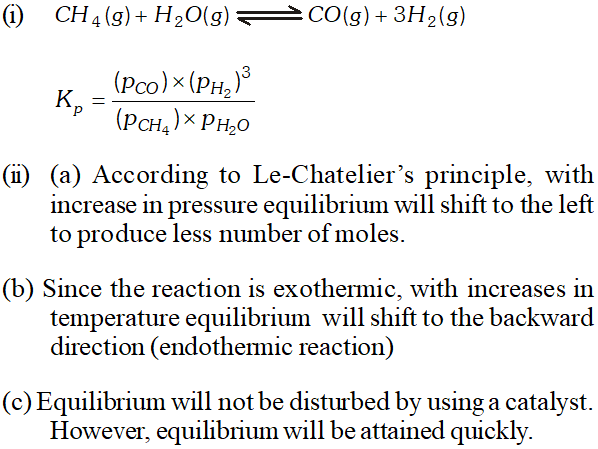
Q. Which of the following reactions will get affected by increasing the pressure? Also mention whether change will cause the reaction to go into forward or backward direction? (i) $\mathrm{COCl}_{2}(g) \rightleftharpoons \mathrm{CO}(g)+\mathrm{Cl}_{2}(g)$ (ii) $C H_{4}(g)+2 S_{2}(g) \rightleftharpoons C S_{2}(g)+2 H_{2} S(g)$ (iii) $C O_{2}(g)+C(s) \rightleftharpoons 2 C O(g)$ (iv) $2 H_{2}(g)+C O(g) \rightleftharpoons C H_{3} O H(g)$ (v) $\mathrm{CaCO}_{3}(\mathrm{s}) \rightleftharpoons \mathrm{CaO}(\mathrm{s})+\mathrm{CO}_{2}(\mathrm{g})$ (vi) $4 N H_{3}(g)+5 O_{2}(g) \rightleftharpoons 4 N O(g)+6 H_{2} O(g)$
Ans. $P C l_{5}(g) \rightleftharpoons P C l_{3}(g)+C l_{2}(g) ; \Delta_{f} H^{\circ}=+124.8 k J m o l^{-1}$ (i) $\quad K_{c}=\frac{\left[P C l_{3}\right]\left[C l_{2}\right]}{\left[P C l_{5}\right]}$ (ii) $(a) K_{c}$ will not be affected if more $P C l_{5}$ is added. (b) does not change with increase in pressure. (c) will increase, when temperature is increased. (d) is not affected by catalyst.
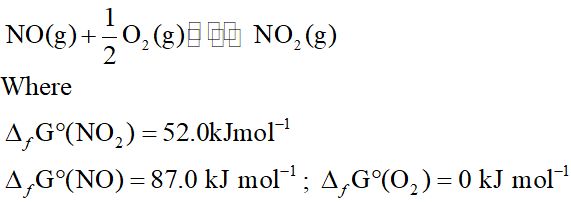
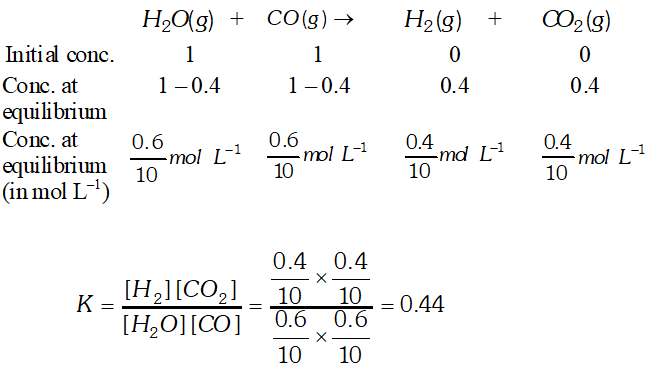
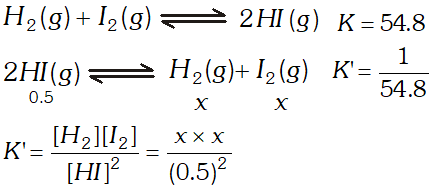 $\frac{1}{54.8}=\frac{x^{2}}{(0.5)^{2}} \Rightarrow x^{2}=\frac{0.5 \times 0.5}{54.8} \Rightarrow x=0.0675 \mathrm{mol} L^{-1}$
$\frac{1}{54.8}=\frac{x^{2}}{(0.5)^{2}} \Rightarrow x^{2}=\frac{0.5 \times 0.5}{54.8} \Rightarrow x=0.0675 \mathrm{mol} L^{-1}$ 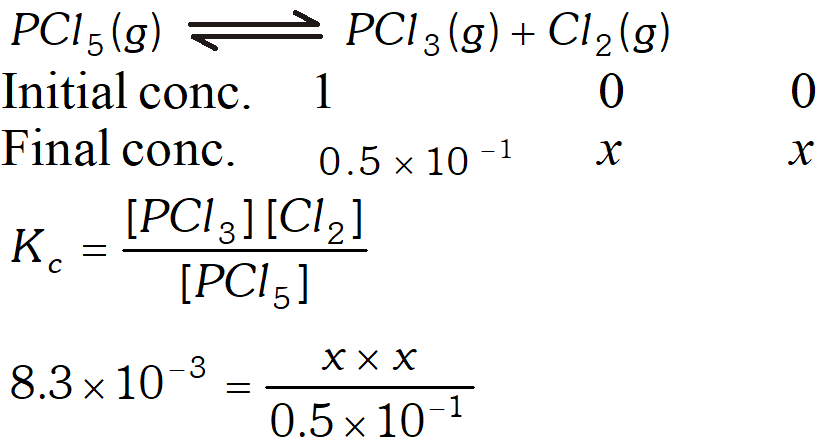
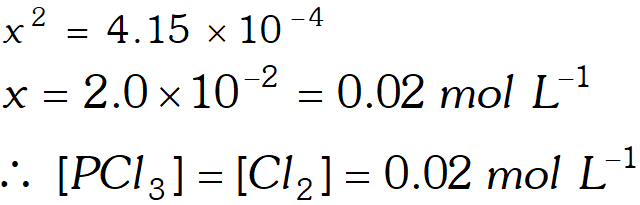

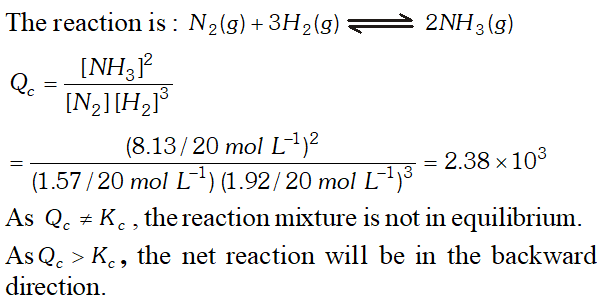
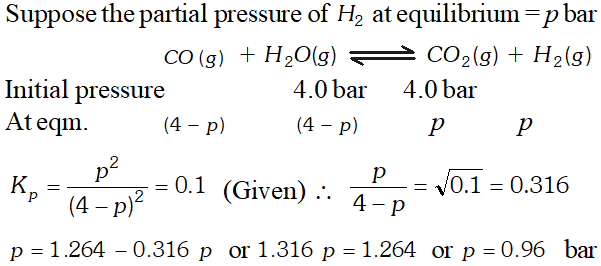


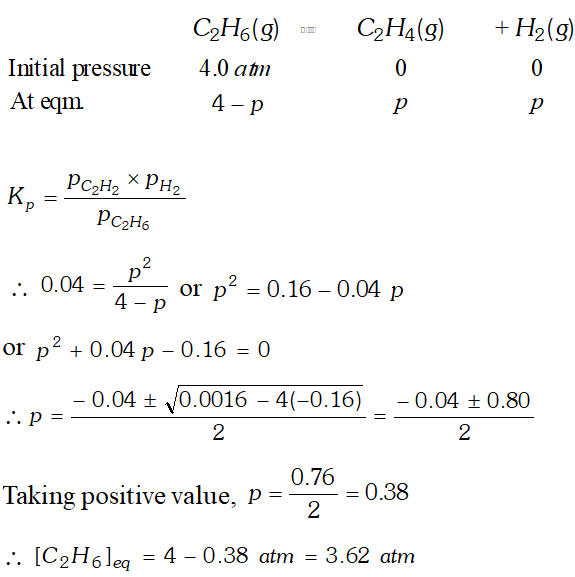

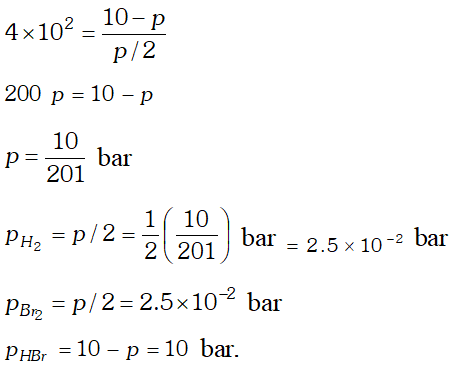
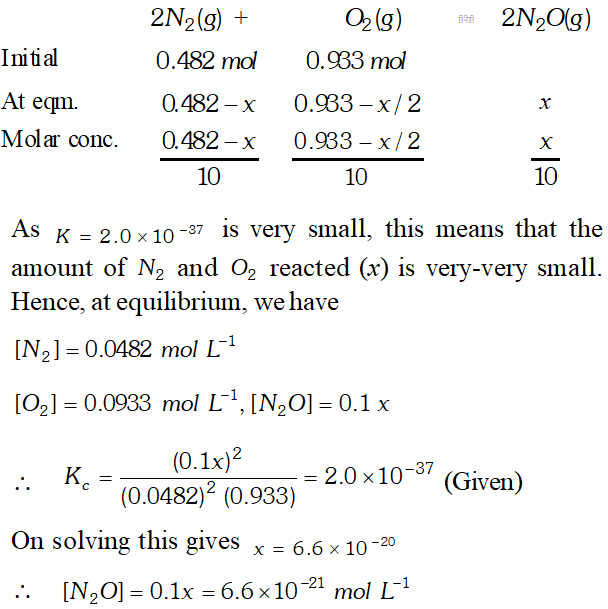

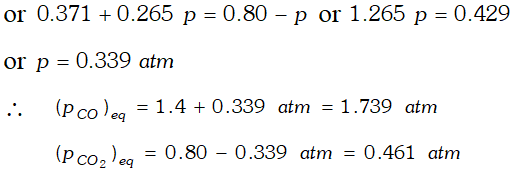
 since $Q_{c}$ is not equal to $K_{c},$ the reaction is not at equilibrium. since is less than, the reaction would tend to proceed in forward direction to attain equilibrium
since $Q_{c}$ is not equal to $K_{c},$ the reaction is not at equilibrium. since is less than, the reaction would tend to proceed in forward direction to attain equilibrium 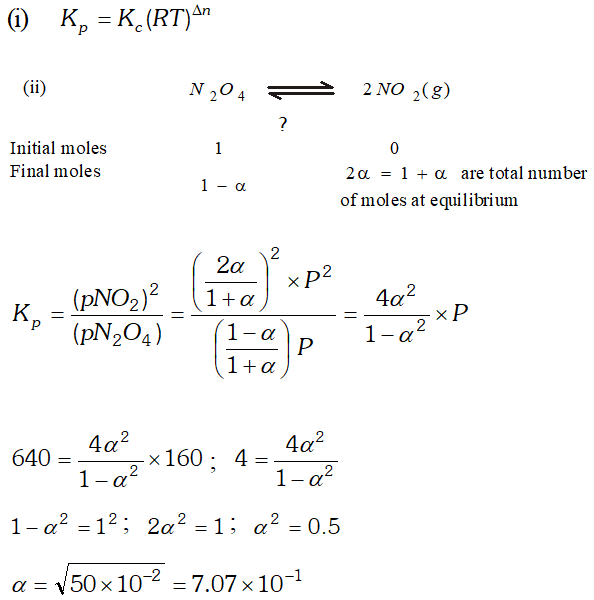
![]()